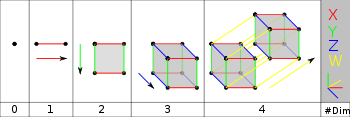
Hypercube

This article is about the mathematical concept. For the film, see Cube 2: Hypercube.

Perspective projections

Cube (3-cube)	Tesseract (4-cube)

In geometry, a hypercube is an n-dimensional analogue of a square (n = 2) and a cube (n = 3). It is a closed, compact, convex figure whose 1-skeleton consists of groups of opposite parallel line segments aligned in each of the space's dimensions, perpendicular to each other and of the same length.

An n-dimensional hypercube is also called an n-cube. The term "measure polytope" is also used, notably in the work of H.S.M. Coxeter (originally from Elte, 1912^[1]), but it has now been superseded.

The hypercube is the special case of a hyperrectangle (also called an orthotope).

A unit hypercube is a hypercube whose side has length one unit. Often, the hypercube whose corners (or vertices) are the 2ⁿ points in Rⁿ with coordinates equal to 0 or 1 is called "the" unit hypercube.

Construction

A diagram showing how to create a tesseract from a point.

0 – A point is a hypercube of dimension zero.

1 – If one moves this point one unit length, it will sweep out a line segment, which is a unit hypercube of dimension one.

2 – If one moves this line segment its length in a perpendicular direction from itself; it sweeps out a 2-dimensional square.

3 – If one moves the square one unit length in the direction perpendicular to the plane it lies on, it will generate a 3-dimensional cube.

4 – If one moves the cube one unit length into the fourth dimension, it generates a 4-dimensional unit hypercube (a unit tesseract).

This can be generalized to any number of dimensions. This process of sweeping out volumes can be formalized mathematically as a Minkowski sum: the d-dimensional hypercube is the Minkowski sum of d mutually perpendicular unit-length line segments, and is therefore an example of a zonotope.

The 1-skeleton of a hypercube is a hypercube graph.

Coordinates

A unit hypercube of n dimensions is the convex hull of the points given by all sign permutations of the Cartesian coordinates $(\pm 1/2, \pm 1/2, \cdots, \pm 1/2)$. It has an edge length of 1 and an n-dimensional volume of 1.

An n-dimensional hypercube is also often regarded as the convex hull of all sign permutations of the coordinates $(\pm 1, \pm 1, \cdots, \pm 1)$. This form is often chosen due to ease of writing out the coordinates. Its edge length is 2, and its n-dimensional volume is 2ⁿ.

Related families of polytopes

The hypercubes are one of the few families of regular polytopes that are represented in any number of dimensions.

The hypercube (offset) family is one of three regular polytope families, labeled by Coxeter as γ_n. The other two are the hypercube dual family, the cross-polytopes, labeled as β_n, and the simplices, labeled as α_n. A fourth family, the infinite tessellations of hypercubes, he labeled as δ_n.

Another related family of semiregular and uniform polytopes is the demihypercubes, which are constructed from hypercubes with alternate vertices deleted and simplex facets added in the gaps, labeled as hγ_n.

Elements

A hypercube of dimension n has 2n "sides" (a 1-dimensional line has 2 end points; a 2-dimensional square has 4 sides or edges; a 3-dimensional cube has 6 2-dimensional faces; a 4-dimensional tesseract has 8 cells). The number of vertices (points) of a hypercube is 2ⁿ (a cube has 2³ vertices, for instance).

A simple formula to calculate the number of "n-2"-faces in an n-dimensional hypercube is: 2n² − 2n

The number of m-dimensional hypercubes (just referred to as m-cube from here on) on the boundary of an n-cube is

$E_{m,n} = 2^{n-m}{n \choose m}$,     where ${n \choose m}=\frac{n!}{m!\,(n-m)!}$ and n! denotes the factorial of n.

For example, the boundary of a 4-cube (n=4) contains 8 cubes (3-cubes), 24 squares (2-cubes), 32 lines (1-cubes) and 16 vertices (0-cubes).

This identity can be proved by combinatorial arguments; each of the 2ⁿ vertices defines a vertex in a m-dimensional boundary. There are ${n \choose m}$ ways of choosing which lines ("sides") that defines the subspace that the boundary is in. But, each side is counted 2^m times since it has that many vertices, we need to divide with this number. Hence the identity above.

These numbers can also be generated by the linear recurrence relation

$E_{m,n} = 2E_{m,n-1} + E_{m-1,n-1} \!$,     with $E_{0,0} = 1 \!$,     and undefined elements = 0.

For example, extending a square via its 4 vertices adds one extra line (edge) per vertex, and also adds the final second square, to form a cube, giving $E_{1,3} \!$ = 12 lines in total.

Hypercube elements $E_{m,n} \!$

			m	0	1	2	3	4	5	6	7	8	9	10
n	γn	n-cube	Names Schläfli symbol Coxeter-Dynkin	Vertices	Edges	Faces	Cells (3-faces)	4-faces (Hypercells)	5-faces	6-faces	7-faces	8-faces	9-faces	10-faces
0	γ0	0-cube	Point -	1
1	γ1	1-cube	Line segment {}	2	1
2	γ2	2-cube	Square Tetragon {4}	4	4	1
3	γ3	3-cube	Cube Hexahedron {4,3}	8	12	6	1
4	γ4	4-cube	Tesseract Octachoron {4,3,3}	16	32	24	8	1
5	γ5	5-cube	Penteract Decateron {4,3,3,3}	32	80	80	40	10	1
6	γ6	6-cube	Hexeract Dodecapeton {4,3,3,3,3}	64	192	240	160	60	12	1
7	γ7	7-cube	Hepteract Tetradeca-7-tope {4,3,3,3,3,3}	128	448	672	560	280	84	14	1
8	γ8	8-cube	Octeract Hexadeca-8-tope {4,3,3,3,3,3,3}	256	1024	1792	1792	1120	448	112	16	1
9	γ9	9-cube	Enneract Octadeca-9-tope {4,3,3,3,3,3,3,3}	512	2304	4608	5376	4032	2016	672	144	18	1
10	γ10	10-cube	Dekeract icosa-10-tope {4,3,3,3,3,3,3,3,3}	1024	5120	11520	15360	13440	8064	3360	960	180	20	1

Graphs

An n-cube can be projected inside a regular 2n-gonal polygon by a skew orthogonal projection, shown here from the line segment to the dodekeract.

Petrie polygon Orthographic projections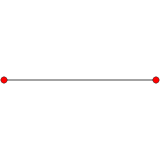

Line segment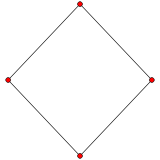	Square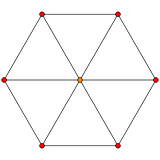	Cube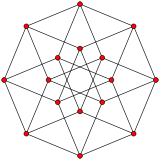	4-cube (tesseract)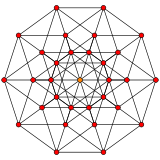
5-cube (penteract)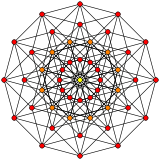	6-cube (hexeract)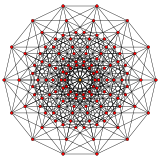	7-cube (hepteract)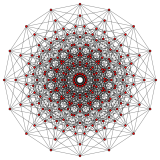	8-cube (octeract)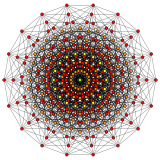
9-cube (enneract)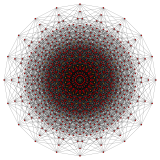	10-cube (dekeract)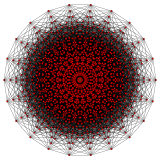	11-cube (hendekeract)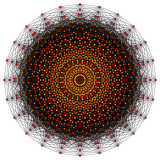	12-cube (dodekeract)

Projection of a rotating hypercube (tesseract to be exact).

Relation to n-simplices

The graph of the n-hypercube's edges is isomorphic to the Hasse diagram of the (n-1)-simplex's face lattice. This can be seen by orienting the n-hypercube so that two opposite vertices lie vertically, corresponding to the (n-1)-simplex itself and the null polytope, respectively. Each vertex connected to the top vertex then uniquely maps to one of the (n-1)-simplex's facets (n-2 faces), and each vertex connected to those vertices maps to one of the simplex's n-3 faces, and so forth, and the vertices connected to the bottom vertex map to the simplex's vertices.

This relation may be used to generate the face lattice of an (n-1)-simplex efficiently, since face lattice enumeration algorithms applicable to general polytopes are more computationally expensive.

See also

• Hyperoctahedral group, the symmetry group of the hypercube
• Hypersphere
• Simplex

• Hypercube interconnection network of computer architecture

Notes

1. ^ Elte, E. L. (1912). The Semiregular Polytopes of the Hyperspaces. Groningen: University of Groningen  Chapter IV, five dimensional semiregular polytope [1]

References

• Bowen, J. P. (April 1982). "Hypercubes". Practical Computing 5 (4): 97–99. http://www.jpbowen.com/publications/ndcubes.html. 
• Coxeter, H. S. M. (1973). Regular Polytopes (3rd ed.). Dover. pp. 123. ISBN 0-486-61480-8.  p. 296, Table I (iii): Regular Polytopes, three regular polytopes in n dimensions (n ≥ 5)
• Hill, Frederick J.; Gerald R. Peterson. Introduction to Switching Theory and Logical Design: Second Edition. NY: John Wiley & Sons. ISBN 0-471-39882-9.  Cf Chapter 7.1 "Cubical Representation of Boolean Functions" wherein the notion of "hypercube" is introduced as a means of demonstrating a distance-1 code (Gray code) as the vertices of a hypercube, and then the hypercube with its vertices so labelled is squashed into two dimensions to form either a Veitch diagram or Karnaugh map.

External links

• Weisstein, Eric W., "Hypercube" from MathWorld.
• Weisstein, Eric W., "Hypercube graphs" from MathWorld.
• Olshevsky, George, Measure polytope at Glossary for Hyperspace.
• www.4d-screen.de (Rotation of 4D – 7D-Cube)
• Rotating a Hypercube by Enrique Zeleny, Wolfram Demonstrations Project.
• Stereoscopic Animated Hypercube