- 5-demicube
-
Demipenteract
(5-demicube)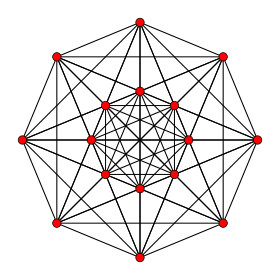
Petrie polygon projectionType Uniform 5-polytope Family (Dn) 5-demicube Families (En) k21 polytope
1k2 polytopeCoxeter symbol 121 Schläfli symbol {31,2,1}
h{4,3,3,3}
s{2,2,2,2}Coxeter-Dynkin diagram 
























4-faces 26 10 {31,1,1} 
16 {3,3,3}
Cells 120 40 {31,0,1} 
80 {3,3}
Faces 160 {3} 
Edges 80 Vertices 16 Vertex figure 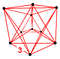
rectified 5-cellPetrie polygon Octagon Symmetry group D5, [34,1,1] = [1+,4,33]
[24]+Properties convex In five dimensional geometry, a demipenteract or 5-demicube is a semiregular 5-polytope, constructed from a 5-hypercube (penteract) with alternated vertices deleted.
It was discovered by Thorold Gosset. Since it was the only semiregular 5-polytope (made of more than one type of regular hypercell), he called it a 5-ic semi-regular.
Coxeter named this polytope as 121 from its Coxeter-Dynkin diagram, which has branches of length 2, 1 and 1 with a ringed node on one of the short branches. It exists in the k21 polytope family as 121 with the Gosset polytopes: 221, 321, and 421.
Contents
Cartesian coordinates
Cartesian coordinates for the vertices of a demipenteract centered at the origin and edge length 2√2 are alternate halves of the penteract:
- (±1,±1,±1,±1,±1)
with an odd number of plus signs.
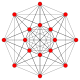
Projected images

Perspective projection.Images
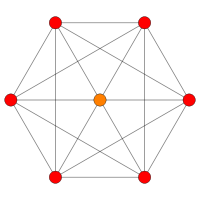
orthographic projections Coxeter plane B5 Graph 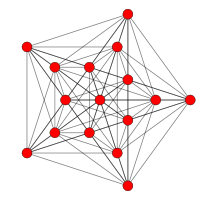
Dihedral symmetry [10/2] Coxeter plane D5 D4 Graph 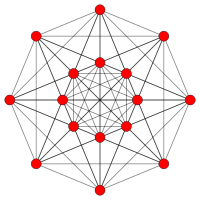
Dihedral symmetry [8] [6] Coxeter plane D3 A3 Graph 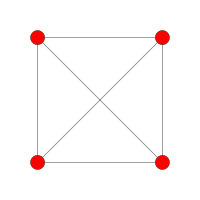
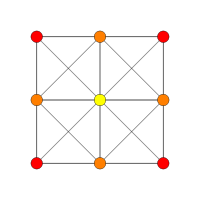
Dihedral symmetry [4] [4] Related polytopes
It is a part of a dimensional family of uniform polytopes called demihypercubes for being alternation of the hypercube family.
There are 23 uniform polytera (uniform 5-polytope) that can be constructed from the D5 symmetry of the demipenteract, 8 of which are unique to this family, and 15 are shared within the penteractic family.
t0(121)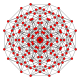
t0,1(121)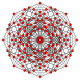
t0,2(121)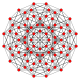
t0,3(121)
t0,1,2(121)
t0,1,3(121)
t0,2,3(121)
t0,1,2,3(121)References
- T. Gosset: On the Regular and Semi-Regular Figures in Space of n Dimensions, Messenger of Mathematics, Macmillan, 1900
- H.S.M. Coxeter:
- Coxeter, Regular Polytopes, (3rd edition, 1973), Dover edition, ISBN 0-486-61480-8, p.296, Table I (iii): Regular Polytopes, three regular polytopes in n-dimensions (n≥5)
- H.S.M. Coxeter, Regular Polytopes, 3rd Edition, Dover New York, 1973, p.296, Table I (iii): Regular Polytopes, three regular polytopes in n-dimensions (n≥5)
- Kaleidoscopes: Selected Writings of H.S.M. Coxeter, editied by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6 [1]
- (Paper 22) H.S.M. Coxeter, Regular and Semi Regular Polytopes I, [Math. Zeit. 46 (1940) 380-407, MR 2,10]
- (Paper 23) H.S.M. Coxeter, Regular and Semi-Regular Polytopes II, [Math. Zeit. 188 (1985) 559-591]
- (Paper 24) H.S.M. Coxeter, Regular and Semi-Regular Polytopes III, [Math. Zeit. 200 (1988) 3-45]
- John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, The Symmetries of Things 2008, ISBN 978-1-56881-220-5 (Chapter 26. pp. 409: Hemicubes: 1n1)
- Richard Klitzing, 5D uniform polytopes (polytera), x3o3o *b3o3o - hin
External links
- Olshevsky, George, Demipenteract at Glossary for Hyperspace.
- Multi-dimensional Glossary
Fundamental convex regular and uniform polytopes in dimensions 2–10 Family An BCn Dn E6 / E7 / E8 / F4 / G2 Hn Regular polygon Triangle Square Hexagon Pentagon Uniform polyhedron Tetrahedron Octahedron • Cube Demicube Dodecahedron • Icosahedron Uniform polychoron 5-cell 16-cell • Tesseract Demitesseract 24-cell 120-cell • 600-cell Uniform 5-polytope 5-simplex 5-orthoplex • 5-cube 5-demicube Uniform 6-polytope 6-simplex 6-orthoplex • 6-cube 6-demicube 122 • 221 Uniform 7-polytope 7-simplex 7-orthoplex • 7-cube 7-demicube 132 • 231 • 321 Uniform 8-polytope 8-simplex 8-orthoplex • 8-cube 8-demicube 142 • 241 • 421 Uniform 9-polytope 9-simplex 9-orthoplex • 9-cube 9-demicube Uniform 10-polytope 10-simplex 10-orthoplex • 10-cube 10-demicube n-polytopes n-simplex n-orthoplex • n-cube n-demicube 1k2 • 2k1 • k21 pentagonal polytope Topics: Polytope families • Regular polytope • List of regular polytopes Categories:- 5-polytopes
Wikimedia Foundation. 2010.
