Conservative force

Classical mechanics
$\mathbf{F} = m \mathbf{a}$ Newton's Second Law
History of classical mechanics · Timeline of classical mechanics
Branches Statics · Dynamics / Kinetics · Kinematics · Applied mechanics · Celestial mechanics · Continuum mechanics · Statistical mechanics
Formulations Newtonian mechanics (Vectorial mechanics) Analytical mechanics: Lagrangian mechanics Hamiltonian mechanics
Fundamental concepts Space · Time · Velocity · Speed · Mass · Acceleration · Gravity · Force · Impulse · Torque / Moment / Couple · Momentum · Angular momentum · Inertia · Moment of inertia · Reference frame · Energy · Kinetic energy · Potential energy · Mechanical work · Virtual work · D'Alembert's principle
Core topics Rigid body · Rigid body dynamics · Euler's equations (rigid body dynamics) · Motion · Newton's laws of motion · Newton's law of universal gravitation · Euler's laws of motion · Equations of motion · Inertial frame of reference · Non-inertial reference frame · Rotating reference frame · Fictitious force · Linear motion · Mechanics of planar particle motion · Displacement (vector) · Relative velocity · Friction · Simple harmonic motion · Harmonic oscillator · Vibration · Damping · Damping ratio · Rotational motion · Circular motion · Uniform circular motion · Non-uniform circular motion · Centripetal force · Centrifugal force · Centrifugal force (rotating reference frame) · Reactive centrifugal force · Coriolis force · Pendulum · Rotational speed · Angular acceleration · Angular velocity · Angular frequency · Angular displacement
Scientists Galileo Galilei · Isaac Newton · Jeremiah Horrocks · Leonhard Euler · Jean le Rond d'Alembert · Alexis Clairaut · Joseph Louis Lagrange · Pierre-Simon Laplace · William Rowan Hamilton · Siméon-Denis Poisson
v · d · e

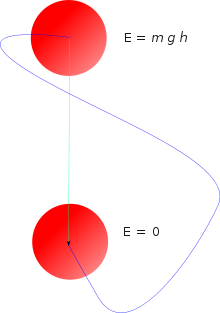
A conservative force is a force with the property that the work done in moving a particle between two points is independent of the path taken.^[1] Equivalently, if a particle travels in a closed loop, the net work done (the sum of the force acting along the path multiplied by the distance travelled) by a conservative force is zero.^[2]

It is possible to define a numerical value of potential at every point in space for a conservative force. When an object moves from one location to another, the force changes the potential energy of the object by an amount that does not depend on the path taken.

Gravity is an example of a conservative force, while friction is an example of a non-conservative force.

Informal definition

Informally, a conservative force can be thought of as a force that conserves mechanical energy. Suppose a particle starts at point A, and there is a constant force F acting on it. Then the particle is moved around by other forces, and eventually ends up at A again. Though the particle may still be moving, at that instant when it passes point A again, it has traveled a closed path. If the net work done by F at this point is 0, then F passes the closed path test. Any force that passes the closed path test for all possible closed paths is classified as a conservative force.

The gravitational force, spring force, magnetic force (according to some definitions, see below) and electric force (at least in a time-independent magnetic field, see Faraday's law of induction for details) are examples of conservative forces, while friction and air drag are classical examples of non-conservative forces.

For non-conservative forces, the mechanical energy that is lost (not conserved) has to go somewhere else, by conservation of energy. Usually the energy is turned into heat, for example the heat generated by friction. In addition to heat, friction also often produces some sound energy. The water drag on a moving boat converts the boat's mechanical energy into not only heat and sound energy, but also wave energy at the edges of its wake. These and other energy losses are irreversible because of the second law of thermodynamics.

Path independence

The work done by the gravitational force on an object depends only on its change in height because the gravitational force is conservative.

A direct consequence of the closed path test is that the work done by a conservative force on a particle moving between any two points does not depend on the path taken by the particle. Also the work done by a conservative force is equal to the negative of change in potential energy during that process. For a proof of that, let's imagine two paths 1 and 2, both going from point A to point B. The variation of energy for the particle, taking path 1 from A to B and then path 2 backwards from B to A, is 0; thus, the work is the same in path 1 and 2, i.e., the work is independent of the path followed, as long as it goes from A to B.

For example, if a child slides down a frictionless slide, the work done by the gravitational force on the child from the top of the slide to the bottom will be the same no matter what the shape of the slide; it can be straight or it can be a spiral. The amount of work done only depends on the vertical displacement of the child.

Mathematical description

A force field F, defined everywhere in space (or within a simply-connected volume of space), is called a conservative force or conservative vector field if it meets any of these three equivalent conditions:

1. The curl of F is zero:

$\nabla \times \vec{F} = 0. \,$

2. There is zero net work (W) done by the force when moving a particle through a trajectory that starts and ends in the same place:

$W \equiv \oint_C \vec{F} \cdot \mathrm{d}\vec r = 0.\,$

3. The force can be written as the gradient of a potential, Φ:

$\vec{F} = -\nabla \Phi. \,$

Proof that these three conditions are equivalent when F is a force field

Main article: Conservative vector field 1 implies 2: Let C be any simple closed path (i.e., a path that starts and ends at the same point and has no self-intersections), and consider a surface S of which C is the boundary. Then Stokes' theorem says that $\int_S (\nabla \times \vec{F}) \cdot \mathrm{d}\vec{a} = \oint_C \vec{F} \cdot \mathrm{d}\vec{r}$ If the curl of F is zero the left hand side is zero - therefore statement 2 is true. 2 implies 3: Assume that statement 2 holds. Let c be a simple curve from the origin to a point x and define a function $\Phi(x) = -\int_c \vec{F} \cdot \mathrm{d}\vec{r}.$ The fact that this function is well-defined (independent of the choice of c) follows from statement 2. Anyway, from the fundamental theorem of calculus, it follows that $\vec{F} = -\nabla \Phi.$ So statement 2 implies statement 3. 3 implies 1: Finally, assume that the third statement is true. A well-known vector calculus identity states that the curl of the gradient of any function is 0. (See proof.) Therefore, if the third statement is true, then the first statement must be true as well. This shows that statement 1 implies 2, 2 implies 3, and 3 implies 1. Therefore all three are equivalent, Q.E.D.. (The equivalence of 1 and 3 is also known as (one aspect of) Helmholtz's theorem.)

The term conservative force comes from the fact that when a conservative force exists, it conserves mechanical energy. The most familiar conservative forces are gravity, the electric force (in a time-independent magnetic field, see Faraday's law), and spring force.

Many forces (particularly those that depend on velocity) are not force fields. In these cases, the above three conditions are not mathematically equivalent. For example, the magnetic force satisfies condition 2 (since the work done by a magnetic field on a charged particle is always zero), but does not satisfy condition 3, and condition 1 is not even defined (the force is not a vector field, so one cannot evaluate its curl). Accordingly, some authors classify the magnetic force as conservative,^[3] while others do not.^[4] The magnetic force is an unusual case; most velocity-dependent forces, such as friction, do not satisfy any of the three conditions, and therefore are unambiguously nonconservative.

Nonconservative forces

Nonconservative forces can only arise in classical physics due to neglected degrees of freedom. For instance, friction may be treated without resorting to the use of nonconservative forces by considering the motion of individual molecules; however that means every molecule's motion must be considered rather than handling it through statistical methods. For macroscopic systems the nonconservative approximation is far easier to deal with than millions of degrees of freedom. Examples of nonconservative forces are friction and non-elastic material stress.

However, general relativity is non-conservative, as seen in the anomalous precession of Mercury's orbit. However, general relativity can be shown to conserve a stress-energy-momentum pseudotensor.

References

1. ^ HyperPhysics - Conservative force
2. ^ Louis N. Hand, Janet D. Finch (1998). Analytical Mechanics. Cambridge University Press. p. 41. ISBN 0521575729. 
3. ^ For example, Mechanics, P.K. Srivastava, 2004, page 94: "In general, a force which depends explicitly upon the velocity of the particle is not conservative. (However, the magnetic force (qv×B) can be included among conservative forces in the sense that it acts perpendicular to velocity and hence work done is always zero". Web link
4. ^ For example, The Magnetic Universe: Geophysical and Astrophysical Dynamo Theory, Rüdiger and Hollerbach, page 178, Web link