Deferent and epicycle

"Deferent" redirects here. For the acknowledgement of the legitimacy of the power of one's superior or superiors, see Deference.

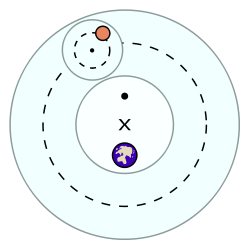The basic elements of Ptolemaic astronomy, showing a planet on an epicycle (smaller dashed circle) , a deferent (larger dashed circle), and an equant (larger black dot).

In the Ptolemaic system of astronomy, the epicycle (literally: on the circle in Greek) was a geometric model used to explain the variations in speed and direction of the apparent motion of the Moon, Sun, and planets. It was first proposed by Apollonius of Perga at the end of the 3rd century BC and formalized by Ptolemy of the Thebaid in his 2nd-century AD astronomical treatise the Almagest. In particular it explained the retrograde motion of the five planets known at the time. Secondarily, it also explained changes in the apparent distances of the planets from Earth.

It is called Ptolemaic after the Greek astronomer Ptolemy, although it had been developed by previous Greek astronomers such as Apollonius of Perga and Hipparchus of Rhodes, who used it extensively, almost five centuries before Ptolemy. Epicyclical motion is used in the Antikythera Mechanism, an ancient Greek astronomical device for computing the phase and position of the Moon using four gears, two of them engaged in an eccentric way that closely approximates Kepler's second law, i.e. the Moon moves faster at perigee and slower at apogee.

In the Ptolemaic system, the planets are assumed to move in a small circle called an epicycle, which in turn moves along a larger circle called a deferent. Both circles rotate eastward and are roughly parallel to the plane of the Sun's orbit (ecliptic). The orbits of planets in this system are epitrochoids.

Despite the fact that the Ptolemaic system is considered geocentric, the planets' motion was not thought to be actually centered on the Earth. Instead, the deferent was centered on a point halfway between the Earth and another point called the equant. The epicycle, meanwhile, rotated and revolved along the deferent with uniform motion. The rate at which the planet moved on the epicycle was fixed such that the angle between the center of the epicycle and the planet was the same as the angle between the earth and the sun.

Ptolemy did not predict the relative sizes of the planetary deferents in the Almagest. All of his calculations were done with respect to a normalized deferent. This is not to say that he believed the planets were all equidistant. He did guess at an ordering of the planets. Later he calculated their distances in the Planetary Hypotheses.

For superior planets the planet would typically move through in the night sky slower than the stars. Each night the planet would "lag" a little behind the star. This is prograde motion. Occasionally, near opposition, the planet would appear to move through in the night sky faster than the stars. This is retrograde motion. Ptolemy's model, in part, sought to explain this behavior.

The inferior planets were always observed to be near the sun, appearing only shortly before sunrise or shortly after sunset. To accommodate this, Ptolemy's model fixed the motion of Mercury and Venus so that the line from the equant point to the center of the epicycle was always parallel to the earth-sun line.

History

When ancient astronomers viewed the sky, they saw the Sun, Moon, and stars moving overhead in a regular fashion. They also saw the "wanderers" or "planetai" (our planets). The regularity in the motions of the wandering bodies suggested that their positions might be predictable.

The apparent movement of sun and planets with earth as center

The most obvious approach to the problem of predicting the motions of the heavenly bodies was simply to map their positions against the star field and then to fit mathematical functions to the changing positions.^[1]

The ancients worked from a geocentric perspective for the simple reason that the Earth was where they stood and observed the sky, and it is the sky which appears to move while the ground seems still and steady underfoot. Some Greek astronomers (e.g., Aristarchus of Samos) speculated that the planets (Earth included) orbited the Sun, but the optics (and the specific mathematics - Newton's Law of Gravitation for example) necessary to provide data that would convincingly support the heliocentric model did not exist in Ptolemy's time and would not come around for over fifteen hundred years after his death. Furthermore, Aristotelian Physics was not designed with these sorts of calculations in mind, and Aristotle's philosophy regarding the heavens was entirely at odds with the concept of heliocentrism. It was not until Galileo Galilei observed the moons of Jupiter on January 7, 1610, and the phases of Venus in September, 1610 that the heliocentric model began to receive broad support among astronomers, who also came to accept the notion that the planets are individual worlds orbiting the Sun (that is, that Earth is a planet and is one among several.) The effects the telescope had on astronomy came quickly and were both controversial and profound. Johannes Kepler was able to formulate his famous three laws of planetary motion, which described the orbits of the planets in our solar system with incredible accuracy; Kepler's three laws are still taught today in University physics and astronomy classes, and the wording of these laws has not changed since Kepler first formulated them four hundred years ago.

The apparent motion of the heavenly bodies with respect to time is cyclical in nature. Apollonius of Perga realized that this cyclical variation could be represented visually by circles, or epicycles (mini circular orbits), revolving on larger circular orbits, or deferents. Deferents and epicycles in the ancient models did not represent orbits in the modern sense. The ancients did not know about orbits.

Claudius Ptolemy refined the deferent/epicycle concept and introduced the equant as a mechanism for accounting for velocity variations in the motions of the planets. The empirical methodology he developed proved to be extraordinarily accurate for its day and was still in use at the time of Copernicus and Kepler.

Owen Gingerich^[2] describes a planetary conjunction that occurred in 1504 that was apparently observed by Copernicus. In notes bound with his copy of the Alfonsine Tables, Copernicus commented that "Mars surpasses the numbers by more than two degrees. Saturn is surpassed by the numbers by one and a half degrees." Using modern computer programs, Gingerich discovered that, at the time of the conjunction, Saturn indeed lagged behind the tables by a degree and a half and Mars led the predictions by nearly two degrees. Moreover, he found that Ptolemy's predictions for Jupiter at the same time were quite accurate. Copernicus and his contemporaries were therefore using Ptolemy's methods and finding them trustworthy well over a thousand years after Ptolemy's original work was published.

When Copernicus transformed Earth-based observations to heliocentric coordinates,^[3] he was confronted with an entirely new problem. The Sun-centered positions displayed a cyclical motion with respect to time but without retrograde loops in the case of the outer planets. In principle, the heliocentric motion was simpler but with new subtleties due to the yet-to-be-discovered elliptical shape of the orbits. Another complication was caused by a problem that Copernicus never solved: correctly accounting for the motion of the Earth in the coordinate transformation.^[4] In keeping with past practice, Copernicus used the deferent/epicycle model in his theory but his epicycles were small and were called "epicyclets".

In the Ptolemaic system the models for each of the planets were different and so it was with Copernicus' initial models. As he worked through the mathematics, however, Copernicus discovered that his models could be combined in a unified system. Furthermore, if they were scaled so that Earth's orbit was the same in all of them, the ordering of the planets we recognize today easily followed from the math. Mercury orbited closest to the Sun and the rest of the planets fell into place in order outward, arranged in distance by their periods of revolution.^[5]

Although Copernicus' models reduced the magnitude of the epicycles considerably, whether they were simpler than Ptolemy's is moot. Copernicus eliminated Ptolemy's somewhat-maligned equant but at a cost of additional epicycles. Various 16th-century books based on Ptolemy and Copernicus use about equal numbers of epicycles.^[6][7][8] The idea that Copernicus used only 34 circles in his system comes from his own statement in a preliminary unpublished sketch called the Commentariolus. By the time he published De revolutionibus orbium coelestium, he had added more circles. Counting the total number is difficult, but estimates are that he created a system just as complicated, or even more so.^[9] The popular total of about 80 circles for the Ptolemaic system seems to have appeared in 1898. It may have been inspired by the non-Ptolemaic system of Girolamo Fracastoro, who used either 77 or 79 orbs in his system inspired by Eudoxus of Cnidus.^[10]

Copernicus' theory was at least as accurate as Ptolemy's but never achieved the stature and recognition of Ptolemy's theory. What was needed was Kepler's elliptical theory, not published till 1609. Copernicus' work provided explanations for phenomena like retrograde motion, but really didn't prove that the planets actually orbited the Sun.

Ptolemy's and Copernicus' theories proved the durability and adaptability of the deferent/epicycle device for representing planetary motion. The deferent/epicycle models worked as well as they did because of the extraordinary orbital stability of the solar system. Either theory could be used today and might still be in use had Isaac Newton not invented Physics and the Calculus.^[11]

The first planetary model without any epicycles was that of Ibn Bajjah (Avempace) in 12th century Andalusian Spain,^[12] but epicycles were not eliminated in Europe until the 17th century, when Johannes Kepler's model of elliptical orbits gradually replaced Copernicus' model based on perfect circles.

Newtonian or Classical Mechanics eliminated the need for deferent/epicycle methods altogether and produced theories many times more powerful. By treating the Sun and planets as point masses and using Newton's law of universal gravitation, equations of motion were derived that could be solved by various means to compute predictions of planetary orbital velocities and positions. Simple two-body problems, for example, can be solved analytically. More-complex n-body problems require numerical methods for solution.

The power of Newtonian mechanics to solve problems in orbital mechanics is illustrated by the discovery of Neptune. Analysis of observed perturbations in the orbit of Uranus produced estimates of the suspected planet's position within a degree of where it was found. This could not have been accomplished with deferent/epicycle methods. Still, Newton in 1702 published Theory of the Moon's Motion which employed an epicycle and remained in use in China into the ninetheenth century. Subsequent tables based on Newton's Theory could have approached arcminute accuracy.^[13]

Epicycles on epicycles

According to one school of thought in the history of astronomy, minor imperfections in the original Ptolemaic system were discovered through observations accumulated over time. It was mistakenly believed that more levels of epicycles (circles within circles) were added to the models to match more accurately the observed planetary motions. The multiplication of epicycles is believed to have led to a nearly unworkable system by the 16th century, and that Copernicus created his heliocentric system in order to simplify the Ptolemaic astronomy of his day, thus succeeding in drastically reducing the number of circles.

With better observations additional epicycles and eccentrics were used to represent the newly observed phenomena till in the later Middle Ages the universe became a 'Sphere/With Centric and Eccentric scribbled o'er,/Cycle and Epicycle, Orb in Orb'-- ^[14]

As a measure of complexity, the number of circles is given as 80 for Ptolemy, versus a mere 34 for Copernicus.^[15] The highest number appeared in the Encyclopaedia Britannica on Astronomy during the 1960s, in a discussion of King Alfonso X of Castile's interest in astronomy during the 13th century. (Alfonso is credited with commissioning the Alfonsine Tables.)

By this time each planet had been provided with from 40 to 60 epicycles to represent after a fashion its complex movement among the stars. Amazed at the difficulty of the project, Alfonso is credited with the remark that had he been present at the Creation he might have given excellent advice. ^[16]

As it turns out, a major difficulty with this epicycles-on-epicycles theory is that historians examining books on Ptolemaic astronomy from the Middle Ages and the Renaissance have found absolutely no trace of multiple epicycles being used for each planet. The Alfonsine Tables, for instance, were apparently computed using Ptolemy's original unadorned methods.^[17]

Another problem is that the models themselves discouraged tinkering. In a deferent/epicycle model, the parts of the whole are interrelated. A change in a parameter to improve the fit in one place would throw off the fit somewhere else. Ptolemy's model is probably optimal in this regard. On the whole it gave good results but missed a little here and there. Experienced astronomers would have recognized these shortcomings and allowed for them.

Slang for Bad Science

In part, due to misunderstandings about how deferent/epicycle models worked, "adding epicycles" has come to be used as a derogatory comment in modern scientific discussion. The term might be used, for example, to describe continuing to try to adjust a theory to make its predictions match the facts. According to this notion, epicycles are regarded by some as the paradigmatic example of Bad Science.^[18] Part of the problem may be due to the misconception of the epicycle as an explanation of a body's motion rather than merely a description. Toomer explains as follows,

"Whereas we use 'hypothesis' to denote a tentative theory which is still to be verified, Ptolemy usually means by ύπόθεσις something more like 'model', 'system of explanation', often indeed referring to 'the hypotheses which we have demonstrated'."^[19]

Legacy

Much of the content of the epicycle system was taken over by its successors and is still in use today. Some of the terms used in modern astronomy are anomaly, equation, mean motion, eccentricity, apogee and perigee.

Mathematical Formalism

According to the historian of science Norwood Russell Hanson:

There is no bilaterally-symmetrical, nor excentrically-periodic curve used in any branch of astrophysics or observational astronomy which could not be smoothly plotted as the resultant motion of a point turning within a constellation of epicycles, finite in number, revolving around a fixed deferent.^[20]

Any path—periodic or not, closed or open—can be represented with an infinite number of epicycles.

This is because epicycles can be represented as a complex Fourier series; so, with a large number epicycles, very complicated paths can be represented in the complex plane.^[21]

Let the complex number

$z_0=a_0 e^{i k_0 t}$,

where a and k are constants, $i=\sqrt{-1}$ is an imaginary number, and t is time, correspond to a deferent centered on the origin of the complex plane and revolving with a radius a and angular velocity

$k=\frac{2\pi}{T}$,

where T is the period.

If z₁ is the path of an epicycle, then the deferent plus epicycle is represented as the sum

$z_2=z_0+z_1=a_0 e^{i k_0 t}+a_1 e^{i k_1 t}$.

Generalizing to N epicycles yields

$z_N=\sum_{j=0}^N a_j e^{i k_j t}$,

which is a complex Fourier series. Finding the coefficients a_j to represent a time-dependent path in the complex plane, z = f(t), is the goal of reproducing an orbit with deferent and epicycles, and this is a way of "saving the phenomena" (σώζειν τα φαινόμενα).^[22]

Pertinent to the Copernican Revolution debate of "saving the phenomena" versus offering explanations, one can understand why Thomas Aquinas, in the 13th century, wrote:

Reason may be employed in two ways to establish a point: firstly, for the purpose of furnishing sufficient proof of some principle [...]. Reason is employed in another way, not as furnishing a sufficient proof of a principle, but as confirming an already established principle, by showing the congruity of its results, as in astronomy the theory of eccentrics and epicycles is considered as established, because thereby the sensible appearances of the heavenly movements can be explained; not, however, as if this proof were sufficient, forasmuch as some other theory might explain them. [...]^[23]

See also

• Epicycloid
• Occam's razor
• Scientific method

Notes

1. ^ For an example of the complexity of the problem, see Owen Gingerich, The Book Nobody Read, Walker, 2004, p. 50
2. ^ Gingerich, Chapter 4
3. ^ One volume of de Revolutionibus was devoted to a description of the trigonometry used to make the transformation between geocentric and heliocentric coordinates.
4. ^ Gingerich, p. 267
5. ^ Gingerich, p. 54
6. ^ Robert Palter, Approach to the History of Astronomy, in Studies in the History and Philosophy of Science 1 (1970): 94.
7. ^ Owen Gingerich, Alfonso X as a Patron of Astronomy, in The Eye of Heaven: Ptolemy, Copernicus, Kepler (New York: American Institute of Physics, 1993), p. 125.
8. ^ Gingerich, Crisis versus Aesthetic in the Copernican Revolution, in Eye of Heaven, pp. 193–204.
9. ^ "The popular belief that Copernicus's heliocentric system constitutes a significant simplification of the Ptolemaic system is obviously wrong ... .[T]he Copernican models themselves require about twice as many circles as the Ptolemaic models and are far less elegant and adaptable.", Neugebauer, Otto (1969) [1957]. The Exact Sciences in Antiquity (2 ed.). Dover Publications. ISBN 978-0-486-22332-2. http://books.google.com/books?id=JVhTtVA2zr8C. , p. 204. This is an extreme estimate in favor of Ptolemy.
10. ^ Palter, Approach to the History of Astronomy, pp. 113–14.
11. ^ Interestingly, a deferent/epicycle model is used to compute Lunar positions needed to define modern Hindu calendars. See Nachum Dershovitz and Edward M. Reingold: Calendrical Calculations, Cambridge University Press, 1997, Chapter 14. (ISBN 0-521-56474-3)
12. ^ Bernard R. Goldstein (March 1972). Theory and Observation in Medieval Astronomy, Isis 63 (1), p. 39–47 [40-41].
13. ^ Kollerstrom, Nicholas (2000). Newton's Forgotten Lunar Theory. Green Lion Press. ISBN 1-888009-08-X. 
14. ^ Dorothy Stimson, The Gradual Acceptance of the Copernican Theory of the Universe (New York, 1917), p. 14. The quotation is from John Milton's Paradise Lost, Book 8, 11.82-85.
15. ^ Robert Palter, An Approach to the History of Early Astronomy
16. ^ Encyclopaedia Britannica, 1968, vol. 2, p. 645. This is identified as the highest number in Owen Gingerich, Alfonso X. Gingerich also expressed doubt about the quotation attributed to Alfonso. In The Book Nobody Read (p. 56), however, Gingerich relates that he challenged Encyclopaedia Britannica about the number of epicycles. Their response was that the original author of the entry had died and its source couldn't be verified.
17. ^ Gingerich, The Book Nobody Read (p. 57)
18. ^ See e.g., Kolb, Rocky, Blind Watchers of the Sky, Addison-Wesley, 1996. P. 299 (ISBN 0-201-48992-9)
19. ^ Toomer, G.J. (1998). Ptolemy's Almagest. Princeton University Press. p. 23. ISBN 0-691-00260-6. 
20. ^ Hanson, Norwood Russell (1960-06-01). "The Mathematical Power of Epicyclical Astronomy". Isis 51 (2): 150-158. ISSN 0021-1753. Archived from the original on 10-21-2011. http://www.u.arizona.edu/%7Eaversa/scholastic/Mathematical%20Power%20of%20Epicyclical%20Astronomy%20%28Hanson%29.pdf. Retrieved 2011-10-21. 
21. ^ See, e.g., this animation, which uses 1,000 epicycles to retrace the cartoon character Homer Simpson; cf. also Christián Carman's “Deferentes, epiciclos y adaptaciones.”
22. ^ Cf. Duhem, Pierre (1969). To save the phenomena, an essay on the idea of physical theory from Plato to Galileo. Chicago: University of Chicago Press. OCLC 681213472.  (excerpt).
23. ^ Summa Theologica, I q. 32 a. 1 ad 2

External links

• Ptolemaic System - at Rice University's Galileo Project

Animated Illustrations

• Java simulation of the Ptolemaic System - at Paul Stoddard's Animated Virtual Planetarium, Northern Illinois University
• Epicycle and Deferent Demo - at Rosemary Kennett's website at the University of Syracuse
• A flash animation showing epicycles with adjustable parameters and presets for various planets.
• An Applet showing the principle of the epicycle, with a side-by-side comparison of the geocentric and heliocentric models.