Tetrakis hexahedron

Tetrakis hexahedron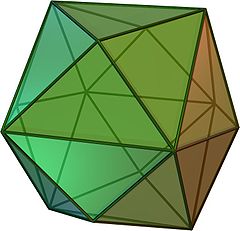
(Click here for rotating model)
Type	Catalan solid
Face type	isosceles triangle
Faces	24
Edges	36
Vertices	14
Vertices by type	6{4}+8{6}
Face configuration	V4.6.6
Symmetry group	Oh, [4,3], *432
Dihedral angle	143°7'48" $\arccos ( -\frac{4}{5} )$
Properties	convex, face-transitive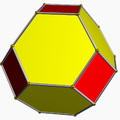
Truncated octahedron (dual polyhedron)	Net

In geometry, a tetrakis hexahedron is a Catalan solid. Its dual is the truncated octahedron, an Archimedean solid. It can be seen as a cube with square pyramids covering each square face; that is, it is the Kleetope of the cube.

It also can be called a disdyakis hexahedron as the dual of an omnitruncated tetrahedron.

Uses

Naturally occurring (crystal) formations of tetrahexahedrons are observed in copper and fluorite systems.

Polyhedral dice shaped like the tetrakis hexahedron are occasionally used by gamers.

A 24-cell viewed under a vertex-first perspective projection has a surface topology of a tetrakis hexahedron and the geometric proportions of the rhombic dodecahedron, with the rhombic faces divided into two triangles.

Gallery

• 

  As tiling by reflection domain of (2,3,3) triangle group

• 

  As a solid

See also

• Disdyakis triacontahedron
• Disdyakis dodecahedron
• Bisected hexagonal tiling
• Compound of three octahedra

References

• Williams, Robert (1979). The Geometrical Foundation of Natural Structure: A Source Book of Design. Dover Publications, Inc. ISBN 0-486-23729-X.  (Section 3-9)
• Wenninger, Magnus (1983), Dual Models, Cambridge University Press, ISBN 978-0-521-54325-5, MR730208  (The thirteen semiregular convex polyhedra and their duals, Page 14, Tetrakishexahedron)
• The Symmetries of Things 2008, John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, ISBN 978-1-56881-220-5 [1] (Chapter 21, Naming the Archimedean and Catalan polyhedra and tilings, page 284, Tetrakis hexahedron )

External links

• Eric W. Weisstein, Tetrakis hexahedron (Catalan solid) at MathWorld.
• Virtual Reality Polyhedra www.georgehart.com: The Encyclopedia of Polyhedra
  • VRML model
  • Conway Notation for Polyhedra Try: "dtO"
• Tetrakis Hexahedron -- Interactive Polyhedron model
• The Uniform Polyhedra

This polyhedron-related article is a stub. You can help Wikipedia by expanding it.v · Categories: Polyhedron stubs Catalan solids Wikimedia Foundation. 2010. Игры ⚽ Поможем написать реферат Fire in the Sky Tracy Tormé Look at other dictionaries: tetrakis-hexahedron — …   Useful english dictionary Tetrakis square tiling — Infobox face uniform tiling Type=Dual semiregular tiling Face List=45 45 90 triangle Symmetry Group=p4m or *442 Face Type=V4.8.8 Dual=Truncated square tiling Property List=face transitive In geometry, the tetrakis square tiling is a tiling of the …   Wikipedia Octahedral symmetry — The cube is the most common shape with octahedral symmetry A regular octahedron has 24 rotational (or orientation preserving) symmetries, and a symmetry order of 48 including transformations that combine a reflection and a rotation. A cube has… …   Wikipedia Truncated octahedron — (Click here for rotating model) Type Archimedean solid Uniform polyhedron Elements F = 14, E = 36, V = 24 (χ = 2) Faces by sides 6 …   Wikipedia Compound of three octahedra — The compound of three octahedra In mathematics, the compound of three octahedra or octahedron 3 compound is a polyhedral compound formed from three regular octahedra, all sharing a common center but rotated with respect to each other. Although… …   Wikipedia Catalanische Körper — Ein catalanischer Körper oder auch dual archimedischer Körper ist ein Körper, der sich zu einem archimedischen Körper dual verhält. So ist zum Beispiel das Rhombendodekaeder dual zum Kuboktaeder. Benannt sind catalanische Körper nach dem… …   Deutsch Wikipedia Dual-Archimedischer Körper — Ein catalanischer Körper oder auch dual archimedischer Körper ist ein Körper, der sich zu einem archimedischen Körper dual verhält. So ist zum Beispiel das Rhombendodekaeder dual zum Kuboktaeder. Benannt sind catalanische Körper nach dem… …   Deutsch Wikipedia Полуправильные многогранники — или Архимедовы тела  выпуклые многогранники, обладающие двумя свойствами: Все грани являются правильными многоугольниками двух или более типов (если все грани  правильные многоугольники одного типа, это  правильный многогранник); Для любой пары… …   Википедия List of mathematics articles (T) — NOTOC T T duality T group T group (mathematics) T integration T norm T norm fuzzy logics T schema T square (fractal) T symmetry T table T theory T.C. Mits T1 space Table of bases Table of Clebsch Gordan coefficients Table of divisors Table of Lie …   Wikipedia tetrakishexahedron — “+ noun Etymology: New Latin, from tetrakis + hexahedron : tetrahexahedron * * * tetrakishexahēˈdron noun (Gr tetrakis four times) a figure formed by erecting equal pyramids on all the faces of a cube • • • Main Entry: ↑tetra …   Useful english dictionary 18+ © Academic, 2000-2024 Contact us: Technical Support, Advertising Dictionaries export, created on PHP, Joomla, Drupal, WordPress, MODx. Mark and share Search through all dictionaries Translate… Search Internet Share the article and excerpts Direct link … Do a right-click on the link above and select “Copy Link”