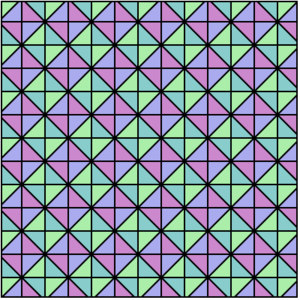
Tetrakis square tiling

Infobox face-uniform tiling

---

Type=Dual semiregular tiling
Face_List=45-45-90 triangle
Symmetry_Group=p4m
or *442
Face_Type=V4.8.8
Dual=Truncated square tiling
Property_List=face-transitive

In geometry, the tetrakis square tiling is a tiling of the Euclidean plane. It is square tiling with each square divided into four triangles from the center point.

Conway calls it a kisquadrille, reprsented by a kis operation that adds a center point and triangles to replace the faces of a square tiling (quadrille).

It is labeled V4.8.8 because each isosceles triangle face has two types of vertices: one with 4 triangles, and two with 8 triangles. It is the dual tessellation of the truncated square tiling which has one square and two octagons at each vertex.

It is topologically related to the polyhedron tetrakis hexahedron, V4.6.6

The symmetry type is:
*with the coloring: cmm; a primitive cell is 8 triangles, a fundamental domain 2 triangles (1/2 for each color)
*with the dark triangles in black and the light ones in white: p4g; a primitive cell is 8 triangles, a fundamental domain 1 triangle (1/2 each for black and white)
*with the edges in black and the interiors in white: p4m; a primitive cell is 2 triangles, a fundamental domain 1/2

See also

* Tilings of regular polygons
* List of uniform tilings

References

* John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, "The Symmetry of Things" 2008, ISBN 978-1-56881-220-5 [http://www.akpeters.com/product.asp?ProdCode=2205]
* (Chapter 2.1: "Regular and uniform tilings", p.58-65)
* Williams, Robert "The Geometrical Foundation of Natural Structure: A Source Book of Design" New York: Dover, 1979. p40