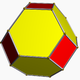
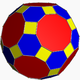
Cuboctahedron

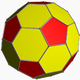
Cuboctahedron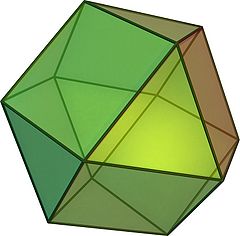
(Click here for rotating model)
Type	Archimedean solid Uniform polyhedron
Elements	F = 14, E = 24, V = 12 (χ = 2)
Faces by sides	8{3}+6{4}
Schläfli symbol	t1{4,3} t0,2{3,3}
Wythoff symbol	2 | 3 4 3 3 | 2
Coxeter-Dynkin
Symmetry	Oh, [4,3], (*432) Td, [3,3], (*332)
Dihedral Angle	125.26° $\sec^{-1} \left(-\sqrt{3}\right)$
References	U07, C19, W11
Properties	Semiregular convex quasiregular
Colored faces	3.4.3.4 (Vertex figure)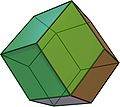
Rhombic dodecahedron (dual polyhedron)	Net

In geometry, a cuboctahedron is a polyhedron with eight triangular faces and six square faces. A cuboctahedron has 12 identical vertices, with two triangles and two squares meeting at each, and 24 identical edges, each separating a triangle from a square. As such it is a quasiregular polyhedron, i.e. an Archimedean solid, being vertex-transitive and edge-transitive.

Its dual polyhedron is the rhombic dodecahedron.

Other names

• Heptaparallelohedron (Buckminster Fuller)^[1]
  • Fuller applied the name "Dymaxion"^[1] and Vector Equilibrium^[2] to this shape, used in an early version of the Dymaxion map.
• With O_h symmetry, it is a rectified cube or rectified octahedron (Norman Johnson)
• With T_d symmetry, it is a cantellated tetrahedron.
• With D_3d symmetry, it is a triangular gyrobicupola.

Area and volume

The area A and the volume V of the cuboctahedron of edge length a are:

$A = (6+2\sqrt{3})a^2 \approx 9.4641016a^2$

$V = \frac{5}{3} \sqrt{2}a^3 \approx 2.3570226a^3.$

Images

Orthographic projections

Cartesian coordinates

The Cartesian coordinates for the vertices of a cuboctahedron (of edge length √2) centered at the origin are:

(±1,±1,0)

(±1,0,±1)

(0,±1,±1)

An alternate set of coordinates can be made in 4-space, as 12 permutations of:

(0,1,1,2)

This construction exists as one of 16 orthant facets of the cantellated 16-cell.

Root vectors

The cuboctahedron's 12 vertices can represent the root vectors of the simple Lie group A₃. With the addition of 6 vertices of the octahedron, these vertices represent the 18 root vectors simple Lie group B₃.

Geometric relations

A cuboctahedron can be obtained by taking an appropriate cross section of a four-dimensional 16-cell.

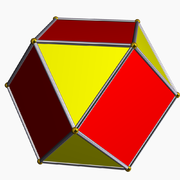
A cuboctahedron has octahedral symmetry. Its first stellation is the compound of a cube and its dual octahedron, with the vertices of the cuboctahedron located at the midpoints of the edges of either.

The cuboctahedron is a rectified cube and also a rectified octahedron.

It is also a cantellated tetrahedron. With this construction it is given the Wythoff symbol: 3 3 | 2.

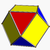
A skew cantellation of the tetrahedron produces a solid with faces parallel to those of the cuboctahedron, namely eight triangles of two sizes, and six rectangles. While its edges are unequal, this solid remains vertex-uniform: the solid has the full tetrahedral symmetry group and its vertices are equivalent under that group.

The edges of a cuboctahedron form four regular hexagons. If the cuboctahedron is cut in the plane of one of these hexagons, each half is a triangular cupola, one of the Johnson solids; the cuboctahedron itself thus can also be called a triangular gyrobicupola, the simplest of a series (other than the gyrobifastigium or "digonal gyrobicupola"). If the halves are put back together with a twist, so that triangles meet triangles and squares meet squares, the result is another Johnson solid, the triangular orthobicupola.

Both triangular bicupolae are important in sphere packing. The distance from the solid's centre to its vertices is equal to its edge length. Each central sphere can have up to twelve neighbors, and in a face-centered cubic lattice these take the positions of a cuboctahedron's vertices. In a hexagonal close-packed lattice they correspond to the corners of the triangular orthobicupola. In both cases the central sphere takes the position of the solid's centre.

Cuboctahedra appear as cells in three of the convex uniform honeycombs and in nine of the convex uniform polychora.

The volume of the cuboctahedron is 5/6 of that of the enclosing cube and 5/8 of that of the enclosing octahedron.

Related polyhedra

Cube

Truncated cube

cuboctahedron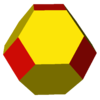

Truncated octahedron

Octahedron

The cuboctahedron shares its edges and vertex arrangement with two nonconvex uniform polyhedra: the cubohemioctahedron (having the square faces in common) and the octahemioctahedron (having the triangular faces in common). It also serves as a cantellated Tetrahedron, as being a rectified Tetratetrahedron.

Cuboctahedron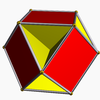

Cubohemioctahedron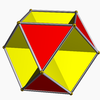

Octahemioctahedron

Name	tetrahedron	rectified tetrahedron (octahedron)	truncated tetrahedron	cantellated tetrahedron (cuboctahedron)	omnitruncated tetrahedron (truncated octahedron)	Snub tetrahedron (icosahedron)
Schläfli	{3,3}	t1{3,3}	t0,1{3,3}	t0,2{3,3}	t0,1,2{3,3}	s{3,3}
Coxeter-Dynkin
Graph (A3)
Graph (A2)
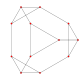
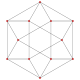
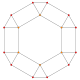
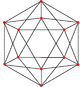
Solid
Tiling

The cuboctahedron 2-covers the tetrahemihexahedron,^[3] which accordingly has the same abstract vertex figure (2 triangles and two squares: 3.4.3.4) and half the vertices, edges, and faces. (The actual vertex figure of the tetrahemihexahedron is 3.4.3/2.4, with the a/2 factor due to the cross.)

Cuboctahedron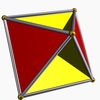

Tetrahemihexahedron

Related polytopes

The cuboctahedron can be decomposed into a regular octahedron and eight irregular but equal octahedra in the shape of the convex hull of a cube with two opposite vertices removed. This decomposition of the cuboctahedron corresponds with the cell-first parallel projection of the 24-cell into 3 dimensions. Under this projection, the cuboctahedron forms the projection envelope, which can be decomposed into 6 square faces, a regular octahedron, and 8 irregular octahedra. These elements correspond with the images of 6 of the octahedral cells in the 24-cell, the nearest and farthest cells from the 4D viewpoint, and the remaining 8 pairs of cells, respectively.

Cultural occurrences

• In the Star Trek episode "By Any Other Name", aliens seize the Enterprise by transforming crew members into inanimate cuboctahedra.

• The "Geo Twister" fidget toy [1] is a flexible cuboctahedron.

See also

• Cube
• Icosidodecahedron
• Octahedron
• Rhombicuboctahedron
• Truncated cuboctahedron

References

1. ^ ^{a b} http://dymaxion.org/dymaxion.shtml
2. ^ http://www.angelfire.com/mt/marksomers/92.html
3. ^ (Richter)

• Williams, Robert (1979). The Geometrical Foundation of Natural Structure: A Source Book of Design. Dover Publications, Inc. ISBN 0-486-23729-X.  (Section 3-9)
• Richter, David A., Two Models of the Real Projective Plane, http://homepages.wmich.edu/~drichter/rptwo.htm 

External links

• The Uniform Polyhedra
• Virtual Reality Polyhedra The Encyclopedia of Polyhedra
• Eric W. Weisstein, Cuboctahedron (Archimedean solid) at MathWorld.
• Richard Klitzing, 3D convex uniform polyhedra, o3x4o - co
• Editable printable net of a Cuboctahedron with interactive 3D view

Archimedean solids
Truncated tetrahedron Truncated cube Truncated octahedron Truncated dodecahedron Truncated icosahedron Cuboctahedron Icosidodecahedron Snub cube Rhombi- cuboctahedron Truncated cuboctahedron Truncated icosidodecahedron Rhomb- icosidodecahedron Snub dodecahedron