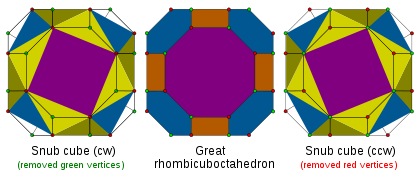
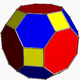
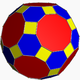
Snub cube

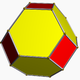
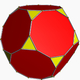
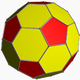
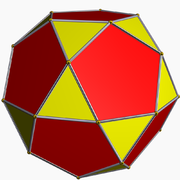
Snub cube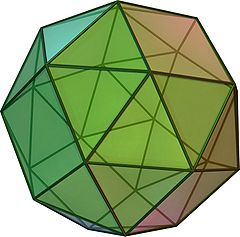
(Click here for rotating model)
Type	Archimedean solid Uniform polyhedron
Elements	F = 38, E = 60, V = 24 (χ = 2)
Faces by sides	(8+24){3}+6{4}
Schläfli symbol	s{4,3}
Wythoff symbol	| 2 3 4
Coxeter-Dynkin
Symmetry	O, [4,3]+, (432)
Dihedral Angle
References	U12, C24, W17
Properties	Semiregular convex chiral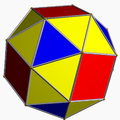
Colored faces	3.3.3.3.4 (Vertex figure)
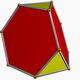
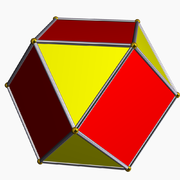
Pentagonal icositetrahedron (dual polyhedron)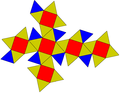	Net

In geometry, the snub cube, or snub cuboctahedron, is an Archimedean solid.

The snub cube has 38 faces, 6 of which are squares and the other 32 are equilateral triangles. It has 60 edges and 24 vertices. It is a chiral polyhedron, that is, it has two distinct forms, which are mirror images (or "enantiomorphs") of each other. The only other chiral Archimedean solid is the snub dodecahedron.

Dimensions

For a snub cube with edge length 1, its surface area is $6+8\sqrt{3}$ and its volume is $\sqrt{\frac{613t+203}{9(35t-62)}}$, where t is the tribonacci constant $\frac{1}{3}(1+\sqrt[3]{19-3\sqrt{33}}+\sqrt[3]{19+3\sqrt{33}}) \approx 1.83929$.

If the original snub cube has edge length 1, its dual pentagonal icositetrahedron has side lengths $\frac{1}{\sqrt{t+1}} \approx 0.593465$ and $\frac{1}{2}\sqrt{t+1} \approx 0.842509$.

Cartesian coordinates

Cartesian coordinates for the vertices of a snub cube are all the even permutations of

(±1, ±ξ, ±1/ξ)

with an even number of plus signs, along with all the odd permutations with an odd number of plus signs, where ξ is the real solution to

ξ³+ξ²+ξ=1,

which can be written

$\xi = \frac{1}{3}\left(\sqrt[3]{17+3\sqrt{33}} - \sqrt[3]{-17+3\sqrt{33}} - 1\right)$

or approximately 0.543689. ξ is the reciprocal of the tribonacci constant. Taking the even permutations with an odd number of plus signs, and the odd permutations with an even number of plus signs, gives a different snub cube, the mirror image.

This snub cube has edges of length α, a number which satisfies the equation

α⁶−4α⁴+16α²−32=0,

and can be written as

$\alpha = \sqrt{\frac{4}{3}-\frac{32}{6\sqrt[3]{2}\beta}+\frac{6\sqrt[3]{2}\beta}{9}}\approx1.60972$

$\beta = \sqrt[3]{13+3\sqrt{33}}$

For a snub cube with unit edge length, use the following coordinates instead:

$(\pm C_1,\pm C_2,\pm C_3)$

$C_1=\sqrt{\frac{1}{6}-\frac{1}{6c_1}+\frac{c_1}{12}}\approx0.621226$

$C_2=\sqrt{\frac{1}{3}-\frac{1}{6c_2}+\frac{c_2}{12}}\approx0.337754$

$C_3=\sqrt{\frac{1}{3}+\frac{1}{12c_3}+\frac{c_4}{12}}\approx1.14261$ --This formula is wrong, but the value correct.^{[improper synthesis?]}

$c_1=\sqrt[3]{3\sqrt{33}+17}$

$c_2=\sqrt[3]{3\sqrt{33}-17}$

$c_3=\sqrt[3]{199+3\sqrt{33}}$

$c_4=\sqrt[3]{199-3\sqrt{33}}$

Geometric relations

The snub cube can be generated by taking the six faces of the cube, pulling them outward so they no longer touch, then giving them each a small rotation on their centers (all clockwise or all counter-clockwise) until the spaces between can be filled with equilateral triangles.

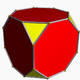
Cube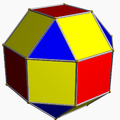	Rhombicuboctahedron (Expanded cube)

It can also be constructed as an alternation of a nonuniform omnitruncated cube, deleting every other vertex and creating new triangles at the deleted vertices. A properly proportioned (nonuniform) great rhombicuboctahedron will create equilateral triangles at the deleted vertices. Depending on which set of vertices are alternated, the resulting snub cube can have a clockwise or counterclockwise twist.

A "improved" snub cube, with a slightly smaller square face and slightly larger triangular faces compared to Archimedes' uniform snub cube, is useful as a spherical design.^[1]

Related polyhedra and tilings

This semiregular polyhedron is part of sequence of snubbed polyhedra and tilings with vertex figure (3.3.3.3.p) and Coxeter-Dynkin diagram , which can be constructed as alternations of the omnitruncation sequence .

232	332	432	532	632	732	832
(4.6.4)	(4.6.6)	(4.6.8)	(4.6.10)	4.6.12	4.6.14	4.6.16
(3.3.3.3.2)	(3.3.3.3.3)	(3.3.3.3.4)	(3.3.3.3.5)	3.3.3.3.6	3.3.3.3.7	3.3.3.3.8

See also

• Truncated cube
• Snub square tiling

References

1. ^ "Spherical Designs" by R. H. Hardin and N. J. A. Sloane

• Jayatilake, Udaya (March 2005). "Calculations on face and vertex regular polyhedra". Mathematical Gazette 89 (514): 76–81. 
• Williams, Robert (1979). The Geometrical Foundation of Natural Structure: A Source Book of Design. Dover Publications, Inc. ISBN 0-486-23729-X.  (Section 3-9)

External links

• Eric W. Weisstein, Snub cube (Archimedean solid) at MathWorld.
• Richard Klitzing, 3D convex uniform polyhedra, s3s4s - snic
• The Uniform Polyhedra
• Virtual Reality Polyhedra The Encyclopedia of Polyhedra
• Editable printable net of a Snub Cube with interactive 3D view

Archimedean solids
Truncated tetrahedron Truncated cube Truncated octahedron Truncated dodecahedron Truncated icosahedron Cuboctahedron Icosidodecahedron Snub cube Rhombi- cuboctahedron Truncated cuboctahedron Truncated icosidodecahedron Rhomb- icosidodecahedron Snub dodecahedron