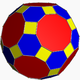
Truncated cuboctahedron

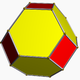
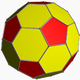
Truncated cuboctahedron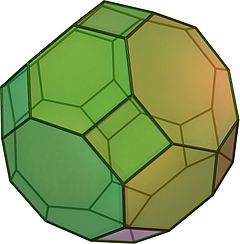
(Click here for rotating model)
Type	Archimedean solid Uniform polyhedron
Elements	F = 26, E = 72, V = 48 (χ = 2)
Faces by sides	12{4}+8{6}+6{8}
Schläfli symbol	t0,1,2{4,3}
Wythoff symbol	2 3 4 |
Coxeter-Dynkin
Symmetry	Oh, [4,3], (*432)
Dihedral Angle
References	U11, C23, W15
Properties	Semiregular convex zonohedron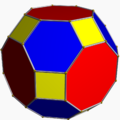
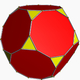
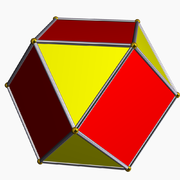
Colored faces	4.6.8 (Vertex figure)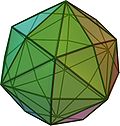
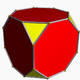
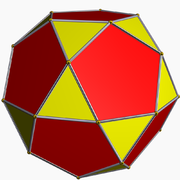
Disdyakis dodecahedron (dual polyhedron)	Net

In geometry, the truncated cuboctahedron is an Archimedean solid. It has 12 square faces, 8 regular hexagonal faces, 6 regular octagonal faces, 48 vertices and 72 edges. Since each of its faces has point symmetry (equivalently, 180° rotational symmetry), the truncated cuboctahedron is a zonohedron.

Cartesian coordinates

Orthographic projections

The Cartesian coordinates for the vertices of a truncated cuboctahedron having edge length 2 and centered at the origin are all permutations of:

(±1, ±(1+√2), ±(1+2√2))

Area and volume

The area A and the volume V of the truncated cuboctahedron of edge length a are:

$A = 12(2+\sqrt{2}+\sqrt{3}) a^2 \approx 61.7551724a^2$

$V = (22+14\sqrt{2}) a^3 \approx 41.7989899a^3.$

Vertices

To derive the number of vertices, we note that each vertex is the meeting point of a square, hexagon, and octagon.

• Each of the 12 squares with their 4 vertices contribute 48 vertices because $12 \times 4=48$.
• Each of the 8 hexagons with their 6 vertices contribute 48 vertices because $8 \times 6=48$.
• Each of the 6 octagons with their 8 vertices contribute 48 vertices because $6 \times 8=48$.

Therefore, there may seem to exist 48 + 48 + 48 = 144 vertices. However, we have over-counted the vertices thrice since a square, hexagon, and octagon meet at each vertex. Consequently, we divide 144 by 3 to correct for our over-counting: 144 / 3 = 48.

Dual

If the original truncated cuboctahedron has edge length 1, its dual disdyakis dodecahedron has edge lengths $\frac{2}{7}\sqrt{30-3\sqrt{2}}$, $\frac{3}{7}\sqrt{6(2+\sqrt{2})}$ and $\frac{2}{7}\sqrt{6(10+\sqrt{2})}$.

Uniform colorings

There is only one uniform coloring of the faces of this polyhedron, one color for each face type.

A 2-uniform coloring also exists with alternately colored hexagons.

Other names

Alternate interchangeable names are:

• Truncated cuboctahedron (Johannes Kepler)
• Rhombitruncated cuboctahedron (Magnus Wenninger^[1])
• Great rhombicuboctahedron (Robert Williams^[2])
• Great rhombcuboctahedron (Peter Cromwell^[3])
• Omnitruncated cube or cantitruncated cube (Norman Johnson)

The name truncated cuboctahedron, given originally by Johannes Kepler, is a little misleading. If you truncate a cuboctahedron by cutting the corners off, you do not get this uniform figure: some of the faces will be rectangles. However, the resulting figure is topologically equivalent to a truncated cuboctahedron and can always be deformed until the faces are regular.

The alternative name great rhombicuboctahedron refers to the fact that the 12 square faces lie in the same planes as the 12 faces of the rhombic dodecahedron which is dual to the cuboctahedron. Compare to small rhombicuboctahedron.

One unfortunate point of confusion: There is a nonconvex uniform polyhedron by the same name. See nonconvex great rhombicuboctahedron.

See also

• cube
• cuboctahedron
• octahedron
• truncated icosidodecahedron
• Truncated octahedron - truncated tetratetrahedron

Notes

1. ^ Wenninger, Magnus (1974), Polyhedron Models, Cambridge University Press, ISBN 978-0-521-09859-5, MR0467493  (Model 15, p. 29)
2. ^ Williams, Robert (1979). The Geometrical Foundation of Natural Structure: A Source Book of Design. Dover Publications, Inc. ISBN 0-486-23729-X.  (Section 3-9, p. 82)
3. ^ Cromwell, P.; Polyhedra, CUP hbk (1997), pbk. (1999). (p. 82)

External links

• Eric W. Weisstein, Great rhombicuboctahedron (Archimedean solid) at MathWorld.
• Richard Klitzing, 3D convex uniform polyhedra, x3x4x - girco
• Editable printable net of a truncated cuboctahedron with interactive 3D view
• The Uniform Polyhedra
• Virtual Reality Polyhedra The Encyclopedia of Polyhedra
• great Rhombicuboctahedron: paper strips for plaiting

Archimedean solids
Truncated tetrahedron Truncated cube Truncated octahedron Truncated dodecahedron Truncated icosahedron Cuboctahedron Icosidodecahedron Snub cube Rhombi- cuboctahedron Truncated cuboctahedron Truncated icosidodecahedron Rhomb- icosidodecahedron Snub dodecahedron