Conway polyhedron notation

This example chart shows how 11 new forms can be derived from the cube using 3 operations. The new polyhedra are shown as maps on the surface of the cube so the topological changes are more apparent. Vertices are marked in all forms with circles.

Conway polyhedron notation is used to describe polyhedra based on a seed polyhedron modified by various operations.

The seed polyhedra are the Platonic solids, represented by their first letter of their name (T,O,C,I,D); the prisms (Pn), antiprisms (An) and pyramids (Yn). Any convex polyhedron can serve as a seed, as long as the operations can be executed on it.

John Conway extended the idea of using operators, like truncation defined by Kepler, to build related polyhedra of the same symmetry. His descriptive operators can generate all the Archimedean solids and Catalan solids from regular seeds. Applied in a series, these operators allow many higher order polyhedra to be generated.

Operations on polyhedra

Elements are given from the seed (v,e,f) to the new forms, assuming seed is a convex polyhedron: (a topological sphere, Euler characteristic=2)

Operator	Name	Alternate construction	vertices	edges	faces	Description
	Seed		v	e	f	Seed form
r	Reflect (Hart)		v	e	f	Mirror image for chiral forms
d	dual		f	e	v	dual of the seed polyhedron - each vertex creates a new face
a	ambo		e	2e	2+e	The edges are new vertices, while old vertices disappear. (rectify)
j	join	da	e+2	2e	e	The seed is augmented with pyramids at a height high enough so that 2 coplanar triangles from 2 different pyramids share an edge.
t	truncate	dkd	2e	3e	e+2	truncate all vertices.
--	--	dk	2e	3e	e+2	Dual of kis, (bitruncation)
--	--	kd	e+2	3e	2e	Kis of dual
k	kis	dtd	e+2	3e	2e	raises a pyramid on each face.
c	chamfer		e+v	4e	2e+f	New hexagonal faces are added in place of edges.
-	-	dc	2e+f	4e	e+v
e	expand	aa	2e	4e	2e+2	Each vertex creates a new face and each edge creates a new quadrilateral. (cantellate)
o	ortho	de	2e+2	4e	2e	Each n-gon faces are divided into n quadrilaterals.
p	propellor (Hart)		v+2e	4e	e+f	A face rotation that creates quadrilaterals at vertices (self-dual)
-	-	dp	e+f	4e	v+2e
s	snub	dg	2e	5e	3e+2	"expand and twist" - each vertex creates a new face and each edge creates two new triangles
g	gyro	ds	3e+2	5e	2e	Each n-gon face is divided into n pentagons.
b	bevel	ta	4e	6e	2e+2	New faces are added in place of edges and vertices, Omnitruncation (Known as cantitruncation in higher polytopes).
m	meta	db & kj	2e+2	6e	4e	n-gon faces are divided into 2n triangles

Special forms

The kis operator has a variation, kn, which only adds pyramids to n-sided faces.

The truncate operator has a variation, tn, which only truncates order-n vertices.

The operators are applied like functions from right to left. For example:

• the dual of a tetrahedron is dT;
• the truncation of a cube is t3C or tC;
• the truncation of a Cuboctahedron is t4aC or taC.

All operations are symmetry-preserving except twisting ones like s and g which lose reflection symmetry.

Examples

The cube can generate all the convex Octahedral symmetry uniform polyhedra. The first row generates the Archimedean solids and the second row the Catalan solids, the second row forms being duals of the first. Comparing each new polyhedron with the cube, each operation can be visually understood. (Two polyhedron forms don't have single operator names given by Conway.)

Cube "seed"	ambo (rectify)	truncate	bitruncate	expand (cantellate)	bevel (omnitruncate)	snub
C	aC = djC	tC = dkdC	tdC = dkC	eC = aaC = doC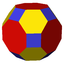	bC = taC = dmC = dkjC	sC = dgC
dual	join		kis (vertex-bisect)	ortho (edge-bisect)	meta (full-bisect)	gyro
dC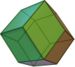	jC = daC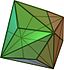	kdC = dtC	kC = dtdC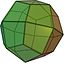	oC = deC = daaC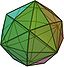	mC = dbC = kjC	gC = dsC

Generating regular seeds

All of the five regular polyhedra can be generated from prismatic generators with zero to two operators:

• Triangular pyramid: Y3 (A tetrahedron is a special pyramid)
  • T = Y3
  • O = aY3 (Rectified tetrahedron)
  • C = daY3 (dual to rectified tetrahedron)
  • I = sY3 (snub tetrahedron)
  • D = dsY3 (dual to snub tetrahedron)
• Triangular antiprism: A3 (An octahedron is a special antiprism)
  • O = A3
  • C = dA3
• Square prism: P4 (A cube is a special prism)
  • C = P4
• Pentagonal antiprism: A5
  • I = k5A5 (A special gyroelongated dipyramid)
  • D = t5dA5 (A special truncated trapezohedron)

Extensions to Conway's symbols

The above operations allow all of the semiregular polyhedrons and Catalan solids to be generated from regular polyhedrons. Combined many higher operations can be made, but many interesting higher order polyhedra require new operators to be constructed.

For example, geometric artist George W. Hart created an operation he called a propellor, and another reflect to create mirror images of the rotated forms.

• p - "propellor" (A rotation operator that creates quadrilaterals at the vertices). This operation is self-dual: dpX=pdX.
• r - "reflect" - makes the mirror image of the seed; it has no effect unless the seed was made with s or p.

Geometric coordinates of derived forms

In general the seed polyhedron can be considered a tiling of a surface since the operators represent topological operations so the exact geometric positions of the vertices of the derived forms are not defined in general. A convex regular polyhedron seed can be considered a tiling on a sphere, and so the derived polyhedron can equally be assumed to be positioned on the surface of a sphere. Similar a regular tiling on a plane, such as a hexagonal tiling can be a seed tiling for derived tilings. Nonconvex polyhedra can become seeds if a related topological surface is defined to constrain the positions of the vertices. For example torus-shaped polyhedra can derive other polyhedra with point on the same torus surface.

Example: A dodecahedron seed as a spherical tiling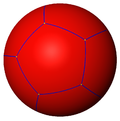

D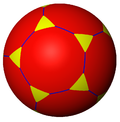	tD	aD	tdD	eD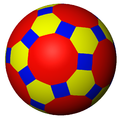	teD	sD
dD					dteD

Example: An Euclidean hexagonal tiling seed (H)

H	tH	aH	tdH = H	eH	teH	sH
dH	dtH	daH	dtdH = dH	deH	dteH	dsH

Example: A transparent Tetrahedron seed (T)

T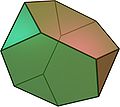	tT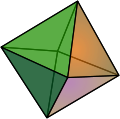	aT	tdT	eT	bT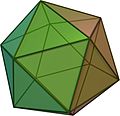	sT
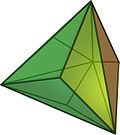
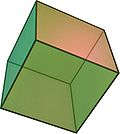
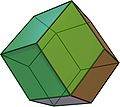
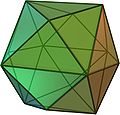
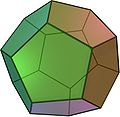
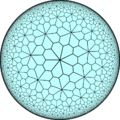
dT	dtT	jT	kT	oT	mT	gT

Example: A hyperbolic heptagonal tiling seed

{7,3} "seed"	truncate	ambo (rectify)	bitruncate	expand (cantellate)	bevel (omnitruncate)	snub
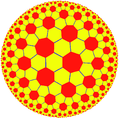
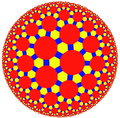
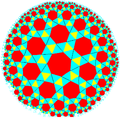
dual		join	kis (vertex-bisect)	ortho (edge-bisect)	meta (full-bisect)	gyro

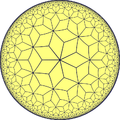
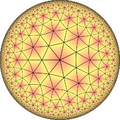
Other polyhedra

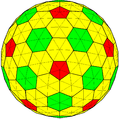
Iterating operators on simple forms can produce progressively larger polyhedra, maintaining the fundamental symmetry of the seed element. The vertices are assumed to be on the same spherical radius. Some generated forms can exist as spherical tilings, but fail to produce polyhedra with planar faces.

Tetrahedral symmetry

• 

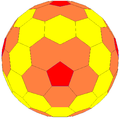
  Truncated triakis tetrahedron
  "t6dtT"

Octahedral symmetry

• 

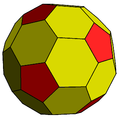
  Truncated rhombic dodecahedron
  "t4daC"

• 

  Tetrakis cuboctahedron
  "k4aC"

Icosahedral symmetry

Rhombic:

• 

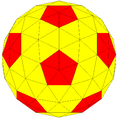
  Rhombic enneacontahedron
  "dakD"

Triangular:

• 

  Pentakis dodecahedron
  "kD"

• 

  Pentakis icosidodecahedron
  "k5aD"

• 

  "k6k5tI"

• 

  "kt5daD"

• 

  "kdktI"
  "dtktI"

• 

  "kdkt5daD"

Dual triangular:

• 

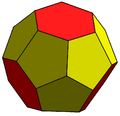
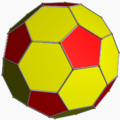
  Truncated icosahedron
  "dkD"

• 

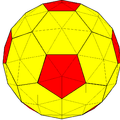
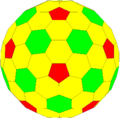
  Truncated rhombic triacontahedron
  "t5daD"
  "cD"

• 

  "dk6k5tI"

• 

  "dkt5daD"

• 

  "tktI"

• 

  "tkt5daD"

Triangular chiral:

• 

  Pentagonal hexecontahedron
  "dsD"

• 

  Pentakis snub dodecahedron
  "k5sD"

• 

  "k5k6stI"
  "kdk5sD"

Dual triangular chiral:

• 

  "sD"

• 

  "dk5sD"

• 

  "dk5k6stI"
  "tk5sD"

See also

• Uniform polyhedra
• Computer graphics algorithms:
  • Doo–Sabin subdivision surface - expand operator
  • Catmull–Clark subdivision surface - ortho operator

References

• George W. Hart, Sculpture based on Propellorized Polyhedra, Proceedings of MOSAIC 2000, Seattle, WA, August, 2000, pp. 61-70 [1]
• John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, The Symmetries of Things 2008, ISBN 978-1-56881-220-5
  • Chapter 21: Naming the Archimedean and Catalan polyhedra and Tilings

External links and references

• George Hart's Conway interpreter: generates polyhedra in VRML, taking Conway notation as input
  • Polyhedra Names
• polyHédronisme: generates polyhedra in HTML5 canvas, taking Conway notation as input
• Weisstein, Eric W., "Conway Polyhedron Notation" from MathWorld.
• John Conway's notation
• Weisstein, Eric W., "Truncation" from MathWorld. (truncate)
• Weisstein, Eric W., "Rectification" from MathWorld. (ambo)
• Weisstein, Eric W., "Cumulation or Apiculation" from MathWorld. (kis)
• Conway operators, PolyGloss, Wendy Krieger
• Derived Solids