Hysteresis

Not to be confused with Hysteria.

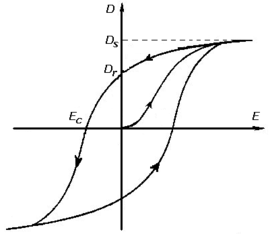
Fig. 1. Electric displacement field D of a ferroelectric material as the electric field E is first decreased, then increased. The curves form a hysteresis loop.

Hysteresis is the dependence of a system not just on its current environment but also on its past. This dependence arises because the system can be in more than one internal state. To predict its future evolution, either its internal state or its history must be known.^[1] If a given input alternately increases and decreases, the output tends to form a loop as in Fig. 1. However, loops may also occur because of a dynamic lag between input and output. Often, this effect is also referred to as hysteresis, or rate-dependent hysteresis. This effect disappears as the input changes more slowly, so many experts do not regard it as true hysteresis.

Hysteresis occurs in ferromagnetic materials and ferroelectric materials, as well as in the deformation of some materials (such as rubber bands and shape-memory alloys) in response to a varying force. In natural systems hysteresis is often associated with irreversible thermodynamic change. Many artificial systems are designed to have hysteresis: for example, in thermostats and Schmitt triggers, hysteresis is produced by positive feedback to avoid unwanted rapid switching. Hysteresis has been identified in many other fields, including economics and biology.

History

The term "hysteresis" is derived from ὑστέρησις, an ancient Greek word meaning "deficiency" or "lagging behind". It was coined by Sir James Alfred Ewing.

Some early work on describing hysteresis in mechanical systems was performed by James Clerk Maxwell. Subsequently, hysteretic models have received significant attention in the works of Ferenc Preisach (Preisach model of hysteresis), Louis Néel and D. H. Everett in connection with magnetism and absorption. A simple parametric description of various hysteretic loops may be found in ref.^[2] (with the model, substitution of rectangle, triangle or trapezoidal pulses instead of the harmonic functions also allows piecewise-linear hysteresis loops frequently used in discrete automatics to be built, see example in Fig. 4). More formal mathematical theory of systems with hysteresis was developed in 1970s by a group of Russian mathematicians led by Mark Krasnosel'skii, one of the founders of nonlinear analysis. He suggested an investigation of hysteresis phenomena using the theory of nonlinear operators.^[3]

Types of hysteresis

Rate-dependent

The meaning of hysteresis closest to its etymology is a lag between input and output. A simple example would be a sinusoidal input X(t) and output Y(t) that are separated by a phase lag φ:

$\begin{align} X(t) &= X_0 \sin \omega t \\ Y(t) &= Y_0 \sin\left(\omega t-\phi\right). \end{align}$

Such behavior can occur in linear systems, and a more general form of response is

$Y(t) = \chi_\text{i} X(t) + \int_{-\infty}^t \Phi_\text{d} (t-\tau) H(\tau) d\tau,$

where χ_i is the instantaneous response and Φ_d(t-τ) is the response at time t to an impulse at time τ. In the frequency domain, input and output are related by a complex generalized susceptibility.^[4]

This kind of hysteresis is often referred to as rate-dependent hysteresis. If the input is reduced to zero, the output has a transient response. This constitutes a memory of the past, but a limited one because it disappears as the transient decays to zero. The phase lag depends on the frequency of change in the input, and goes to zero as the frequency decreases.^[4]

In physical systems, rate-dependent hysteresis is often due to dissipative effects like friction and is associated with power loss.^[4]

Rate-independent

Systems with rate-independent hysteresis have a persistent memory of the past that remains after the transients have died out.^[5] The future evolution of such a system depends on the past. If an input variable X cycles from X₀ to X₁ and back, the output Y(X) may be Y₀ initially and a different value Y₂ on the return. The values of Y(X) depend on the values that X passes through, but not on the rate of change of X.^[4] Many authors define hysteresis as rate-independent hysteresis.^[6]

Hysteresis in engineering

Control systems

Fig. 4. Sharp hysteresis loop of a Schmitt trigger

Hysteresis can be used to filter signals so that the output reacts slowly by taking recent history into account. For example, a thermostat controlling a heater may turn the heater on when the temperature drops below A degrees, but not turn it off until the temperature rises above B degrees (e.g., if one wishes to maintain a temperature of 20 °C, then one might set the thermostat to turn the furnace on when the temperature drops below 18 °C, and turn it off when the temperature exceeds 22 °C). This thermostat has hysteresis. Thus the on/off output of the thermostat to the heater when the temperature is between A and B depends on the history of the temperature. This prevents rapid switching on and off as the temperature drifts around the set point. The furnace is either off or on, with nothing in between. The thermostat is a system; the input is the temperature, and the output is the furnace state. If the temperature is 21 °C, then it is not possible to predict whether the furnace is on or off without knowing the history of the temperature.

Similarly a pressure switch also exhibits hysteresis. Its pressure setpoints are substituted for those of temperature corresponding to a thermostat.

Electronic circuits

A Schmitt trigger is a simple electronic circuit that also exhibits this property. Often, some amount of hysteresis is intentionally added to an electronic circuit to prevent unwanted rapid switching. This and similar techniques are used to compensate for contact bounce in switches, or noise in an electrical signal.

A latching relay uses a solenoid to actuate a ratcheting mechanism that keeps the relay closed even if power to the relay is terminated.

Hysteresis is essential to the workings of some memristors (circuit components which "remember" changes in the current passing through them by changing their resistance).^[7]

The hysteresis effect can be used when connecting complex circuits with the so-called passive matrix addressing. This scheme is praised^{[by whom?]} as a technique that can be used in modern nanoelectronics, electrochrome cells, memory effect, etc. In this scheme, shortcuts are made between adjacent components (see crosstalk) and the hysteresis helps to keep the components in a particular state while the other components change states. That is, one can address all rows at the same time instead of doing each individually.

In the field of audio electronics, a noise gate often implements hysteresis intentionally to prevent the gate from "chattering" when signals close to its threshold are applied.

User interface design

A hysteresis is sometimes intentionally added to computer algorithms. The field of user interface design has borrowed the term hysteresis to refer to times when the state of the user interface intentionally lags behind the apparent user input. For example, a menu that was drawn in response to a mouse-over event may remain on-screen for a brief moment after the mouse has moved out of the trigger region and the menu region. This allows the user to move the mouse directly to an item on the menu, even if part of that direct mouse path is outside of both the trigger region and the menu region. For instance, right-clicking on the desktop in most Windows interfaces will create a menu that exhibits this behavior.

Hysteresis in mechanics

Elastic hysteresis

Fig. 3. Elastic hysteresis of an idealized rubber band. The area in the centre of the hysteresis loop is the energy dissipated due to material plasticity.^{[dubious – discuss]}

Elastic hysteresis was one of the first types of hysteresis to be examined.^[8][9]

A simple way to understand it is in terms of a rubber band with weights attached to it. If the top of a rubber band is hung on a hook and small weights are attached to the bottom of the band one at a time, it will get longer. As more weights are loaded onto it, the band will continue to extend because the force the weights are exerting on the band is increasing. When each weight is taken off, or unloaded, it will get shorter as the force is reduced. As the weights are taken off, each weight that produced a specific length as it was loaded onto the band now produces a slightly longer length as it is unloaded. This is because the band does not obey Hooke's law perfectly. The hysteresis loop of an idealized rubber band is shown in Fig. 3.

In one sense the rubber band was harder to stretch when it was being loaded than when it was being unloaded. In another sense, as one unloads the band, the cause (the force of the weights) lags behind the effect (the length) because a smaller value of weight produces the same length. In another sense more energy was required during the loading than the unloading; that energy must have gone somewhere, it was dissipated or "lost" as heat.

Elastic hysteresis is more pronounced when the loading and unloading is done quickly than when it is done slowly.^[10] Some materials such as hard metals don't show elastic hysteresis under a moderate load, whereas other hard materials like granite and marble do. Materials such as rubber exhibit a high degree of elastic hysteresis.

A word of caution: rubber behaves like a gas. When the rubber band is stretched it heats up. If it is suddenly released, the rubber cools down, very easy to perceive just by touching. So, there is a large hysteresis from the thermal exchange with the environment and a smaller hysteresis due to internal friction within the rubber. This proper, intrinsic hysteresis could be measured only if adiabatic isolation of the rubber band is imposed.

Small vehicle suspensions using rubber (or other elastomers) can achieve the dual function of springing and damping because rubber, unlike metal springs, has pronounced hysteresis and does not return all the absorbed compression energy on the rebound. Mountain bikes have frequently made use of elastomer suspension, as did the original Mini car.

Contact angle hysteresis

The contact angle formed between a liquid and solid phase will exhibit a range of contact angles that are possible. There are two common methods for measuring this range of contact angles. The first method is referred to as the tilting base method. Once a drop is dispensed on the surface with the surface level, the surface is then tilted from 0° to 90°. As the drop is tilted, the downhill side will be in a state of imminent wetting while the uphill side will be in a state of imminent dewetting. As the tilt increases the downhill contact angle will increase and represents the advancing contact angle while the uphill side will decrease; this is the receding contact angle. The values for these angles just prior to the drop releasing will typically represent the advancing and receding contact angles. The difference between these two angles is the contact angle hysteresis. The second method is often referred to as the add/remove volume method. When the maximum liquid volume is removed from the drop without the interfacial area decreasing the receding contact angle is thus measured. When volume is added to the maximum before the interfacial area increases, this is the advancing contact angle. As with the tilt method, the difference between the advancing and receding contact angles is the contact angle hysteresis. Most researchers prefer the tilt method; the add/remove method requires that a tip or needle stay embedded in the drop which can affect the accuracy of the values, especially the receding contact angle.

Adsorption hysteresis

Hysteresis can also occur during physical adsorption processes. In this type of hysteresis, the quantity adsorbed is different when gas is being added than it is when being removed. The specific causes of adsorption hysteresis are still an active area of research, but it is linked to differences in the nucleation and evaporation mechanisms inside mesopores. These mechanisms are further complicated by effects such as cavitation and pore blocking.

In physical adsorption, hysteresis is evidence of mesoporosity-indeed, the definition of mesopores (2–50 nm) is associated with the appearance (50 nm) and disappearance (2 nm) of mesoporosity in nitrogen adsorption isotherms as a function of Kelvin radius.^[11] An adsorption isotherm showing hysteresis is said to be of Type IV (for a wetting adsorbate) or Type V (for a non-wetting adsorbate), and hysteresis loops themselves are classified according to how symmetric the loop is.^[12] Adsorption hysteresis loops also have the unusual property that it is possible to scan within a hysteresis loop by reversing the direction of adsorption while on a point on the loop. The resulting scans are called "crossing," "converging," or "returning," depending on the shape of the isotherm at this point.^[13]

Matric potential hysteresis

The relationship between matric water potential and water content is the basis of the water retention curve. Matric potential measurements (Ψ_m) are converted to volumetric water content (θ) measurements based on a site or soil specific calibration curve. Hysteresis is a source of water content measurement error. Matric potential hysteresis arises from differences in wetting behaviour causing dry medium to re-wet; that is, it depends on the saturation history of the porous medium. Hysteretic behaviour means that, for example, at a matric potential (Ψ_m) of 5 kPa, the volumetric water content (θ) of a fine sandy soil matrix could be anything between 8% to 25%.^[14]

Tensiometers are directly influenced by this type of hysteresis. Two other types of sensors used to measure soil water matric potential are also influenced by hysteresis effects within the sensor itself. Resistance blocks, both nylon and gypsum based, measure matric potential as a function of electrical resistance. The relation between the sensor’s electrical resistance and sensor matric potential is hysteretic. Thermocouples measure matric potential as a function of heat dissipation. Hysteresis occurs because measured heat dissipation depends on sensor water content, and the sensor water content–matric potential relationship is hysteretic. As of 2002^[update], only desorption curves are usually measured during calibration of soil moisture sensors. Despite the fact that it can be a source of significant error, the sensor specific effect of hysteresis is generally ignored.^[15]

Hysteresis in materials

Magnetic hysteresis

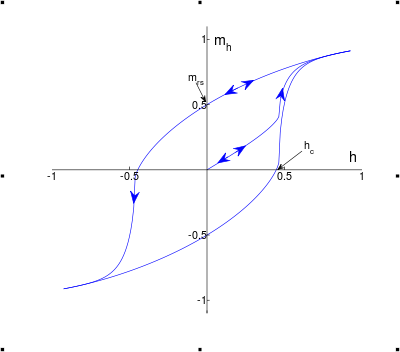Fig. 2. A plot of magnetization m against magnetic field h calculated using a theoretical model. Starting at the origin, the upward curve is the initial magnetization curve. The downward curve after saturation, along with the lower return curve, form the main loop. The intercepts h_c and m_rs are the coercivity and saturation remanence.

When an external magnetic field is applied to a ferromagnet such as iron, the atomic dipoles align themselves with it. Even when the field is removed, part of the alignment will be retained: the material has become magnetized. Once magnetized, the magnet will stay magnetized indefinitely. To demagnetize it requires heat or a magnetic field in the opposite direction. This is the effect that provides the element of memory in a hard disk drive.

The relationship between field strength H and magnetization M is not linear in such materials. If a magnet is demagnetized (H=M=0) and the relationship between H and M is plotted for increasing levels of field strength, M follows the initial magnetization curve. This curve increases rapidly at first and then approaches an asymptote called magnetic saturation. If the magnetic field is now reduced monotonically, M follows a different curve. At zero field strength, the magnetization is offset from the origin by an amount called the remanence. If the H-M relationship is plotted for all strengths of applied magnetic field the result is a hysteresis loop called the main loop. The width of the middle section is twice the coercivity of the material.^[16]

A closer look at a magnetization curve generally reveals a series of small, random jumps in magnetization called Barkhausen jumps. This effect is due to crystallographic defects such as dislocations.^[17]

Physical origin

Main article: Ferromagnetism

The phenomenon of hysteresis in ferromagnetic materials is the result of two effects: rotation of magnetization and changes in size or number of magnetic domains. In general, the magnetization varies (in direction but not magnitude) across a magnet, but in sufficiently small magnets, it does not. In these single-domain magnets, and the magnetization responds to a magnetic field by rotating. Single-domain magnets are used wherever a strong, stable magnetization is needed (for example, magnetic recording).

Larger magnets are divided into regions called domains. Across each domain, the magnetization does not vary; but between domains are relatively thin domain walls in which the direction of magnetization rotates from the direction of one domain to another. If the magnetic field changes, the walls move, changing the relative sizes of the domains. Because the domains are not magnetized in the same direction, the magnetic moment per unit volume is smaller than it would be in a single-domain magnet; but domain walls involve rotation of only a small part of the magnetization, so it is much easier to change the magnetic moment. The magnetization can also change by addition or subtraction of domains (called nucleation and denucleation).

Applications

Main article: Magnet#Common uses of magnets

There are a great variety of applications of the hysteresis in ferromagnets. Many of these make use of their ability to retain a memory, for example magnetic tape, hard disks, and credit cards. In these applications, hard magnets (high coercivity) like iron are desirable so the memory is not easily erased.

Soft magnets (low coercivity) are used as cores in electromagnets. The nonlinear response of the magnetic moment to a magnetic field boosts the response of the coil wrapped around it. The low coercivity reduces that energy loss associated with hysteresis.

Electrical hysteresis

Electrical hysteresis typically occurs in ferroelectric material, where domains of polarization contribute to the total polarization. Polarization is the electrical dipole moment (either C·m⁻² or C·m). The mechanism, an organization of the polarization into domains, is similar to that of magnetic hysteresis.

Liquid–solid-phase transitions

Hysteresis manifests itself in state transitions when melting temperature and freezing temperature do not agree. For example, agar melts at 85 °C and solidifies from 32 to 40 °C. This is to say that once agar is melted at 85 °C, it retains a liquid state until cooled to 40 °C. Therefore, from the temperatures of 40 to 85 °C, agar can be either solid or liquid, depending on which state it was before.

Hysteresis in biology

Cell biology and genetics

Main article: Cell biology

Cells undergoing cell division exhibit hysteresis in that it takes a higher concentration of cyclins to switch them from G2 phase into mitosis than to stay in mitosis once begun.^[18]

Main article: Chromatin

Darlington in his classic works on genetics^[19][20] discussed hysteresis of the chromosomes, by which he meant "failure of the external form of the chromosomes to respond immediately to the internal stresses due to changes in their molecular spiral", as they lie in a somewhat rigid medium in the limited space of the cell nucleus.

Main article: Morphogen

In developmental biology, cell type diversity is regulated by long range-acting signaling molecules called morphogens that pattern uniform pools of cells in a concentration- and time-dependent manner. The morphogen Sonic Hedgehog (Shh), for example, acts on limb bud and neural progenitors to induce expression of a set of homeodomain-containing transcription factors to subdivide these tissues into distinct domains. It has been shown that these tissues have a 'memory' of previous exposure to Shh.^[21] In neural tissue, this hysteresis is regulated by a homeodomain (HD) feedback circuit that amplifies Shh signaling.^[22] In this circuit, expression of Gli transcription factors, the executors of the Shh pathway, is suppressed. Glis are processed to repressor forms (GliR) in the absence of Shh, but in the presence of Shh, a proportion of Glis are maintained as full-length proteins allowed to translocate to the nucleus, where they act as activators (GliA) of transcription. By reducing Gli expression then, the HD transcription factors reduce the total amount of Gli (GliT), so a higher proportion of GliT can be stabilized as GliA for the same concentration of Shh.

Neuroscience

See also: Refractory period

The property by which some neurons do not return to their basal conditions from a stimulated condition immediately after removal of the stimulus is an example of hysteresis.

Respiratory physiology

Lung hysteresis is evident when observing the compliance of a lung on inspiration versus expiration. The difference in compliance (volume/pressure) is due to the additional energy required during inspiration to recruit and inflate additional alveoli.^[23]

The transpulmonary pressure vs Volume curve of inhalation is different from the Pressure vs Volume curve of exhalation, the difference being described as hysteresis. Lung volume at any given pressure during inhalation is less than the lung volume at any given pressure during exhalation.^[24]

Hysteresis in economics

Main article: Hysteresis (economics)

Economic systems can exhibit hysteresis. For example, export performance is subject to strong hysteresis effects: because of the fixed transportation costs it may take a big push to start a country's exports, but once the transition is made, not much may be required to keep them going.

Hysteresis is used extensively in the area of labor markets. According to theories based on hysteresis, economic downturns (recession) result in an individual becoming unemployed, losing his/her skills (commonly developed 'on the job'), demotivated/disillusioned, and employers may use time spent in unemployment as a screen. In times of an economic upturn or 'boom', the workers affected will not share in the prosperity, remaining long-term unemployed (over 52 weeks). Hysteresis has been put forward^{[by whom?]} as a possible explanation for the poor unemployment performance of many economies in the 1990s. Labor market reform, and/or strong economic growth, may not therefore aid this pool of long-term unemployed, and thus specific targeted training programs are presented as a possible policy solution.^{[citation needed]}

Permanently higher unemployment

Hysteresis is a hypothesized property of unemployment rates. It's possible that there is a ratchet effect, so a short-term rise in unemployment rates tends to persist.

An example is the notion that inflationary policy leads to a permanently higher 'natural' rate of unemployment (NAIRU), because inflationary expectations are 'sticky' downward due to wage rigidities and imperfections in the labour market.

When some negative shock reduces employment in a company or industry, there are fewer employed workers left. As usually the employed workers have the power to set wages, their reduced number incentivizes them to bargain for even higher wages when the economy again gets better, instead of letting the wage be at the equilibrium wage level, where the supply and demand of workers would match. This causes hysteresis, i.e., the unemployment becomes permanently higher after negative shocks.^[25]

Another channel through which hysteresis can occur is through learning by doing. Workers who lose their jobs due to a temporary shock may become permanently unemployed because they miss out on the job training and skill acquisition that normally takes place.

Hysteresis has been invoked, by Olivier Blanchard among others, as explaining the differences in long run unemployment rates between Europe and the United States.

Game theory and capital controls

Hysteresis occurs in applications of game theory to economics, in models with product quality, agent honesty or corruption of various institutions. Slightly different initial conditions can lead to opposite outcomes and resulting stable "good" and "bad" equilibria.

Another area where hysteresis phenomena are found is capital controls. A developing country can ban a certain kind of capital flow (e.g. engagement with international private equity funds), but when the ban is removed, the system takes a long time to return to the pre-ban state.

Additional considerations

Models of hysteresis

Each subject that involves hysteresis has models that are specific to the subject. In addition, there are models that capture general features of many systems with hysteresis.^[2][3] An example is the Preisach model of hysteresis, which represents a hysteresis nonlinearity as a superposition of square loops called hysterons.^[3]

The Bouc-Wen model of hysteresis is often used to describe non-linear hysteretic systems. It was introduced by Bouc^[26][27] and extended by Wen,^[28] who demonstrated its versatility by producing a variety of hysteretic patterns. This model is able to capture in analytical form, a range of shapes of hysteretic cycles which match the behaviour of a wide class of hysteretical systems; therefore, given its versability and mathematical tractability, the Bouc-Wen model has quickly gained popularity and has been extended and applied to a wide variety of engineering problems, including multi-degree-of-freedom (MDOF) systems, buildings, frames, bidirectional and torsional response of hysteretic systems two- and three-dimensional continua, and soil liquefaction among others. The Bouc-Wen model and its variants/extensions have been used in applications of structural control, in particular in the modeling of the behaviour of magnetorheological dampers, base isolation devices for buildings and other kinds of damping devices; it has also been in the modelling and analysis of structures built of reinforced concrete, steel, mansory and timber.^{[citation needed]}

Energy

When hysteresis occurs with extensive and intensive variables, the work done on the system is the area under the hysteresis graph.

See also

• Hysteresivity
• Path dependence
• Backlash (engineering)
• Bean's critical state model

Notes

1. ^ Mielke, A.; Roubicek, T. (2003). "A Rate-Independent Model for Inelastic Behavior of Shape-Memory Alloys". Multiscale Model. Simul. 1 (4): 571–597. doi:10.1137/S1540345903422860. 
2. ^ ^{a b} R. V. Lapshin (1995). "Analytical model for the approximation of hysteresis loop and its application to the scanning tunneling microscope" (PDF). Review of Scientific Instruments (USA: AIP) 66 (9): 4718–4730. Bibcode 1995RScI...66.4718L. doi:10.1063/1.1145314. ISSN 0034-6748. http://www.nanoworld.org/homepages/lapshin/publications.htm#analytical1995.  (Russian translation is available).
3. ^ ^{a b c} Mayergoyz 2003
4. ^ ^{a b c d} Bertotti 1998
5. ^ The term is attributed to Truesdell & Noll 1965 by Visintin 1994, page 13.
6. ^ Visintin 1994, page 13
7. ^ Johnson, R. Colin. "'Missing link' memristor created: Rewrite the textbooks?". EE Times April 30, 2008. http://www.eetimes.com/news/latest/showArticle.jhtml?articleID=207403521&pgno=1. Retrieved September 2011. 
8. ^ Augustus E. Love (1927). Treatise on the Mathematical Theory of Elasticity (Dover Books on Physics & Chemistry). New York: Dover Publications. ISBN 0-486-60174-9. 
9. ^ Ewing, J. A. (1889). "On hysteresis in the relation of strain to stress". British Association Reports: 502. 
10. ^ Hopkinson, B.; Williams, G. T. (1912). "The Elastic Hysteresis of Steel". Proceedings of the Royal Society 87 (598): 502. Bibcode 1912RSPSA..87..502H. doi:10.1098/rspa.1912.0104. 
11. ^ Gregg, S. J.; Sing, Kenneth S. W. (1982). Adsorption, Surface Area, and Porosity (Second ed.). London: Academic Press. ISBN 978-0123009562. 
12. ^ Sing, K. S. W.; Everett, D. H.; Haul, R. A. W.; Moscou, L.; Pierotti, R. A.; J. Roquérol, J.; Siemieniewska, T. (1985). "Reporting physisorption data for gas/solid systems with special reference to the determination of surface area and porosity (Recommendations 1984)". Pure and Applied Chemistry 57 (4): 603–619. doi:10.1351/pac198557040603. 
13. ^ Tompsett, G. A.; Krogh, L.; Griffin, D. W.; Conner, W. C. (2005). "Hysteresis and Scanning Behavior of Mesoporous Molecular Sieves". Langmuir 21 (8): 8214–8225. doi:10.1021/la050068y. PMID 16114924. 
14. ^ Parkes, Martin (8 April 1999). "Subject: Accuracy of capacitance soil moisture ...". SOWACS mailing list. http://www.sowacs.com/archives/99-04/msg00000.html. Retrieved 28 September 2011. 
15. ^ B. R., Scanlon; B. J., Andraski; J., Bilskie (2002). "Methods of soil analysis: Physical Methods: Miscellaneous methods for measuring matric or water potential" (PDF). Soil Science Society of America 4: 643–670. ISBN 0-89118-810-X. http://www.beg.utexas.edu/environqlty/vadose/pdfs/webbio_pdfs/Chapt3-2-4.pdf. Retrieved 2006-05-26. 
16. ^ Chikazumi 1997, Chapter 1
17. ^ Chikazumi 1997, Chapter 15
18. ^ Pomerening, Joseph R.; Sontag, Eduardo D.; Ferrell, Jr., James E. (2003). "Building a cell cycle oscillator: hysteresis and bistability in the activation of Cdc2". Nature Cell Biology 5 (4): 346–251. doi:10.1038/ncb954. PMID 12629549. 
19. ^ Darlington, C. D. (1937). Recent Advances in Cytology (Genes, Cells, & Organisms) (Second ed.). P. Blakiston's Son & Co.. ISBN 978-0824013769. http://www.biodiversitylibrary.org/ia/recentadvancesin00darl. 
20. ^ Rieger, R.; Michaelis, A.; M. M. (1968). A Glossary of Genetics and Cytogenetics : Classical and Molecular (Third ed.). Springer. ISBN 978-3540043164. 
21. ^ Harfe, B. D.; Scherz, P. J.; Nissim, S.; Tian, H.; McMahon, A. P.; Tabin, C. J. (2004). "Evidence for an expansion-based temporal Shh gradient in specifying vertebrate digit identities". Cell 118 (4): 517–28. doi:10.1016/j.cell.2004.07.024. PMID 15315763. 
22. ^ M., Lek; Dias, J. M.; Marklund, U.; Uhde, C. W.; Kurdija, S.; Lei, Q.; Sussel, L.; Rubenstein, J. L. et al. (2010). "A homeodomain feedback circuit underlies step-function interpretation of a Shh morphogen gradient during ventral neural patterning". Development 137 (23): 4051–4060. doi:10.1242/dev.054288. PMID 21062862. 
23. ^ Escolar, J. D.; Escola, A. (2004). "Lung histeresis: a morphological view". Histology and Histopathology Cellular and Molecular Biology 19 (1): 159–166. PMID 14702184. http://www.hh.um.es/pdf/Vol_19/19_1/Escolar-19-159-166-2004.pdf. Retrieved 1 March 2011. 
24. ^ West, John B. (2005). Respiratory physiology: the essentials. Hagerstown, MD: Lippincott Williams & Wilkins. ISBN 0-7817-5152-7. 
25. ^ Blanchard, Olivier J.; Summers, Lawrence H. (1986). "Hysteresis and the European Unemployment Problem". NBER Macroeconomics Annual 1: 15–78. doi:10.2307/3585159. http://www.nber.org/papers/w1950. 
26. ^ Bouc, R. (1967). "Forced vibration of mechanical systems with hysteresis". Proceedings of the Fourth Conference on Nonlinear Oscillation. Prague, Czechoslovakia. pp. 315. 
27. ^ Bouc, R. (1971). "Modèle mathématique d'hystérésis: application aux systèmes à un degré de liberté" (in French). Acustica 24,: 16–25. 
28. ^ Wen, Y. K. (1976). "Method for random vibration of hysteretic systems". Journal of Engineering Mechanics (American Society of Civil Engineers) 102 (2): 249–263. 

References

• Bertotti, Giorgio (1998). Hysteresis in magnetism: For physicists, materials scientists, and engineers. Academic Press. ISBN 978-0120932702. 
• Chikazumi, Sōshin (1997). Physics of Ferromagnetism. Clarendon Press. ISBN 0-19-851776-9. 
• Jiles, D. C.; Atherton, D. L. (1986). "Theory of ferromagnetic hysteresis". Journal of Magnetism and Magnetic Materials 61: 48–60. Bibcode 1986JMMM...61...48J. doi:10.1016/0304-8853(86)90066-1. 
• Krasnosel'skii, Mark; Pokrovskii, Alexei (1989). Systems with Hysteresis. New York: Springer-Verlag. ISBN 978-0387155432. 
• Mayergoyz, Isaak D. (2003). Mathematical Models of Hysteresis and their Applications: Second Edition (Electromagnetism). Academic Press. ISBN 978-0124808737. 
• Mayergoyz, Isaak D.; Bertotti, Giorgio, eds (2005). The Science of Hysteresis (3-volume set). Academic Press. ISBN 978-0124808744. 
• Truesdell, C.; Noll, Walter (2004). Antman, Stuart. ed. The Non-Linear Field Theories of Mechanics (Third ed.). ISBN 978-3-540-02779-9.  Originally published as Volume III/3 of Handbuch der Physik in 1965.
• Visintin, Augusto (1994). Differential models of hysteresis. Springer. ISBN 9783540547938. 

External links

• Overview of contact angle Hysteresis
• Preisach model of hysteresis – Matlab codes developed by Zs. Szabó and Gy. Kádár
• Hysteresis
• What's hysteresis?
• Dynamical systems with hysteresis (interactive web page)
• Magnetization reversal applet (coherent rotation)
• Elastic hysteresis and rubber bands