- Delta-v budget
-
Delta-v budget (or velocity change budget) is an astrogation term used in astrodynamics and aerospace industry for total delta-v (or total velocity change) requirements for the various propulsive tasks and orbital maneuvers over phases of a space mission.
Sample delta-v budget will enumerate various classes of maneuvers, delta-v per maneuver, number of maneuvers required over the time of the mission.
In the absence of an atmosphere, the delta-v is typically the same for changes in orbit in either direction; in particular, gaining and losing speed cost an equal effort.
Because the delta-v needed to achieve the mission usually varies with the relative position of the gravitating bodies, launch windows are often calculated from porkchop plots that show delta-v plotted against the launch time.
Contents
General principles
The rocket equation shows that the delta-v of a rocket is proportional to the logarithm of the mass ratio of the vehicle. Minimising the delta-v budget as far as possible is usually very important to avoid the necessity for infeasibly big and expensive rockets.
The simplest budget can be calculated with Hohmann transfer, which moves from one circular orbit to another coplanar circular orbit via an elliptical transfer orbit. In some cases a bi-elliptic transfer can give a lower delta-v.
A more complex transfer occurs when the orbits are not coplanar. In that case there is an additional delta-v necessary to change the plane of the orbit. The velocity of the vehicle needs substantial burns at the intersection of the two orbital planes and the delta-v is usually extremely high. However, these plane changes can be almost free in some cases if the gravity and mass of a planetary body is used to perform the deflection. In other cases, boosting up to a relatively high altitude apoapsis gives low speed before performing the plane change and this can give lower total delta-v.
The slingshot effect can be used in some cases to give a boost of speed/energy; if a vehicle goes past a planetary or lunar body, it is possible to pick up (or lose) much of that body's orbital speed relative to the Sun or a planet.
Another effect is the Oberth effect - this can be used to greatly decrease the delta-v needed, as using propellant at low potential energy/high speed multiplies the effect of a burn. Thus for example the delta-v for a Hohmann transfer from Earth's orbital radius to Mars' orbital radius (to overcome the Sun's gravity) is many kilometres per second, but the incremental burn from LEO over and above the burn to overcome the Earth's gravity is far less if the burn is done close to the Earth than if the burn to reach a Mars transfer orbit is performed at Earth's orbit, but far away from Earth.
Because the slingshot effect and Oberth effect depend on the position and motion of bodies, the delta-v budget changes with launch time. These can be plotted on a porkchop plot.
Course corrections usually also require some propellant budget. Propulsion systems never provide precisely the right propulsion in precisely the right direction at all times and navigation also introduces some uncertainty. Some propellant needs to be reserved to correct variations from the optimum trajectory.
Budget
Launch/landing
The delta-v requirements for sub-orbital spaceflight can be surprisingly low. For the Ansari X Prize altitude of 100 km, Space Ship One required a delta-v of roughly 1.4 km/s. To reach low earth orbit of the space station of 300 km, the delta-v is over six times higher about 9.4 km/s. Because of the exponential nature of the rocket equation the orbital rocket needs to be considerably bigger.
- Launch to LEO — this not only requires an increase of velocity from 0 to 7.8 km/s, but also typically 1.5–2 km/s for atmospheric drag and gravity drag
- Re-entry from LEO — the delta-v required is the orbital maneuvering burn to lower perigee into the atmosphere, atmospheric drag takes care of the rest.
Stationkeeping
Maneuver Average delta-v per year [m/s] Maximum per year [m/s] Drag compensation in 400–500 km LEO <25 <100 Drag compensation in 500–600 km LEO < 5 < 25 Drag compensation in > 600 km LEO < 7.5 Station-keeping in geostationary orbit 50–55 Station-keeping in L1/L2 30–100 Station-keeping in lunar orbit 0–400 [1] Attitude control (3-axis) 2–6 Spin-up or despin 5–10 Stage booster separation 5–10 Momentum-wheel unloading 2–6 Earth–Moon space
Delta-v needed to move inside Earth–Moon system (speeds lower than escape velocity) are given in km/s units. This table assumes that the Oberth effect is being used, possible with chemical propulsion but not with current electrical propulsion.
The return to LEO figures assume that a heat shield and aerobraking/aerocapture is used to reduce the speed by up to 3.2 km/s. The heat shield increases the mass, possibly by 15%. Where a heat shield is not used the higher from LEO Delta-v figure applies. LEO-Ken refers to a low Earth orbit with an inclination to the equator of 28 degrees, corresponding to a launch from Kennedy Space Center. LEO-Eq is an equatorial orbit.
∆V km/s From\To LEO-Ken LEO-Eq GEO EML-1 EML-2 EML-4/5 LLO Moon C3=0 Earth 9.3 - 10 Low Earth Orbit (LEO-Ken) 4.24 4.33 3.77 3.43 3.97 4.04 5.93 3.22 Low Earth Orbit (LEO-Eq) 4.24 3.90 3.77 3.43 3.99 4.04 5.93 3.22 Geostationary Orbit (GEO) 2.06 1.63 1.38 1.47 1.71 2.05 3.92 1.30 Lagrangian point 1 (EML-1) 0.77 0.77 1.38 0.14 0.33 0.64 2.52 0.14 Lagrangian point 2 (EML-2) 0.33 0.33 1.47 0.14 0.34 0.64 2.52 0.14 Lagrangian point 4/5 (EML-4/5) 0.84 0.98 1.71 0.33 0.34 0.98 2.58 0.43 Low Lunar orbit (LLO) 1.31 1.31 2.05 0.64 0.65 0.98 1.87 1.40 Moon (Moon) 2.74 2.74 3.92 2.52 2.53 2.58 1.87 2.80 Earth Escape velocity (C3=0) 0.00 0.00 1.30 0.14 0.14 0.43 1.40 2.80 Interplanetary
The spacecraft is assumed to be using chemical propulsion and the Oberth Effect.
From To delta-v in km/s LEO Mars Transfer Orbit 4.3 [5] Earth Escape velocity (C3=0) Mars Transfer Orbit 0.6 [6] Mars Transfer Orbit Mars Capture Orbit 0.9 [6] Mars Capture Orbit Deimos Transfer Orbit 0.2 [6] Deimos Transfer Orbit Deimos surface 0.7 [6] Deimos Transfer Orbit Phobos Transfer Orbit 0.3 [6] Phobos Transfer Orbit Phobos surface 0.5 [6] Mars Capture Orbit Low Mars Orbit 1.4 [6] Low Mars Orbit Mars surface 4.1 [6] EML2 Mars Transfer Orbit <1.0 [5] Mars Transfer Orbit Low Mars Orbit 2.7 [5] Earth Escape velocity (C3=0) Closest NEO Asteroids[7] 0.8 - 2.0 According to Marsden and Ross, "The energy levels of the Sun-Earth L1 and L2 points differ from those of the Earth-Moon system by only 50 m/s (as measured by maneuver velocity)."[8]
Delta-vs between Earth, Moon and Mars
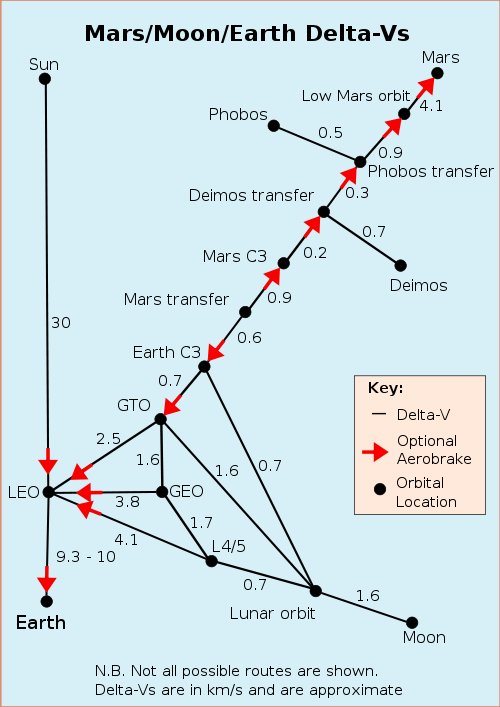 Delta-v needed for various orbital manoeuvers using conventional rockets.[6][9] Red arrows show where optional aerobraking can be performed in that particular direction, black numbers give delta-v in km/s that apply in either direction. Lower delta-v transfers than shown can often be achieved, but involve rare transfer windows or take significantly longer, see: fuzzy orbital transfers. Electric propulsion vehicles going from Mars C3=0 to Earth C3=0 without using the Oberth Effect need a larger deltaV of between 2.6km/s and 3.15km/s.[10] Not all possible links are shown.
Delta-v needed for various orbital manoeuvers using conventional rockets.[6][9] Red arrows show where optional aerobraking can be performed in that particular direction, black numbers give delta-v in km/s that apply in either direction. Lower delta-v transfers than shown can often be achieved, but involve rare transfer windows or take significantly longer, see: fuzzy orbital transfers. Electric propulsion vehicles going from Mars C3=0 to Earth C3=0 without using the Oberth Effect need a larger deltaV of between 2.6km/s and 3.15km/s.[10] Not all possible links are shown.
Abbrevations key: Escape orbit (C3), Geosynchronous orbit (GEO), Geostationary transfer orbit (GTO), Earth–Moon L5 Lagrangian point (L5), Low Earth orbit (LEO)Near earth object
Near earth objects are asteroids that are within the orbit of Mars. The delta-v to return from them are usually quite small, sometimes as low as 60m/s, using aerobraking on Earths atmosphere (substantial reentry shields would be required).[11] The orbital phasing can be problematic; once rendezvous has been achieved, low delta-v return windows can be fairly far apart (more than a year, often many years), depending on the body.
However, the delta-v to reach them is usually rather higher, over 3.8 km/s,[11] which is still less than the delta-v to reach the moon's surface.
In general bodies that are much further away or closer to the Sun than the Earth have more frequent windows for travel, but usually have larger delta-v's.
See also
- Bi-elliptic transfer
- Gravity assist
- Hohmann transfer
- The Oberth effect
- Tsiolkovsky rocket equation
- Pork-chop plot
- Synodic period
References
- ^ Frozen lunar orbits
- ^ list of delta-v
- ^ L2 Halo lunar orbit
- ^ Strategic Considerations for Cislunar Space Infrastructure
- ^ a b c Zegler and Kutter (AIAA 2010-8638)
- ^ a b c d e f g h i Rockets and Space Transportation[dead link]. See: Atomic Rocket: Missions
- ^ NEO list
- ^ "New methods in celestial mechanics and mission design". Bull. Amer. Math. Soc.. http://www.ams.org/bull/2006-43-01/S0273-0979-05-01085-2/home.html.
- ^ cislunar delta-vs
- ^ ""Ion Propulsion for a Mars Sample Return Mission" John R. Brophy and David H. Rodgers, AIAA-200-3412, Table 1". http://trs-new.jpl.nasa.gov/dspace/bitstream/2014/15789/1/00-1530.pdf.
- ^ a b [1]
External links
Categories:- Celestial mechanics
- Orbits
- Astrodynamics
- Spacecraft propulsion
Wikimedia Foundation. 2010.













