Runcinated 24-cell

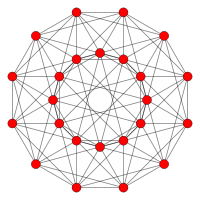
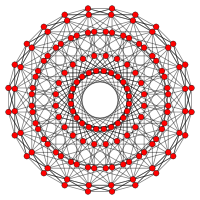
24-cell	Runcinated 24-cell
Runcitruncated 24-cell	Omnitruncated 24-cell (Runcicantitruncated 24-cell)
Orthogonal projections in F4 Coxeter plane

In four-dimensional geometry, a runcinated 24-cell is a convex uniform polychoron, being a runcination (a 3rd order truncation) of the regular 24-cell.

There are 3 unique degrees of runcinations of the 24-cell including with permutations truncations and cantellations.

Runcinated 24-cell

Runcinated 24-cell
Type	Uniform polychoron
Schläfli symbol	t0,3{3,4,3}
Coxeter-Dynkin diagram
Cells	240	48 3.3.3.3 192 3.4.4
Faces	672	384{3} 288{4}
Edges	576
Vertices	144
Vertex figure	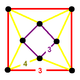elongated square antiprism
Symmetry group	F4, [[3,4,3]], order 2304
Properties	convex, edge-transitive
Uniform index	25 26 27

In geometry, the runcinated 24-cell is a uniform polychoron bounded by 48 octahedra and 192 triangular prisms. The octahedral cells correspond with the cells of a 24-cell and its dual.

Coordinates

The Cartesian coordinates of the runcinated 24-cell having edge length 2 is given by all permutations of sign and coordinates of:

(0, 0, √2, 2+√2)

(1, 1, 1+√2, 1+√2)

The permutations of the second set of coordinates coincide with the vertices of an inscribed cantellated tesseract.

Projections

orthographic projections

Coxeter plane	F4	B4
Graph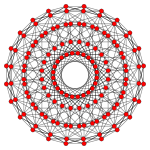
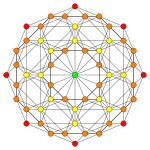
Dihedral symmetry	[[12]]	[8]
Coxeter plane	B3 / A2	B2 / A3
Graph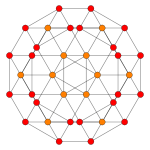
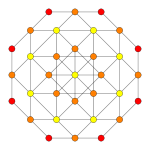
Dihedral symmetry	[6]	[[4]]

3D perspective projections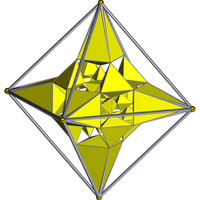
Schlegel diagram, centered on octahedron, with the octahedra shown.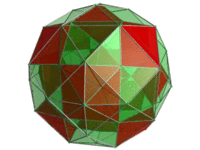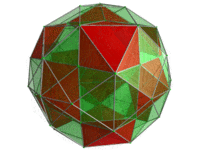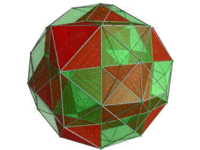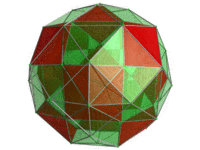	Perspective projection of the runcinated 24-cell into 3 dimensions, centered on an octahedral cell. The rotation is only of the 3D image, in order to show its structure, not a rotation in 4-space. Fifteen of the octahedral cells facing the 4D viewpoint are shown here in red. The gaps between them are filled up by a framework of triangular prisms.	Stereographic projection with 24 of its 48 octahedral cells

Related regular skew polyhedron

The regular skew polyhedron, {4,8|3}, exists in 4-space with 8 square around each vertex, in a zig-zagging nonplanar vertex figure. These square faces can be seen on the runcinated 24-cell, using all 576 edges and 288 vertices. The 384 triangular faces of the runcinated 24-cell can be seen as removed. The dual regular skew polyhedron, {8,3|3}, is similarly related to the octagonal faces of the bitruncated 24-cell.

Runcitruncated 24-cell

Runcitruncated 24-cell
Type	Uniform polychoron
Schläfli symbol	t0,1,3{3,4,3}
Coxeter-Dynkin diagram
Cells	240	24 4.6.6 96 4.4.6 96 3.4.4 24 3.4.4.4
Faces	1104	192{3} 720{4} 192{6}
Edges	1440
Vertices	576
Vertex figure	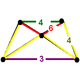Trapezoidal pyramid
Symmetry group	F4, [3,4,3]
Properties	convex
Uniform index	28 29 30

The runcitruncated 24-cell is a uniform polychoron derived from the 24-cell. It is bounded by 24 truncated octahedra, corresponding with the cells of a 24-cell, 24 rhombicuboctahedra, corresponding with the cells of the dual 24-cell, 96 triangular prisms, and 96 hexagonal prisms.

Coordinates

The Cartesian coordinates of an origin-centered runcitruncated 24-cell having edge length 2 are given by all permutations of coordinates and sign of:

(0, √2, 2√2, 2+3√2)

(1, 1+√2, 1+2√2, 1+3√2)

The permutations of the second set of coordinates give the vertices of an inscribed omnitruncated tesseract.

The dual configuration has coordinates generated from all permutations and signs of:

(1,1,1+√2,5+√2)

(1,3,3+√2,3+√2)

(2,2,2+√2,4+√2)

Projections

orthographic projections

Coxeter plane	F4
Graph
Dihedral symmetry	[12]
Coxeter plane	B3 / A2 (a)	B3 / A2 (b)
Graph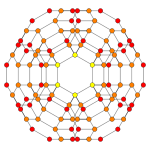
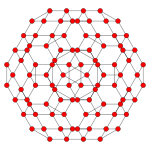
Dihedral symmetry	[6]	[6]
Coxeter plane	B4	B2 / A2
Graph
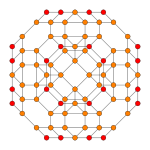
Dihedral symmetry	[8]	[4]

Schlegel diagram centered on rhombicuboctahedron only triangular prisms shown

Omnitruncated 24-cell

Omnitruncated 24-cell
Type	Uniform polychoron
Schläfli symbol	t0,1,2,3{3,4,3}
Coxeter-Dynkin diagram
Cells	240	48 (4.6.8) 192 (4.4.6)
Faces	1392	864{4} 384{6} 144{8}
Edges	2304
Vertices	1152
Vertex figure	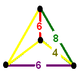Irreg. tetrahedron
Symmetry group	F4, [[3,4,3]], order 2304
Properties	convex
Uniform index	29 30 31

The omnitruncated 24-cell is a uniform polychoron derived from the 24-cell. It is composed of 1152 vertices, 2304 edges, and 1392 faces (864 squares, 384 hexagons, and 144 octagons). It has 240 cells: 48 great rhombicuboctahedra, 192 hexagonal prisms. Each vertex contains four cells in an irregular tetrahedral vertex figure: two hexagonal prisms, and two truncated cuboctahedra.

Structure

The 48 great rhombicuboctahedral cells are joined to each other via their octagonal faces. They can be grouped into two groups of 24 each, corresponding with the cells of a 24-cell and its dual. The gaps between them are filled in by a network of 192 hexagonal prisms, joined to each other via alternating square faces in alternating orientation, and to the great rhombicuboctahedra via their hexagonal faces and remaining square faces.

Coordinates

The Cartesian coordinates of an omnitruncated 24-cell having edge length 2 are all permutations of coordinates and sign of:

(1, 1+√2, 1+2√2, 5+3√2)

(1, 3+√2, 3+2√2, 3+3√2)

(2, 2+√2, 2+2√2, 4+3√2)

Projections

orthographic projections

Coxeter plane	F4	B4
Graph
Dihedral symmetry	[[12]]	[8]
Coxeter plane	B3 / A2	B2 / A3
Graph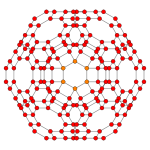
Dihedral symmetry	[6]	[[4]]

3D perspective projections

Schlegel diagram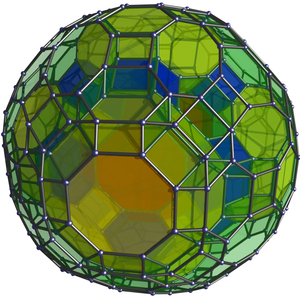

Perspective projection into 3D centered on a great rhombicuboctahedron. The nearest great rhombicuboctahedral cell to the 4D viewpoint is shown in red, with the six surrounding great rhombicuboctahedra in yellow. Twelve of the hexagonal prisms sharing a square face with the nearest cell and hexagonal faces with the yellow cells are shown in blue. The remaining cells are shown in green. Cells lying on the far side of the polytope from the 4D viewpoint have been culled for clarity.

Related polytopes

Name	24-cell	truncated 24-cell	rectified 24-cell	cantellated 24-cell	bitruncated 24-cell	cantitruncated 24-cell	runcinated 24-cell	runcitruncated 24-cell	omnitruncated 24-cell	snub 24-cell
Schläfli symbol	{3,4,3}	t0,1{3,4,3}	t1{3,4,3}	t0,2{3,4,3}	t1,2{3,4,3}	t0,1,2{3,4,3}	t0,3{3,4,3}	t0,1,3{3,4,3}	t0,1,2,3{3,4,3}	h0,1{3,4,3}
Coxeter-Dynkin diagram
Schlegel diagram
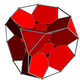
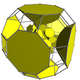
F4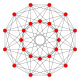
B4
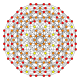
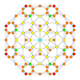
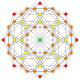
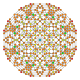
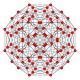
B3(a)
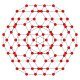
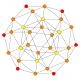
B3(b)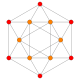
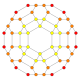
B2

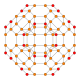
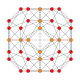
References

• Kaleidoscopes: Selected Writings of H.S.M. Coxeter, editied by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6 [1]
  • (Paper 22) H.S.M. Coxeter, Regular and Semi-Regular Polytopes I, [Math. Zeit. 46 (1940) 380-407, MR 2,10]
  • (Paper 23) H.S.M. Coxeter, Regular and Semi-Regular Polytopes II, [Math. Zeit. 188 (1985) 559-591]
  • (Paper 24) H.S.M. Coxeter, Regular and Semi-Regular Polytopes III, [Math. Zeit. 200 (1988) 3-45]
• J.H. Conway and M.J.T. Guy: Four-Dimensional Archimedean Polytopes, Proceedings of the Colloquium on Convexity at Copenhagen, page 38 und 39, 1965
• N.W. Johnson: The Theory of Uniform Polytopes and Honeycombs, Ph.D. Dissertation, University of Toronto, 1966
• Four-dimensional Archimedean Polytopes (German), Marco Möller, 2004 PhD dissertation [2] m58 m59 m53
• 3. Convex uniform polychora based on the icositetrachoron (24-cell), George Olshevsky.
• Richard Klitzing, 4D, uniform polytopes (polychora) x3o4o3x - spic, x3x4o3x - prico, x3x4x3x - gippic