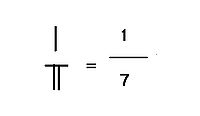Counting rods

Yang Hui (Pascal's) triangle, as depicted by Zhu Shijie in 1303, using rod numerals.

Numeral systems by culture
Hindu-Arabic numerals
Western Arabic (Hindu numerals) Eastern Arabic Indian family Tamil	Burmese Khmer Lao Mongolian Thai
East Asian numerals
Chinese Japanese Suzhou	Korean Vietnamese Counting rods
Alphabetic numerals
Abjad Armenian Āryabhaṭa Cyrillic	Ge'ez Greek (Ionian) Hebrew
Other systems
Aegean Attic Babylonian Brahmi Egyptian Etruscan Inuit	Kharosthi Mayan Quipu Roman Sumerian Urnfield
List of numeral system topics
Positional systems by base
Decimal (10)
2, 3, 4, 5, 6, 7, 8, 9, 11, 12, 13, 14, 15, 16, 20, 24, 30, 36, 60, 64
List of numeral systems
v · d · e

Counting rods (simplified Chinese: 筹; traditional Chinese: 籌; pinyin: chóu; Japanese: 算木, sangi) are small bars, typically 3–14 cm long, used by mathematicians for calculation in China, Japan, Korea, and Vietnam. They are placed either horizontally or vertically to represent any number and any fraction.

The written forms based on them are called rod numerals. They are a true positional numeral system with digits for 1-9 and a blank for 0 , since Warring states to 16th century.

History

Counting rods were used by ancient Chinese for more than two thousand years. In 1954, forty-odd counting rods of the Warring States Period were found in Zuǒjiāgōngshān (左家公山) Chǔ Grave No.15 in Changsha, Hunan.^[1][2].

In 1973, archeologists unearthed a number of wood scripts from a Han dynasty tomb in Hubei, one of the wooden script written:“当利二月定算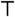”，this is one of the earliest examples of using counting rod numeral in writing.

In 1976, a bundle of West Han counting rods made of bones in was unearthed from Qian yang county in Shanxi^[3] The use of counting rods must predate it; the Laozi, a text originating from the Warring States, said "a good calculator doesn't use counting rods."^[4] The Book of Han recorded: "they calculate with bamboo, diameter one fen, length six cun, arranged into a hexagonal bundle of two hundred seventy one pieces."

After the abacus flourished, counting rods were abandoned except in Japan, where rod numerals developed into symbolic notation for algebra.

Using counting rods

rod numeral place value from Yongle Encyclopedia

Japanese counting board with grids

A checker counting board diagram in a 18th century Japanese mathematics textbook

counting rod numerals in grids in a Japanese mathematic book

Counting rods represent digits by the number of rods, and the perpendicular rod represents five. To avoid confusion, vertical and horizontal forms are alternately used. Generally, vertical rod numbers are used for the position for the units, hundreds, ten thousands, etc., while horizontal rod numbers are used for the tens, thousands, hundred thousands etc. Sun Tzu wrote that "one is vertical, ten is horizontal."^[5]

Red rods represent positive numbers and black rods represent negative numbers. Ancient Chinese clearly understood negative numbers and zero (leaving a blank space for it), though they had no symbol for the latter. The Nine Chapters on the Mathematical Art, which was mainly composed in the first century CE, stated "(when using subtraction) subtract same signed numbers, add different signed numbers, subtract a positive number from zero to make a negative number, and subtract a negative number from zero to make a positive number."^[6][7] Later, a go stone was sometimes used to represent 0.

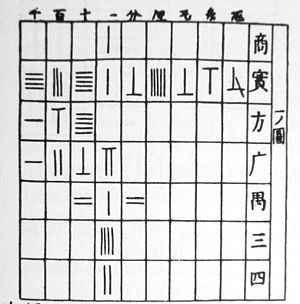
This alternation of vertical and horizontal rod numeral form is very important to correctly understand written trascription of rod numerals on manuscripts. For instance, in Licheng suanjin, 81 was transribed as , and 108 was transribed as ; it is clear that the latter clearly had a blank zero on the "counting board" (ie, floor or mat), even though on the written transcription, there was no blank. In the same manuscript, 405 was transcribed as , with a blank space in between for obvious reasons, and could in no way be interpreted as "45". In other words, transcribed rod numerals may not be positional, but on the counting board, they are positional. is an exact image of the counting rod number 405 on a table top or floor.

Place value

The value of a number depends on its physical position on the counting board; a 9 at the rightmost position on the board stands for 9, move the batch of rods representing 9 to the left one position (i.e., the ten position) = 9blanck =90, shift left three position = 9[][] = 900; and so on. Similariy, move a number right each rank tantamount to divide the number by 10. This applies to single-digit number or multiple digit number.

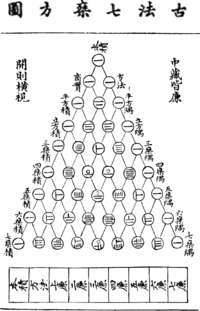
Song dynasty mathematician Jia Xian used hand-written Chinese decimal orders 步十百千万 as rod numeral place value, as evident from a facsimile from a page of Yongle Encyclopedia. He arranged 七万一千八百二十四 as

七一八二四

万千百十步

He treated the Chinese order numbers as place value markers, and 七一八二四 became place value decimal number. He then wrote the rod numerals according to their place value:

七	一	八	二	四
万	千	百	十	步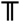

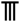
In Japan, mathematicians put counting rods on a counting board, a sheet of cloth with grids, and used only vertical forms relying on the grids. An 18th-century Japanese mathematics book has a checker counting board diagram, with the order of magnitude symbols "千百十一分厘毛“（myriad, hundred, ten, unit, one tenth, etc)^[8]

Positive numbers

	0	1	2	3	4	5	6	7	8	9
Vertical
Horizontal

Negative numbers

	0	-1	-2	-3	-4	-5	-6	-7	-8	-9
Vertical
Horizontal

^{[citation needed]}

Examples:

231
5089
-407
-6720

Rod numerals

Rod numerals are a positional numeral system made from shapes of counting rods. Positive numbers are written as they are and the negative numbers are written with a slant bar at the last digit. The vertical bar in the horizontal forms 6-9 is drawn shorter to have the same character height.

A circle (〇) is used for 0. Many historians think it was imported from Indian numerals by Gautama Siddha in 718^[6], but some think it was created from the Chinese text space filler "□"^[9].

In the 13th century, Southern Song mathematicians changed digits for 4, 5, and 9 to reduce strokes^[9]. The new horizontal forms eventually transformed into Suzhou numerals. Japanese continued to use the traditional forms.

Positive numbers (traditional)

	0	1	2	3	4	5	6	7	8	9
Vertical
Horizontal

Negative numbers (traditional)

	-0	-1	-2	-3	-4	-5	-6	-7	-8	-9
Vertical

Positive numbers (Southern Song)

	0	1	2	3	4	5	6	7	8	9
Vertical
Horizontal

Examples:

	Traditional	Southern Song
231
5089
-407
-6720

In Japan, Seki Takakazu developed the rod numerals into symbolic notation for algebra and drastically improved Japanese mathematics^[6]. After his period, the positional numeral system using Chinese numeral characters was invented, and the rod numerals worked only as the plus and minus signs.

Western	Seki	After Seki
x + y + 246	甲乙	甲乙二四六
5x - 6y	甲乙	五甲六乙
7xy	甲乙 d	七甲乙
8x / y	N/A	乙八甲

Fractions

Fraction 1/7

A fraction was expressed with rod numerals as two rod numerals one on top of another (without any other symbol, like the modern horizontal bar).

Rod calculus

Main article: Rod calculus

The method for using counting rods for mathematical calculation was called rod calculation or rod calculus (筹算‎). Rod calculus can be used for a wide range of calculations, including finding the value of π, finding square roots, cube roots, or higher order roots, and solving a system of linear equations. As a result, the character 籌 is extended to connote the concept of planning in Chinese. For example, the science of using counting rods 運籌學 does not refer to counting rods; it means operational research.

Before the introduction of written zero, there was no way to separate 10007 and 107 in written forms except by inserting a bigger space between 1 and 7, and so rod numerals were used only for doing calculations with counting rods. Once written zero came into play, the rod numerals had become independent, and their use indeed outlives the counting rods, after its replacement by abacus One variation of horizontal rod numerals, the Suzhou numerals is still in use for book-keeping and in herbal medicine prescription in Chinatowns in some parts of the world.

Counting rods in Unicode

Unicode 5.0 includes counting rod numerals in their own block in the Supplementary Multilingual Plane (SMP) from U+1D360 to U+1D37F. The code points for the horizontal digits 1-9 are U+1D360 to U+1D368 and those for the vertical digits 1-9 are U+1D369 to U+1D371. The former are called unit digits and the latter are called tens digits^[10], which is opposite of the convention described above. Zero should be represented by U+3007 (〇, ideographic number zero) and the negative sign should be represented by U+20E5 (combining reverse solidus overlay)^[11]. As these were recently added to the character set and since they are included in the SMP, font support may still be limited. Grey areas indicate non-assigned code points.

Counting Rod Numerals[1] Unicode.org chart (PDF)
	0	1	2	3	4	5	6	7	8	9	A	B	C	D	E	F
U+1D36x	Wikimedia Foundation. 2010. Игры ⚽ Нужно решить контрольную? Craster Cartmel Priory Look at other dictionaries: Prehistoric numerals — Counting in prehistory was first assisted by using body parts, most notably the fingers. This is reflected in the etymology of certain number names, notably in the names of ten and hundred in the Proto Indo European numerals, both… …   Wikipedia Chinese numerals — Numeral systems by culture Hindu Arabic numerals Western Arabic (Hindu numerals) Eastern Arabic Indian family Tamil Burmese Khmer Lao Mongolian Thai East Asian numerals Chinese Japanese Suzhou Korean …   Wikipedia mathematics, East Asian — Introduction       the discipline of mathematics as it developed in China and Japan.       When speaking of mathematics in East Asia, it is necessary to take into account China, Japan, Korea, and Vietnam as a whole. At a very early time in their… …   Universalium Chinese mathematics — Mathematics in China emerged independently by the 11th century BC.[1] The Chinese independently developed very large and negative numbers, decimals, a place value decimal system, a binary system, algebra, geometry, and trigonometry. Many[who?]… …   Wikipedia Rod calculus — or rod calculation is the method of mathematical computation with counting rods in China from The Warring States to Ming dynasty before the counting rods were replaced by more convenient and faster abacus.HardwareThe basic equipment for carrying… …   Wikipedia Suanpan — (the number represented in the picture is 6,302,715,408) Chinese Abacus …   Wikipedia Numerical digit — The ten digits of the Arabic numerals, in order of value. A digit is a symbol (a numeral symbol such as 3 or 7 ) used in combinations (such as 37 ) to represent numbers in positional numeral systems. The name digit comes from the fact that the 10 …   Wikipedia Unicode numerals — Numerals (often called numbers in Unicode) are characters that denote a number. The same Arabic Indic numerals are used widely in various writing systems throughout the world and all share the same semantics for denoting numbers, However, the… …   Wikipedia Positional notation — Numeral systems by culture Hindu Arabic numerals Western Arabic (Hindu numerals) Eastern Arabic Indian family Tamil Burmese Khmer Lao Mongolian Thai East Asian numerals Chinese Japanese Suzhou Korean Vietnamese …   Wikipedia Hindu–Arabic numeral system — Numeral systems by culture Hindu Arabic numerals Western Arabic (Hindu numerals) Eastern Arabic Indian family Tamil Burmese Khmer Lao Mongolian Thai East Asian numerals Chinese Japanese Suzhou Korean Vietna …   Wikipedia 18+ © Academic, 2000-2024 Contact us: Technical Support, Advertising Dictionaries export, created on PHP, Joomla, Drupal, WordPress, MODx. Mark and share Search through all dictionaries Translate… Search Internet Share the article and excerpts Direct link … Do a right-click on the link above and select “Copy Link”