- Grand antiprism
-
Grand antiprism 
(Schlegel diagram wireframe)Type Uniform polychoron Uniform index 47 Cells 100+200 (3.3.3) 
20 (3.3.3.5)
Faces 20 {5}
700 {3}Edges 500 Vertices 100 Vertex figure 12 (3.3.3)
2 (3.3.3.5)
Symmetry group Ionic diminished Coxeter group [[10,2+,10]], of order 400 Schläfli symbol s{5}.s{5} (extended) Properties convex In geometry, the grand antiprism or pentagonal double antiprismoid is a uniform polychoron (4-dimensional uniform polytope) bounded by 320 cells: 20 pentagonal antiprisms, and 300 tetrahedra. It is an anomalous, non-Wythoffian uniform polychoron, discovered in 1965 by Conway and Guy.[1][2]
Contents
Alternate names
- Pentagonal double antiprismoid Norman W. Johnson
- Gap (Jonathan Bowers: for grand antiprism)
Structure
The 20 pentagonal antiprisms occur in two disjoint rings of 10 antiprisms each. The antiprisms in each ring are joined to each other via their pentagonal faces. The two rings are mutually perpendicular, in a structure similar to a duoprism.
The 300 tetrahedra join the two rings to each other, and are laid out in a 2-dimensional arrangement topologically equivalent to the 2-torus and the ridge of the duocylinder.
This structure is analogous to that of the 3-dimensional antiprisms. However, the grand antiprism is the only uniform analogue of the antiprism in 4 dimensions (although the 16-cell may be regarded as a regular analogue of the digonal antiprism).
Vertex figure
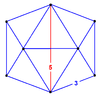
The vertex figure of the grand antiprism is a dissected regular icosahedron: a regular icosahedron with two vertices removed. In their place 8 triangles are replaced by a pair of trapezoids, edge lengths φ, 1, 1, 1 (where φ is the golden ratio), joined together along their edge of length φ, to give a tetradecahedron whose faces are the 2 trapezoids and the 12 remaining equilateral triangles.

12 (3.3.3)
2 (3.3.3.5)
Dissected regular icosahedronConstruction
The grand antiprism can be constructed by diminishing the 600-cell: subtracting 20 pyramids whose bases are three-dimensional pentagonal antiprisms. Conversely, the two rings of pentagonal antiprisms in the grand antiprism may be triangulated by 10 tetrahedra joined to the triangular faces of each antiprism, and a circle of 5 tetrahedra between every pair of antiprisms, joining the 10 tetrahedra of each, yielding 150 tetrahedra per ring. These combined with the 300 tetrahedra that join the two rings together yield the 600 tetrahedra of the 600-cell.
This relationship is analogous to how a pentagonal antiprism can be constructed from an icosahedron by removing two opposite vertices, thereby removing 5 triangles from the opposite 'poles' of the icosahedron, leaving the 10 equatorial triangles and two pentagons on the top and bottom.
Computationally speaking, this diminishing may be realized by removing two rings of vertices from the 600-cell, each ring having 10 vertices, and each lying in mutually orthogonal planes, and taking the convex hull of the remaining vertices.
(The snub 24-cell can also be constructed by another diminishing of the 600-cell, removing 24 icosahedral pyramids. Equivalently, this may be realized as taking the convex hull of the vertices remaining after 24 vertices, corresponding to those of an inscribed 24-cell, are removed from the 600-cell.)
Projections
These are two perspective projections, projecting the polytope into a hypersphere, and applying a stereographic projection into 3-space.
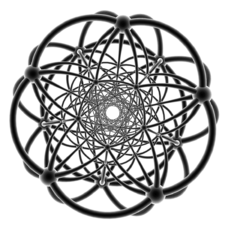
Wireframe, viewed down one of the pentagonal antiprism columns.
with transparent triangular faces
Orthographic projection
Centered on hyperplane of an antiprism in one of the two rings.See also
Notes
- ^ J.H. Conway and M. J. T. Guy: Four-Dimensional Archimedean Polytopes, Proceedings of the Colloquium on Convexity at Copenhagen, page 38 und 39, 1965. (Michael Guy is son of Richard K. Guy)
- ^ Conway, 2008, p.402-403 The Grand Antiprism
References
- Kaleidoscopes: Selected Writings of H.S.M. Coxeter, editied by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6 [1]
- (Paper 23) H.S.M. Coxeter, Regular and Semi-Regular Polytopes II, [Math. Zeit. 188 (1985) 559-591] 2.8 The Grand Antiprism
- Anomalous convex uniform polychoron - Model 47, George Olshevsky.
- Richard Klitzing, 4D uniform polytopes (polychora), gap
- John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, The Symmetries of Things 2008, ISBN 978-1-56881-220-5 (Chapter 26) The Grand Antiprism
External links
- In the Belly of the Grand Antiprism (middle section, describing the analogy with the icosahedron and the pentagonal antiprism)
Fundamental convex regular and uniform polytopes in dimensions 2–10 Family An BCn Dn E6 / E7 / E8 / F4 / G2 Hn Regular polygon Triangle Square Hexagon Pentagon Uniform polyhedron Tetrahedron Octahedron • Cube Demicube Dodecahedron • Icosahedron Uniform polychoron 5-cell 16-cell • Tesseract Demitesseract 24-cell 120-cell • 600-cell Uniform 5-polytope 5-simplex 5-orthoplex • 5-cube 5-demicube Uniform 6-polytope 6-simplex 6-orthoplex • 6-cube 6-demicube 122 • 221 Uniform 7-polytope 7-simplex 7-orthoplex • 7-cube 7-demicube 132 • 231 • 321 Uniform 8-polytope 8-simplex 8-orthoplex • 8-cube 8-demicube 142 • 241 • 421 Uniform 9-polytope 9-simplex 9-orthoplex • 9-cube 9-demicube Uniform 10-polytope 10-simplex 10-orthoplex • 10-cube 10-demicube n-polytopes n-simplex n-orthoplex • n-cube n-demicube 1k2 • 2k1 • k21 pentagonal polytope Topics: Polytope families • Regular polytope • List of regular polytopes Categories:- Four-dimensional geometry
- Polychora
Wikimedia Foundation. 2010.

