- Duoprism
-
Set of uniform p,q-duoprisms
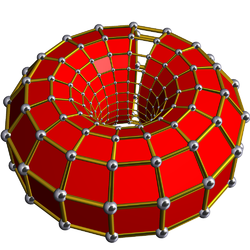
Example 16,16-duoprism
Schlegel diagram
Projection from the center of one 16-gonal prism, and all but one of the opposite 16-gonal prisms are shown.Type Prismatic uniform polychoron Schläfli symbol {p}x{q} Coxeter-Dynkin diagram 






Cells p q-gonal prisms,
q p-gonal prismsFaces pq squares,
p q-gons,
q p-gonsEdges 2pq Vertices pq Vertex figure 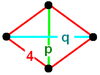
disphenoid tetrahedronSymmetry [p,2,q], order 4pq
[[p,2,p]], order 8p2, p=qDual Duopyramid Properties convex if both bases are convex In geometry of 4 dimensions or higher, a duoprism is a polytope resulting from the Cartesian product of two polytopes, each of two dimensions or higher. The Cartesian product of an n-polytope and an m-polytope is an (n+m)-polytope, where n and m are 2 (polygon) or higher.
The lowest dimensional duoprisms exist in 4-dimensional space as polychora (4-polytopes) being the Cartesian product of two polygons in 2-dimensional Euclidean space. More precisely, it is the set of points:
where P1 and P2 are the sets of the points contained in the respective polygons. Such a duoprism is convex if both bases are convex, and is bounded by prismatic cells.
Contents
Nomenclature
Four-dimensional duoprisms are considered to be prismatic polychora. A duoprism constructed from two regular polygons of the same size is a uniform duoprism.
A duoprism made of n-polygons and m-polygons is named by prefixing 'duoprism' with the names of the base polygons, for example: a triangular-pentagonal duoprism is the Cartesian product of a triangle and a pentagon.
An alternative, more concise way of specifying a particular duoprism is by prefixing with numbers denoting the base polygons, for example: 3,5-duoprism for the triangular-pentagonal duoprism.
Other alternative names:
- q-gonal-p-gonal prism
- q-gonal-p-gonal double prism
- q-gonal-p-gonal hyperprism
The term duoprism is coined by George Olshevsky, shortened from double prism. Conway proposed a similar name proprism for product prism.
Geometry of 4-dimensional duoprisms
 A close up inside the 23-29 duoprism projected onto a 3-sphere, and perspective projected to 3-space. As m and n become large, a duoprism approaches the geometry of duocylinder just like a p-gonal prism approaches a cylinder.
A close up inside the 23-29 duoprism projected onto a 3-sphere, and perspective projected to 3-space. As m and n become large, a duoprism approaches the geometry of duocylinder just like a p-gonal prism approaches a cylinder.
A 4-dimensional uniform duoprism is created by the product of a regular n-sided polygon and a regular m-sided polygon with the same edge length. It is bounded by n m-gonal prisms and m n-gonal prisms. For example, the Cartesian product of a triangle and a hexagon is a duoprism bounded by 6 triangular prisms and 3 hexagonal prisms.
- When m and n are identical, the resulting duoprism is bounded by 2n identical n-gonal prisms. For example, the Cartesian product of two triangles is a duoprism bounded by 6 triangular prisms.
- When m and n are identically 4, the resulting duoprism is bounded by 8 square prisms (cubes), and is identical to the tesseract.
The m-gonal prisms are attached to each other via their m-gonal faces, and form a closed loop. Similarly, the n-gonal prisms are attached to each other via their n-gonal faces, and form a second loop perpendicular to the first. These two loops are attached to each other via their square faces, and are mutually perpendicular.
As m and n approach infinity, the corresponding duoprisms approach the duocylinder. As such, duoprisms are useful as non-quadric approximations of the duocylinder.
Polychoral duoantiprisms
Like the antiprisms as alternated prisms, there is a set of 4-dimensional duoantiprisms polychorons that can be created by an alternation operation applied to a duoprism. However most are not uniform. The alternated vertices create nonregular tetrahedral cells, except for the special case, the 4-4 duoprism (tesseract) which creates the uniform (and regular) 16-cell. The 16-cell is the only convex uniform duoantiprism.
See also grand antiprism.
Images of uniform polychoral duoprisms
All of these images are Schlegel diagrams with one cell shown. The p-q duoprisms are identical to the q-p duoprisms, but look different because they are projected in the center of different cells.
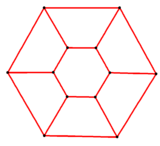
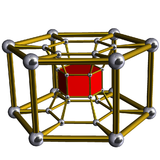
6-prism 6-6-duoprism A hexagonal prism, projected into the plane by perspective, centered on a hexagonal face, looks like a double hexagon connected by (distorted) squares. Similarly a 6-6 duoprism projected into 3D approximates a torus, hexagonal both in plan and in section. 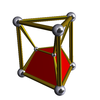
3-3
3-4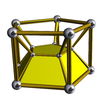
3-5
3-6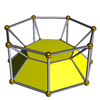
3-7
3-8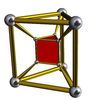
4-3
4-4
4-5
4-6
4-7
4-8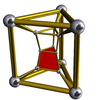
5-3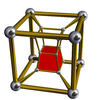
5-4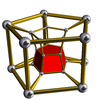
5-5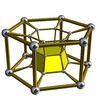
5-6
5-7
5-8
6-3
6-4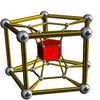
6-5
6-6
6-7
6-8
7-3
7-4
7-5
7-6
7-7
7-8
8-3
8-4
8-5
8-6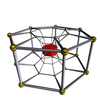
8-7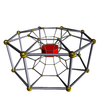
8-8Related polytopes
 A stereographic projection of a rotating duocylinder, divided into a checkerboard surface of squares from the {4,4|n} skew polyhedron
A stereographic projection of a rotating duocylinder, divided into a checkerboard surface of squares from the {4,4|n} skew polyhedronThe regular skew polyhedron, {4,4|n}, exists in 4-space as the n2 square faces of a n-n duoprism, using all 2n2 edges and n2 vertices. The 2n n-gonal faces can be seen as removed. (skew polyhedra can be seen in the same way by a n-m duoprism, but these are not regular.)
See also
- Polytope and polychoron
- Convex regular polychoron
- Duocylinder
- Tesseract
References
- Regular Polytopes, H. S. M. Coxeter, Dover Publications, Inc., 1973, New York, p. 124.
- Coxeter, The Beauty of Geometry: Twelve Essays, Dover Publications, 1999, ISBN 0486409198 (Chapter 5: Regular Skew Polyhedra in three and four dimensions and their topological analogues)
- Coxeter, H. S. M. Regular Skew Polyhedra in Three and Four Dimensions. Proc. London Math. Soc. 43, 33-62, 1937.
- The Fourth Dimension Simply Explained, Henry P. Manning, Munn & Company, 1910, New York. Available from the University of Virginia library. Also accessible online: The Fourth Dimension Simply Explained—contains a description of duoprisms (double prisms) and duocylinders (double cylinders). Googlebook
- John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, The Symmetries of Things 2008, ISBN 978-1-56881-220-5 (Chapter 26)
- Norman Johnson Uniform Polytopes, Manuscript (1991)
- N.W. Johnson: The Theory of Uniform Polytopes and Honeycombs, Ph.D. Dissertation, University of Toronto, 1966
- Olshevsky, George, Duoprism at Glossary for Hyperspace.
- Olshevsky, George, Cartesian product at Glossary for Hyperspace.
- Catalogue of Convex Polychora, section 6, George Olshevsky.
External links
- The Fourth Dimension Simply Explained—describes duoprisms as "double prisms" and duocylinders as "double cylinders"
- Polygloss - glossary of higher-dimensional terms
- Exploring Hyperspace with the Geometric Product
Categories:- Four-dimensional geometry
- Algebraic topology
- Polychora
Wikimedia Foundation. 2010.


