Comma (music)

Syntonic comma on C  Play (help·info).

Pythagorean comma on C  Play (help·info).

In music theory, a comma is a minute interval, the difference resulting from tuning one note two different ways.^[1] The word "comma" used without qualification refers to the syntonic comma,^[2] which can be defined, for instance, as the difference between an F♯ tuned using the D-based Pythagorean tuning system, and another F♯ tuned using the D-based quarter-comma meantone system. Even within the same tuning system, when scales with more than 12 notes are built, two notes which should coincide may have a different tuning. For example, in extended scales produced with 5-limit tuning an A♭ tuned as a major third below C5 and a G♯ tuned as two major thirds above C4 will not be exactly the same note, as they would be in equal temperament. The difference between those notes, the diesis, also known as lesser diesis, is more than 40% of a semitone, and is easily audible.

Commas are often defined as the difference in size between two semitones, even within a single non-extended 12-tone scale. Each meantone temperament tuning system produces a 12-tone scale characterized by two different kinds of semitones (diatonic and chromatic), and hence by a comma of unique size. The same is true for Pythagorean tuning.

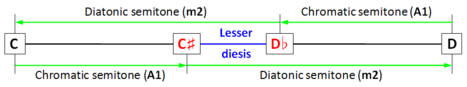
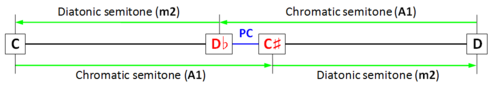
Lesser diesis defined in quarter-comma meantone as difference between semitones (m2 − A1 ≈ 117.1 − 76.0 ≈ 41.1 cents), or interval between enharmonically equivalent notes (from C♯ to D♭). The interval from C to D is narrower than in Pythagorean tuning (see below).  Play (help·info) Pythagorean comma (PC) defined in Pythagorean tuning as difference between semitones (A1 − m2 ≈ 113.7 − 90.2 ≈ 23.5 cents), or interval between enharmonically equivalent notes (from D♭ to C♯). The interval from C to D is wider than in quarter-comma meantone (see above).

In just intonation, more than two kinds of semitones may be produced. Thus, a single tuning system may be characterized by several different commas. For instance, a commonly used version of 5-limit tuning produces a 12-tone scale with four kinds of semitones and four commas.

The size of commas is commonly expressed and compared in terms of cents – 1/1200 fractions of an octave on a logarithmic scale.

Commas in different contexts

In the column labeled "Difference between semitones", m2 is the minor second (diatonic semitone), A1 is the augmented unison (chromatic semitone), and S₁, S₂, S₃, S₄ are semitones as defined here. In the columns labeled "Interval 1" and "Interval 2", all intervals are presumed to be tuned in just intonation. Notice that the Pythagorean comma (PC) and the syntonic comma (SC) are basic intervals which can be used as yardsticks to define some of the other commas. For instance, the difference between them is a small comma called schisma. A schisma is not audible in many contexts, as its size is narrower than the smallest noticeable difference between tones (which is around six cents).

Name of comma	Alternative Name	Definitions				Size
		Difference between semitones	Difference between commas	Difference between		Cents	Ratio
				Interval 1	Interval 2
Schisma	Skhisma	A1 − m2 in 1/12-comma meantone	1 PC − 1 SC	8 perfect fifths + 1 major third	5 octaves	1.95	32805:32768
Septimal kleisma				2 minor thirds	Septimal major third	7.71	225:224
Kleisma				6 minor thirds	Tritave (1 octave + 1 perfect fifth)	8.11	15625:15552
Small undecimal comma[3]						17.58	99:98
Diaschisma	Diaskhisma	m2 − A1 in 1/6-comma meantone, S3 − S2 in 5-limit tuning	2 SC − 1 PC	3 octaves	4 perfect fifths + 2 major thirds	19.55	2048:2025
Syntonic comma (SC)	Didymus' comma	S2 − S1 in 5-limit tuning		4 perfect fifths	2 octaves + 1 major third	21.51	81:80
				Major tone	Minor tone
Pythagorean comma (PC)	Ditonic comma	A1 − m2 in Pythagorean tuning		12 perfect fifths	7 octaves	23.46	531441:524288
Septimal comma[4]				Minor seventh	Septimal minor seventh	27.26	64:63
Diesis	Lesser diesis	m2 − A1 in 1/4-comma meantone, S3 − S1 in 5-limit tuning	3 SC − 1 PC	Octave	3 major thirds	41.06	128:125
Undecimal comma[5][6]	Undecimal quarter-tone			Undecimal tritone	Perfect fourth	53.27	33:32
Greater diesis		m2 − A1 in 1/3-comma meantone, S4 − S1 in 5-limit tuning	4 SC − 1 PC	4 minor thirds	Octave	62.57	648:625
Tridecimal comma	Tridecimal third-tone			Tridecimal tritone	Perfect fourth	65.3	27:26

The syntonic comma has a crucial role in the history of music. It is the amount by which some of the notes produced in Pythagorean tuning were flattened or sharpened to produce just minor and major thirds. In Pythagorean tuning, the only highly consonant intervals were the perfect fifth and its inversion, the perfect fourth. The Pythagorean major third (81:64) and minor third (32:27) were dissonant, and this prevented musicians from freely using triads and chords, forcing them to write music with relatively simple texture. In late Middle Ages, musicians realized that by slightly tempering the pitch of some notes, the Pythagorean thirds could be made consonant. For instance, if you decrease by a syntonic comma (81:80) the frequency of E, C-E (a major third), and E-G (a minor third) become just. Namely, C-E is flattened to a justly intonated ratio of

${81\over64} \cdot {80\over81} = {{1\cdot5}\over{4\cdot1}} = {5\over4}$

and at the same time E-G is sharpened to the just ratio of

${32\over27} \cdot {81\over80} = {{2\cdot3}\over{1\cdot5}} = {6\over5}$

This brought to the creation of a new tuning system, known as quarter-comma meantone, which permitted the full development of music with complex texture, such as polyphonic music, or melodies with instrumental accompaniment. Since then, other tuning systems were developed, and the syntonic comma was used as a reference value to temper the perfect fifths in an entire family of them. Namely, in the family belonging to the syntonic temperament continuum, including meantone temperaments.

Alternative definitions

In quarter-comma meantone, and any kind of meantone temperament tuning system which tempers the fifth to a size smaller than 700 cents, the comma can be equivalently defined as:

• A diminished second, that is the difference between minor second (diatonic semitone) and augmented unison (chromatic semitone).
• The difference between major second and diminished third.
• The difference between minor third and augmented second.
• The difference between major third and diminished fourth.
• The difference between perfect fourth and augmented third.
• The difference between augmented fourth and diminished fifth.
• The difference between perfect fifth and diminished sixth.
• The difference between minor sixth and augmented fifth.
• The difference between major sixth and diminished seventh.
• The difference between minor seventh and augmented sixth.
• The difference between major seventh and diminished octave.

In Pythagorean tuning, and any kind of meantone temperament tuning system which tempers the fifth to a size larger than 700 cents (such as 1/12-comma meantone), the comma is the opposite of the above mentioned differences. For instance, the Pythagorean comma is the opposite of a Pythagorean diminished second, i.e. it is the difference between chromatic semitone and diatonic semitone, rather than vice versa.

In each of the above mentioned tuning systems, these differences have all the same size. For instance, in Pythagorean tuning they are all equal to the opposite of a Pythagorean comma, and in quarter-comma meantone they are all equal to a diesis.

Notation

In the years 2000-2004, Marc Sabat and Wolfgang von Schweinitz worked together in Berlin to develop a method to exactly indicate pitches in staff notation. This method was called the extended Helmholtz-Ellis JI pitch notation.^[7] Sabat and Schweinitz take the "conventional" flats, naturals and sharps as a Pythagorean series of perfect fifths. Thus, a series of perfect fifths beginning with F proceeds C G D A E B F# and so on. The advantage for musicians is that conventional reading of the basic fourths and fifths remains familiar. Such an approach has also been advocated by Daniel James Wolf and by Joe Monzo, who refers to it by the acronym HEWM (Helmholtz-Ellis-Wolf-Monzo).^[8] In the Sabat-Schweinitz design, syntonic commas are marked by arrows attached to the flat, natural or sharp sign, septimal commas using Giuseppe Tartini's symbol, and undecimal quartertones using the common practice quartertone signs (a single cross and backwards flat). For higher primes, additional signs have been designed. To facilitate quick estimation of pitches, cents indications may be added (downward deviations below and upward deviations above the respective accidental). The convention used is that the cents written refer to the tempered pitch implied by the flat, natural, or sharp sign and the note name. One of the great advantages of such a notation is that it allows the natural harmonic series to be precisely notated. A complete legend and fonts for the notation (see samples) are open source and available from Plainsound Music Edition.

Tempering of commas

Commas are frequently used in the description of musical temperaments, where they describe distinctions between musical intervals that are eliminated by that tuning system. A comma can be viewed as the distance between two musical intervals. When a given comma is tempered out in a tuning system, the ability to distinguish between those two intervals in that tuning is eliminated. For example, the difference between the diatonic semitone and chromatic semitone is called the diesis. The widely used 12-tone equal temperament tempers out the diesis, and thus does not distinguish between the two different types of semitones. On the other hand, 19-tone equal temperament does not temper out this comma, and thus it distinguishes between the two semitones.

Examples:

• 12-TET tempers out the diesis, as well as a variety of other commas.
• 19-TET tempers out the septimal diesis and syntonic comma, but does not temper out the diesis.
• 22-TET tempers out the septimal comma of Archytas, but does not temper out the septimal diesis or syntonic comma.
• 31-TET tempers out the syntonic comma, as well as the comma defined by the ratio (99:98), but does not temper out the diesis, septimal diesis, or septimal comma of Archytas.

Comma sequence

A comma sequence defines a musical temperament through a unique sequence of commas at increasing prime limits.^[9] The first comma of the comma sequence will be in the q-limit, where q is the nth odd prime, and n is the number of generators. Subsequent commas will be in prime limits each one prime beyond the last.

Other intervals called commas

There are also several intervals called commas, which are not technically commas because they are not rational fractions like those above, but are irrational approximations of them. These include the Holdrian and Mercator's commas.

See also

• List of musical intervals
• Semicomma

Sources

1. ^ Waldo Selden Pratt (1922). Grove's Dictionary of Music and Musicians, Volume 1, p.568. John Alexander Fuller-Maitland, Sir George Grove, eds. Macmillan.
2. ^ Benson, Dave (2006). Music: A Mathematical Offering, p.171. ISBN 0521853877.
3. ^ Haluška, Ján (2003). The Mathematical Theory of Tone Systems, p.xxvi. ISBN 0824747143.
4. ^ David Dunn, 2000. Harry Partch: an anthology of critical perspectives.
5. ^ Rasch, Rudolph (2000). "A Word or Two on the Tunings of Harry Partch", Harry Partch: An Anthology of Critical Perspectives , p.34. Dunn, David, ed. ISBN 9057550652. Difference between 11-limit and 3-limit intervals.
6. ^ Rasch, Rudolph (1988). "Farey Systems of Musical Intonation", Listening 2, p.40. Benitez, J.M. et al., eds. ISBN 3718648466. Source for 32:33 as difference between 11:16 & 2:3.
7. ^ see article "The Extended Helmholtz-Ellis JI Pitch Notation: eine Notationsmethode für die natürlichen Intervalle" in "Mikrotöne und Mehr - Auf György Ligetis Hamburger Pfaden", ed. Manfred Stahnke, von Bockel Verlag, Hamburg 2005 ISBN 3-932696-62-X
8. ^ Tonalsoft Encyclopaedia article about 'HEWM' notation
9. ^ Smith, G.H., Comma Sequences, Xenharmony.