Spontaneous symmetry breaking

Quantum field theory
(Feynman diagram)
History of...
Background Gauge theory Field theory Poincaré symmetry Quantum mechanics Spontaneous symmetry breaking
Symmetries Crossing Charge conjugation Parity Time reversal
Tools Anomaly Effective field theory Expectation value Faddeev–Popov ghosts Feynman diagram Lattice gauge theory LSZ reduction formula Partition function Propagator Quantization Renormalization Vacuum state Wick's theorem Wightman axioms
Equations Dirac equation Klein–Gordon equation Proca equations Wheeler–DeWitt equation
Standard Model Electroweak interaction Higgs mechanism Quantum chromodynamics Quantum electrodynamics Yang–Mills theory
Incomplete theories Quantum gravity String theory Supersymmetry Technicolor Theory of everything
Scientists Jamal Nazrul Islam • Adler • Bethe • Bogoliubov • Callan • Candlin • Coleman • DeWitt • Dirac • Dyson • Fermi • Feynman • Fierz • Fröhlich • Gell-Mann • Goldstone • Gross • 't Hooft • Jackiw • Klein • Landau • Lee • Lehmann • Majorana • Nambu • Parisi • Polyakov • Salam • Schwinger • Skyrme • Stueckelberg • Symanzik • Tomonaga • Veltman • Weinberg • Weisskopf • Wilson • Witten • Yang • Yukawa • Hoodbhoy  • Zimmermann • Zinn-Justin
v · d · e

Spontaneous symmetry breaking is the process by which a system described in a theoretically symmetrical way ends up in an apparently asymmetric state.

Though the process in itself is interesting from a mathematical point of view, it is fairly simple. Its notoriety outside the mathematical community stems from its established role in the standard model of particle physics. In the context of its role within the standard model, it is more complicated (because it further involves the so-called Higgs mechanism—and the standard model itself is a complicated theory).

Illustration

For spontaneous symmetry breaking to occur, there must be a system in which there are several equally likely outcomes. The system as a whole is therefore symmetric with respect to these outcomes (if we consider any two outcomes, the probability is the same). However, if the system is sampled (i.e. if the system is actually used or interacted with in any way), a specific outcome must occur. Though we know the system as a whole is symmetric, we also see that it is never encountered with this symmetry, only in one specific state. Because one of the outcomes is always found with probability 1, and the others with probability 0, they are no longer symmetric.

Hence, the symmetry is said to be spontaneously broken in that theory. Nevertheless, the fact that each outcome is equally likely is a reflection of the underlying symmetry, which is thus often dubbed "hidden symmetry", and has crucial formal consequences, such as the presence of Nambu-Goldstone bosons.

Formal point of view

When a theory is symmetric with respect to a symmetry group, but requires that one element of the group is distinct, then spontaneous symmetry breaking has occurred. The theory must not dictate which member is distinct, only that one is. From this point on, the theory can be treated as if this element actually is distinct, with the proviso that any results found in this way must be resymmetrized, by taking the average of each of the elements of the group being the distinct one.

Everyday example

A common example to help explain this phenomenon is a ball sitting on top of a hill. This ball is in a completely symmetric state. It could move in various directions or it could sit and mark time. However, its state is unstable because it might move in some direction: the slightest perturbing force will cause the ball to roll down the hill. When the ball comes out of its unstable, symmetric state by moving symmetry has been broken, because the direction in which the ball rolled has a visible feature that distinguishes it from all other directions. The "choice" of direction is immaterial, however, as any other direction would do, i.e. the system is still bearing traces of the symmetry of the hill, albeit now somewhat less apparent.

Importance in the standard model

Main article: Higgs mechanism

Without spontaneous symmetry breaking, the Standard Model of elementary particle interactions predicts the existence of a number of particles. However, some particles (the W and Z bosons) would then be predicted to be massless, when, in reality, they are observed to have mass. (This would appear to be a major failing of the theory.) To overcome this, the combination of spontaneous symmetry breaking in conjunction with the Higgs mechanism gives these particles mass. It also suggests the presence of a new, as yet undetected particle, the Higgs boson.

If the Higgs boson is not found, it will mean that the simplest implementation of the Higgs mechanism and spontaneous symmetry breaking as they are currently formulated are invalid, requiring an alternative model implementation of SSB and the Higgs mechanism operative in the accepted theory of electroweak interactions.

A detailed presentation of the Higgs mechanism is given in the article on the Yukawa interaction, illustrating how it further gives mass to fermions.

Technical usage within physics

The crucial concept here is the order parameter. If there is a field (often a background field) which acquires an expectation value (not necessarily a vacuum expectation value) which is not invariant under the symmetry in question, we say that the system is in the ordered phase, and the symmetry is spontaneously broken. This is because other subsystems interact with the order parameter which forms a "frame of reference" to be measured against, so to speak. In that case, the vacuum state does not obey the initial symmetry (which would put it in the Wigner mode), and, instead has the (hidden) symmetry implemented in the Nambu-Goldstone mode. Normally, in the absence of the Higgs mechanism, massless Goldstone bosons arise.

The symmetry group can be discrete, such as the space group of a crystal, or continuous (e.g., a Lie group), such as the rotational symmetry of space. However, if the system contains only a single spatial dimension, then only discrete symmetries may be broken in a vacuum state of the full quantum theory, although a classical solution may break a continuous symmetry.

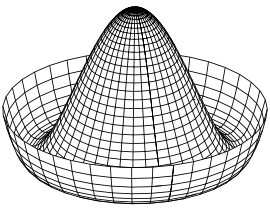
Mathematical example: the Mexican hat potential

Graph of a Mexican hat potential function.

In the simplest idealized relativistic model, the spontaneously broken field is described through a scalar field theory. In physics, one way of seeing spontaneous symmetry breaking is through the use of Lagrangians. Lagrangians, which essentially dictate how a system behaves, can be split up into kinetic and potential terms,

$(1) \qquad \mathcal{L} = \partial^\mu \phi \partial_\mu \phi - V(\phi).$

It is in this potential term (V(Φ)) that the symmetry breaking occurs. An example of a potential is illustrated in the graph at the right.

$(2) \qquad V(\phi) = -10|\phi|^2 + |\phi|^4 \,$

This potential has an infinite number of possible minima (vacuum states) given by

$(3) \qquad \phi = \sqrt{5} e^{i\theta}$

for any real θ between 0 and 2π. The system also has an unstable vacuum state corresponding to Φ = 0. This state has a U(1) symmetry. However, once the system falls into a specific stable vacuum state (amounting to a choice of θ), this symmetry will appear to be lost, or "spontaneously broken".

In fact, any other choice of θ would have exactly the same energy, implying the existence of a massless Nambu-Goldstone boson, the mode running around the circle at the minimum of this potential, and indicating there is some memory of the original symmetry in the Lagrangian.

Other examples

• For ferromagnetic materials, the underlying laws are invariant under spatial rotations. Here, the order parameter is the magnetization, which measures the magnetic dipole density. Above the Curie temperature, the order parameter is zero, which is spatially invariant, and there is no symmetry breaking. Below the Curie temperature, however, the magnetization acquires a constant nonvanishing value, which points in a certain direction (in the idealized situation where we have full equilibrium; otherwise, translational symmetry gets broken as well). The residual rotational symmetries which leave the orientation of this vector invariant remain unbroken, unlike the other rotations which do not and are thus spontaneously broken.
• The laws describing a solid are invariant under the full Euclidean group, but the solid itself spontaneously breaks this group down to a space group. The displacement and the orientation are the order parameters.
• General relativity has a Lorenz symmetry, but in FRW cosmological models, the mean 4-velocity field defined by averaging over the velocities of the galaxies (the galaxies act like gas particles at cosmological scales) acts as an order parameter breaking this symmetry. Similar comments can be made about the cosmic microwave background.
• For the electroweak model, as explained earlier, a component of the Higgs field provides the order parameter breaking the electroweak gauge symmetry to the electromagnetic gauge symmetry. Like the ferromagnetic example, there is a phase transition at the electroweak temperature. The same comment about us not tending to notice broken symmetries suggests why it took so long for us to discover electroweak unification.
• In superconductors, there is a condensed-matter collective field ψ, which acts as the order parameter breaking the electromagnetic gauge symmetry.
• Take a thin cylindrical plastic rod and push both ends together. Before buckling, the system is symmetric under rotation, and so visibly cylindrically symmetric. But after buckling, it looks different, and asymmetric. Nevertheless, features of the cylindrical symmetry are still there: ignoring friction, it would take no force to freely spin the rod around, displacing the ground state in time, and amounting to an oscillation of vanishing frequency, unlike the radial oscillations in the direction of the buckle. This spinning mode is effectively the requisite Nambu-Goldstone boson.
• Consider a uniform layer of fluid over an infinite horizontal plane. This system has all the symmetries of the Euclidean plane. But now heat the bottom surface uniformly so that it becomes much hotter than the upper surface. When the temperature gradient becomes large enough, convection cells will form, breaking the Euclidean symmetry.
• Consider a bead on a circular hoop that is rotated about a vertical diameter. As the rotational velocity is increased gradually from rest, the bead will initially stay at its initial equilibrium point at the bottom of the hoop (intuitively stable, lowest gravitational potential). At a certain critical rotational velocity, this point will become unstable and the bead will jump to one of two other newly created equilibria, equidistant from the center. Initially, the system is symmetric with respect to the diameter, yet after passing the critical velocity, the bead ends up in one of the two new equilibrium points, thus breaking the symmetry. Note: This can easily be tried at home with an electric drill, a marble, and a pot cover, (or any other combination you can think of).

Nobel Prize

On October 7, 2008, the Royal Swedish Academy of Sciences awarded the 2008 Nobel Prize in Physics to two Japanese citizens and a Japanese-born American for their work in subatomic physics symmetry breaking. American Yoichiro Nambu, 87, of the University of Chicago, won half of the prize for the discovery of the mechanism of spontaneous broken symmetry in the context of the strong interactions. (Japanese physicists Makoto Kobayashi and Toshihide Maskawa shared the other half of the prize for discovering the origin of the explicit breaking of CP symmetry in the weak interactions.^[1] This origin is ultimately reliant on the Higgs mechanism, but, so far understood as a "just so" feature of Higgs couplings, not a spontaneously broken symmetry phenomenon.)

See also

• Autocatalytic reactions and order creation
• Catastrophe theory
• CP-violation
• Dynamical symmetry breaking
• Explicit symmetry breaking
• Gauge gravitation theory
• Goldstone boson
• Grand unified theory
• Magnetic catalysis of chiral symmetry breaking
• Mermin-Wagner theorem
• Second-order phase transition
• Symmetry breaking
• Vacuum fluctuation
• J. J. Sakurai Prize for Theoretical Particle Physics
• Higgs mechanism
• Higgs boson
• 1964 PRL symmetry breaking papers

Notes

1. ^ The Nobel Foundation. "The Nobel Prize in Physics 2008". nobelprize.org. http://nobelprize.org/nobel_prizes/physics/laureates/2008/index.html. Retrieved January 15, 2008. 

External links

• Spontaneous symmetry breaking
• Physical Review Letters - 50th Anniversary Milestone Papers
• In CERN Courier, Steven Weinberg reflects on spontaneous symmetry breaking
• Englert-Brout-Higgs-Guralnik-Hagen-Kibble Mechanism on Scholarpedia
• History of Englert-Brout-Higgs-Guralnik-Hagen-Kibble Mechanism on Scholarpedia
• The History of the Guralnik, Hagen and Kibble development of the Theory of Spontaneous Symmetry Breaking and Gauge Particles
• International Journal of Modern Physics A: The History of the Guralnik, Hagen and Kibble development of the Theory of Spontaneous Symmetry Breaking and Gauge Particles
• Guralnik, G S; Hagen, C R and Kibble, T W B (1967). Broken Symmetries and the Goldstone Theorem. Advances in Physics, vol. 2 Interscience Publishers, New York. pp. 567-708 ISBN 0470170573
• Spontaneous Symmetry Breaking in Gauge Theories: a Historical Survey