- Convex regular 4-polytope
-
In mathematics, a convex regular 4-polytope is a 4-dimensional polytope that is both regular and convex. These are the four-dimensional analogs of the Platonic solids (in three dimensions) and the regular polygons (in two dimensions).
These polytopes were first described by the Swiss mathematician Ludwig Schläfli in the mid-19th century. Schläfli discovered that there are precisely six such figures. Five of these may be thought of as higher dimensional analogs of the Platonic solids. There is one additional figure (the 24-cell) which has no three-dimensional equivalent.
Each convex regular 4-polytope is bounded by a set of 3-dimensional cells which are all Platonic solids of the same type and size. These are fitted together along their respective faces in a regular fashion.
Contents
Properties
The following tables lists some properties of the six convex regular 4-dimensional polytopes. The symmetry groups of these polytopes are all Coxeter groups and given in the notation described in that article. The number following the name of the group is the order of the group.
Names Family Schläfli
symbolVertices Edges Faces Cells Vertex figures Dual polytope Symmetry group 5-cell
pentatope
hyperpyramid
hypertetrahedron
4-simplexsimplex
(n-simplex){3,3,3} 5 10 10
triangles5
tetrahedratetrahedra (self-dual) A4 120 8-cell
Tesseract
hypercube
4-cubehypercube
(n-cube){4,3,3} 16 32 24
squares8
cubestetrahedra 16-cell B4 384 16-cell
orthoplex
hyperoctahedron
4-orthoplexcross-polytope
(n-orthoplex){3,3,4} 8 24 32
triangles16
tetrahedraoctahedra tesseract B4 384 24-cell
octaplex
polyoctahedron{3,4,3} 24 96 96
triangles24
octahedracubes (self-dual) F4 1152 120-cell
dodecaplex
hyperdodecahedron
polydodecahedron{5,3,3} 600 1200 720
pentagons120
dodecahedratetrahedra 600-cell H4 14400 600-cell
tetraplex
hypericosahedron
polytetrahedron{3,3,5} 120 720 1200
triangles600
tetrahedraicosahedra 120-cell H4 14400 Since the boundaries of each of these figures is topologically equivalent to a 3-sphere, whose Euler characteristic is zero, we have the 4-dimensional analog of Euler's polyhedral formula:
where Nk denotes the number of k-faces in the polytope (a vertex is a 0-face, an edge is a 1-face, etc.).
Visualizations
The following table shows some 2 dimensional projections of these polytopes. Various other visualizations can be found in the external links below. The Coxeter-Dynkin diagram graphs are also given below the Schläfli symbol.
5-cell 8-cell 16-cell 24-cell 120-cell 600-cell {3,3,3} {4,3,3} {3,3,4} {3,4,3} {5,3,3} {3,3,5} 









































Wireframe orthographic projections inside Petrie polygons. 
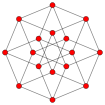
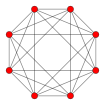
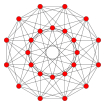


Solid orthographic projections 
tetrahedral
envelope
(cell/vertex-centered)
cubic envelope
(cell-centered)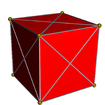
Cubic envelope
(cell-centered)
cuboctahedral
envelope
(cell-centered)
truncated rhombic
triacontahedron
envelope
(cell-centered)
Pentakis icosidodecahedral
envelope
(vertex-centered)Wireframe Schlegel diagrams (Perspective projection) 
(Cell-centered)
(Cell-centered)
(Cell-centered)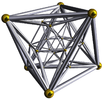
(Cell-centered)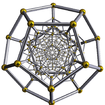
(Cell-centered)
(Vertex-centered)Wireframe stereographic projections (Hyperspherical) 
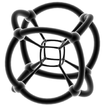




See also
- Infinite regular 4-polytopes:
- One regular Euclidean honeycomb: {4,3,4}
- Four regular hyperbolic honeycombs: {3,5,3}, {4,3,5}, {5,3,4}, {5,3,5}
- Nonconvex regular 4-polytopes:
- Schläfli-Hess polychoron - Ten nonconvex regular 4-polytopes
- Abstract regular polychora:
- Uniform polychoron 4-polytope families constructed from the from these 6 regular forms.
- Regular polytope
- Platonic solid
References
- H. S. M. Coxeter, Introduction to Geometry, 2nd ed., John Wiley & Sons Inc., 1969. ISBN 0-471-50458-0.
- H. S. M. Coxeter, Regular Polytopes, 3rd. ed., Dover Publications, 1973. ISBN 0-486-61480-8.
- D. M. Y. Sommerville, An Introduction to the Geometry of n Dimensions. New York, E. P. Dutton, 1930. 196 pp. (Dover Publications edition, 1958) Chapter X: The Regular Polytopes
External links
- Weisstein, Eric W., "Regular polychoron" from MathWorld.
- Jonathan Bowers, 16 regular polychora
- Regular 4D Polytope Foldouts
- Catalog of Polytope Images A collection of stereographic projections of 4-polytopes.
- A Catalog of Uniform Polytopes
- Dimensions 2 hour film about the fourth dimension (contains stereographic projections of all regular polychorons)
8-cell 16-cell 24-cell 120-cell 600-cell {3,3,3}
pentachoron{4,3,3}
tesseract{3,3,4}
hexadecachoron{3,4,3}
icositetrachoron{5,3,3}
hecatonicosachoron{3,3,5}
hexacosichoronCategories:- Four-dimensional geometry
- Polychora
Wikimedia Foundation. 2010.


