Nitrogen-vacancy center

The nitrogen-vacancy center (N-V center) is one of numerous point defects in diamond. Its most explored and useful property is photoluminescence, which can be easily detected from an individual N-V center. Electron spins at N-V centers, localized at atomic scales, can be manipulated at room temperature by applying a magnetic field, electric field, microwave radiation or light, or a combination, resulting in sharp resonances in the intensity and wavelength of the photoluminescence. These resonances can be explained in terms of electron spin related phenomena such as quantum entanglement, spin-orbit interaction and Rabi oscillations, and analysed using advanced quantum optics theory. An individual N-V center can be viewed as a basic unit of a quantum computer, and it has potential applications in novel, more efficient fields of electronics and computational science including spintronics, quantum cryptography and quantum computing.

Structure

Primitive picture of the N-V⁻ center

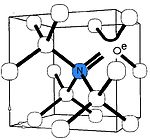
The nitrogen-vacancy center is a point defect in the diamond lattice. It consists of a nearest-neighbor pair of a nitrogen atom, which substitutes for a carbon atom, and a lattice vacancy.

Two charge states of this defect, neutral N-V⁰ and negative N-V⁻, are known from spectroscopic studies using optical absorption,^[1][2] photoluminescence (PL),^[3] electron paramagnetic resonance (EPR)^[4][5][6] and optically detected magnetic resonance (ODMR),^[7] which can be viewed as a hybrid of PL and EPR; most details of the structure originate from EPR. A nitrogen atom has five valence electrons. Three of them covalently bond to the carbon atoms and two remain non-bonded and are called a lone pair. The vacancy has three unpaired electrons. Two of them make a quasi covalent bond and one remains unpaired. The overall symmetry, however, is axial (trigonal C_3V); one can visualize this by imagining the three unpaired vacancy electrons continuously exchanging their roles.

The N-V⁰ thus has one unpaired electron and is paramagnetic. However, despite extensive efforts, electron paramagnetic resonance signals from N-V⁰ avoided detection for decades until 2008. Optical excitation is required to bring the N-V⁰ defect into the EPR-detectable excited state; the signals from the ground state are presumably too broad for EPR detection.^[8]

In the negative charge state N-V⁻, the extra electron is located at the vacancy site forming a spin S=1 pair with one of the vacancy electrons. As in N-V⁰, the vacancy electrons are "exchanging roles" preserving the overall trigonal symmetry. This N-V⁻ state is what is commonly, and somewhat incorrectly, called "the nitrogen-vacancy center". The neutral state has not yet been explored for spin manipulations.

The N-V centers are randomly oriented within a diamond crystal. Ion implantation techniques can enable their artificial creation in predetermined positions.^[9]

Production

Main article: Crystallographic defects in diamond

Nitrogen-vacancy centers are produced from single substitutional nitrogen centers (called C or P1 centers in diamond literature) by irradiation followed by annealing at temperatures above 700 ⁰C.^[1] A wide range of high-energy particles are suitable for such irradiation, including electrons, protons, neutrons, ions, and gamma photons. Irradiation produces lattice vacancies, which are a part of N-V centers. Those vacancies are immobile at room temperature, and annealing is required to move them. Single substitutional nitrogen produces strain in the diamond lattice;^[10] it therefore efficiently captures moving vacancies,^[11] producing the N-V centers.

Diamonds are notorious by having relatively large lattice strain. Strain splits and shifts optical transitions from individual centers resulting in broad lines in the ensembles of centers.^[1] Special care is taken to produce extremely sharp N-V lines (line width ~10 MHz)^[12] required for most experiments: high-quality, pure natural or better synthetic diamonds (type IIa) are selected. Many of them already have sufficient concentrations of grown-in N-V centers and are suitable for applications. If not, they are irradiated by high-energy particles and annealed. Selection of a certain irradiation dose allows tuning the concentration of produced N-V centers such that individual N-V centers are separated by micrometre-large distances. Then, individual N-V centers can be studied with standard optical microscopes or, better, near-field scanning optical microscopes having sub-micrometre resolution.^[7][13]

Basic optical properties

Optical absorption and emission of the N-V⁻ center at room temperature

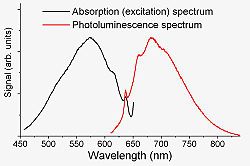
N-V⁻ centers emit bright red light which can be conveniently excited by visible light sources, such as argon or krypton lasers, frequency doubled Nd:YAG lasers, dye lasers, or He-Ne lasers. Laser illumination, however, also converts some N-V⁻ into N-V⁰ centers.^[3] Emission is very quick (relaxation time ~10 ns).^[14][15] At room temperature, no sharp peaks are observed because of the thermal broadening. However, cooling the N-V⁻ centers with liquid nitrogen or liquid helium dramatically narrows the lines down to few megahertz width.

An important property of the luminescence from individual N-V⁻ centers is its high temporal stability. Whereas many single-molecular emitters bleach after emission of 10⁶–10⁸ photons, no bleaching is observed for the N-V centers at room temperature.^[7][13]

Energy level structure and its manipulation by external fields

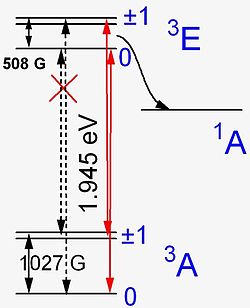
Schematic energy level structure of the N-V⁻ center. Electron transitions between the ground ³A and excited ³E states, separated by 1.945 eV (637 nm), produce absorption and luminescence. The ³A state is split by 1027 gauss^[4][5] (~5.6 µeV) and the ³E state by 508 gauss^[16] (~2.9 µeV). Numbers 0, ±1 indicate spin value; splitting due to the orbital degeneracy is not shown.

The energy level structure of the N-V⁻ center was established by combining optical, electron paramagnetic resonance and theoretical results, as shown in the figure. In particular, several theoretical works have been done, using the Linear Combination of Atomic Orbitals (LCAO) approach, to build the electronic orbitals to describe the possible quantum states, looking at the NV center as a molecule. Moreover, group theory results are used, to take into account the symmetry of the diamond crystal, and so the symmetry of the NV itself. The energy levels are labeled according to the group theory, and in particular are labelled after the irreducible representations of the C_3V symmetry group of the defect center, A₁, A₂ and E. The numbers 3 in ³A and 1 in ¹A represent the number of allowable m_s spin states, or the spin multiplicity, which range from –S to S for a total of 2S+1 possible states. If S = 1, m_s can be –1, 0, or 1. The ¹A level is predicted by theory but not directly observed in experiment, and it is believed to play an important role in the quenching of photoluminescence.

In the absence of an external magnetic field, the ground and excited states are split by the magnetic interaction between the two unpaired electrons at the N-V⁻ center (see microscopic model): when two electrons have parallel spins (m_s=±1), their energy is higher than when spins are antiparallel (m_s=0). The farther apart the electrons are, the weaker their interaction energy D (roughly D ~1/r³).^[5] Thus the smaller splitting in the excited state can be viewed in terms of larger electron-electron separation in the excited state. When an external magnetic field is applied to the N-V⁻ center, it does not affect the m_s=0 states nor the ¹A state (because it has S = 0), but it splits the m_s = ±1 levels. If a magnetic field is oriented along the defect axis and reaches about 1027 G (or 508 G) then the m_s = –1 and m_s = 0 states in the ground (or excited) state become equal in energy; they strongly interact resulting in so-called spin polarization, which strongly affects the intensity of optical absorption and luminescence transitions involving those states.^[16]

In order to understand why this happens, we have to keep in mind that transitions between electronic states are mediated by a photon which cannot change overall spin. Thus optical transitions must preserve the total spin and occur between levels of the same total spin. For this reason, transitions ³E↔¹A and ¹A ↔ ³A are non-radiative and quench the luminescence. Whereas m_s = −1 (excited state) ↔ m_s = 0 (ground state) transition was forbidden in the absence of an external magnetic field, it becomes allowed when a magnetic field mixes the m_s = −1 and m_s = 0 levels in the ground state. As a measurable outcome of this phenomenon, luminescence intensity can be strongly modulated by magnetic field.

There is an additional level splitting in the excited ³E state due to the orbital degeneracy and spin-orbit interaction. Importantly, this splitting can be modulated by applying a static electric field,^[12][17] in a similar fashion to the magnetic field mechanism outlined above, though the physics of the splitting is somewhat more complex. Nevertheless, an important practical outcome is that the intensity and position of the luminescence lines can be modulated by applying electric or/and magnetic fields.

The energy difference between the m_s = 0 and m_s = ±1 states corresponds to the microwave region. Thus by irradiating the N-V centers with microwave radiation, one can change the relative population of those levels, thereby again modulating the luminescence intensity.

There is an additional splitting of the m_s = ±1 energy levels, which originates from the "hyperfine" interaction between the nuclear and electron spins. Thus finally, the optical absorption and luminescence from the N-V⁻ center consists of roughly a dozen sharp lines with a separation in the MHz-GHz range, and all those lines can be resolved, given proper sample preparation. The intensity and position of those lines can be modulated using the following tools:

1. Amplitude and orientation of magnetic field, which splits the m_s = ±1 levels in the ground and excited states.
2. Amplitude and orientation of elastic field (strain), which can be applied by, e.g., squeezing the diamond. Similar effects can be induced by applying electric field,^[12][17] and the electric field can be controlled with much higher precision.
3. Continuous-wave microwave radiation, which changes the population of the sublevels within the ground and excited state.^[17]
4. Tunable laser, which can selectively excite certain sublevels of the ground and excited state.^[17][18]
5. In addition to those static perturbations, numerous dynamic effects (spin echo, Rabi oscillations, etc.) can be exploited by applying a carefully designed sequence of microwave pulses.^{[19][20][21][22][23]} The first pulse coherently excites the electron spins, and this coherence is then manipulated and probed by the subsequent pulses. Those dynamic effects are rather important for practical realization of quantum computers, which ought to work at high frequency.

As a final remark, it should be noted that the above-described energy structure is by no means exceptional for a defect in diamond or other semiconductor (see, e.g.^[24]). It was not this structure alone, but a combination of several favorable factors (previous knowledge, easy production and excitation, etc.) which suggested the use of the N-V⁻ center.

Biological applications

In addition to the quantum optical applications, luminescence from the N-V⁻ centers can be applied for imaging biological processes, such as fluid flow in living cells.^[25] This application relies on good compatibility of diamond nanoparticles with the living cells and on favorable properties of photoluminescence from the N-V⁻ centers (strong intensity, easy excitation and detection, temporal stability, etc.). Compared with large single-crystal diamonds, nanodiamonds are cheap (~1 USD per gram) and available from various suppliers. N-V⁻ centers are produced in diamond powders with submicrometre particle size using the standard process of irradiation and annealing described above. Those nanodiamonds are introduced in a cell, and their luminescence is monitored using a standard fluorescence microscope.

Historical remarks

The microscopic model and most optical properties of ensembles of the N-V⁻ centers have been firmly established in the 1970s based on the optical measurements combined with uniaxial stress^[1] and on the electron paramagnetic resonance.^[4][5] However, a minor error in EPR results (it was assumed that illumination is required to observe N-V⁻ EPR signals) resulted in the incorrect multiplicity assignments in the energy level structure. In 1991 it was shown that EPR can be observed without illumination,^[6] which established the energy level scheme shown above. The magnetic splitting in the excited state has been measured only recently.^[16]

Regarding the characterization of single N-V⁻ centers, this field is very competitive nowadays; numerous papers have been published in the major scientific journals and reviewing them is hardly possible on these pages. However, one result published in 1997 is worth mentioning.^[7] In that paper, it was demonstrated that the fluorescence of single N-V⁻ centers can be detected by room-temperature fluorescence microscopy and also that the defect shows perfect photostability. Also one of the outstanding properties of the NV center was demonstrated, namely room temperature optically detected magnetic resonance.

References

1. ^ ^{a b c d} Davies, G.; Hamer, M. F. (1976). "Optical Studies of the 1.945 eV Vibronic Band in Diamond". Proceedings of the Royal Society of London. Series A, Mathematical and Physical Sciences (1934-1990) 348 (1653): 285. Bibcode 1976RSPSA.348..285D. doi:10.1098/rspa.1976.0039. 
2. ^ Mita, Yoshimi (1996). "Change of absorption spectra in type-Ib diamond with heavy neutron irradiation". Physical Review B 53 (17): 11360. Bibcode 1996PhRvB..5311360M. doi:10.1103/PhysRevB.53.11360. 
3. ^ ^{a b} Iakoubovskii, K; Adriaenssens, G J; Nesladek, M (2000). Journal of Physics: Condensed Matter 12 (2): 189. Bibcode 2000JPCM...12..189I. doi:10.1088/0953-8984/12/2/308. 
4. ^ ^{a b c} Loubser, J.H.N. and van Wyk, J.A. Diamond Res. 11 (1977) 4
5. ^ ^{a b c d} Loubser, J H N; Wyk, J A van (1978). "Electron spin resonance in the study of diamond". Reports on Progress in Physics 41 (8): 1201. Bibcode 1978RPPh...41.1201L. doi:10.1088/0034-4885/41/8/002. 
6. ^ ^{a b} Redman, D.; Brown, S.; Sands, R.; Rand, S. (1991). "Spin dynamics and electronic states of N-V centers in diamond by EPR and four-wave-mixing spectroscopy". Physical Review Letters 67 (24): 3420–3423. Bibcode 1991PhRvL..67.3420R. doi:10.1103/PhysRevLett.67.3420. PMID 10044729. 
7. ^ ^{a b c d} Gruber, A.; Dräbenstedt, A.; Tietz, C.; Fleury, L.; Wrachtrup, J.; von Borczyskowski, C. (1997). "Scanning Confocal Optical Microscopy and Magnetic Resonance on Single Defect Centers". Science 276 (5321): 2012. doi:10.1126/science.276.5321.2012. 
8. ^ Felton, S.; Edmonds, A. M.; Newton, M. E.; Twitchen, D. J.; Fisher, D.; Twitchen, D. (2008). "Electron paramagnetic resonance studies of the neutral nitrogen vacancy in diamond". Physical Review B 77 (8): 081201. Bibcode 2008PhRvB..77h1201F. doi:10.1103/PhysRevB.77.081201. 
9. ^ David D. Awschalom, Ryan Epstein and Ronald Hanson (October 2007). Diamond Age of Spintronics. Scientific American. pp. 90. 
10. ^ Lang, A. R.; Moore, M.; Makepeace, A. P. W.; Wierzchowski, W.; Welbourn, C. M. (1991). "On the Dilatation of Synthetic Type Ib Diamond by Substitutional Nitrogen Impurity". Philosophical Transactions of the Royal Society: Physical and Engineering Sciences (1990-1995) 337 (1648): 497. Bibcode 1991RSPTA.337..497L. doi:10.1098/rsta.1991.0135. 
11. ^ . Bibcode 2001JPCM...13.6015I. doi:10.1088/0953-8984/13/26/316. 
12. ^ ^{a b c} Tamarat, Ph.; Gaebel, T.; Rabeau, J.; Khan, M.; Greentree, A.; Wilson, H.; Hollenberg, L.; Prawer, S. et al. (2006). "Stark Shift Control of Single Optical Centers in Diamond". Physical Review Letters 97 (8): 083002. arXiv:quant-ph/0607170. Bibcode 2006PhRvL..97h3002T. doi:10.1103/PhysRevLett.97.083002. PMID 17026299. 
13. ^ ^{a b} Kuhn, S.; Hettich, C.; Schmitt, C.; Poizat, J-PH.; Sandoghdar, V. (2001). "Diamond colour centres as a nanoscopic light source for scanning near-field optical microscopy". Journal of Microscopy 202 (Pt 1): 2–6. doi:10.1046/j.1365-2818.2001.00829.x. PMID 11298860. 
14. ^ Collins, A T; Thomaz, M F; Jorge, M I B (1983). "Luminescence decay time of the 1.945 eV centre in type Ib diamond". Journal of Physics C: Solid State Physics 16 (11): 2177. Bibcode 1983JPhC...16.2177C. doi:10.1088/0022-3719/16/11/020. 
15. ^ Hanzawa, H (1997). "Measurement of decay time for the NV centre in Ib diamond with a picosecond laser pulse". Diamond and Related Materials 6 (11): 1595. doi:10.1016/S0925-9635(97)00037-X. 
16. ^ ^{a b c} Fuchs, G. D.; Dobrovitski, V. V.; Hanson, R.; Batra, A.; Weis, C. D.; Schenkel, T.; Awschalom, D. D. (2008). "Excited-State Spectroscopy Using Single Spin Manipulation in Diamond". Physical Review Letters 101 (11): 117601. Bibcode 2008PhRvL.101k7601F. doi:10.1103/PhysRevLett.101.117601. PMID 18851332. 
17. ^ ^{a b c d} Tamarat, Ph; Manson, N B; Harrison, J P; McMurtrie, R L; Nizovtsev, A; Santori, C; Beausoleil, R G; Neumann, P et al. (2008). "Spin-flip and spin-conserving optical transitions of the nitrogen-vacancy centre in diamond". New Journal of Physics 10 (4): 045004. Bibcode 2008NJPh...10d5004T. doi:10.1088/1367-2630/10/4/045004. 
18. ^ Santori, Charles; Tamarat, Philippe; Neumann, Philipp; Wrachtrup, Jörg; Fattal, David; Beausoleil, Raymond; Rabeau, James; Olivero, Paolo et al. (2006). "Coherent Population Trapping of Single Spins in Diamond under Optical Excitation". Physical Review Letters 97 (24): 247401. arXiv:quant-ph/0607147. Bibcode 2006PhRvL..97x7401S. doi:10.1103/PhysRevLett.97.247401. PMID 17280321. 
19. ^ Hanson, R.; Gywat, O.; Awschalom, D. D. (2006). "Room-temperature manipulation and decoherence of a single spin in diamond". Physical Review B 74 (16): 161203. arXiv:quant-ph/0608233. Bibcode 2006PhRvB..74p1203H. doi:10.1103/PhysRevB.74.161203. 
20. ^ Dutt, M. V. G.; Childress, L.; Jiang, L.; Togan, E.; Maze, J.; Jelezko, F.; Zibrov, A. S.; Hemmer, P. R. et al. (2007). "Quantum Register Based on Individual Electronic and Nuclear Spin Qubits in Diamond". Science 316 (5829): 1312–6. Bibcode 2007Sci...316.....D. doi:10.1126/science.1139831. PMID 17540898. 
21. ^ Childress, L.; Gurudev Dutt, M. V.; Taylor, J. M.; Zibrov, A. S.; Jelezko, F.; Wrachtrup, J.; Hemmer, P. R.; Lukin, M. D. (2006). "Coherent Dynamics of Coupled Electron and Nuclear Spin Qubits in Diamond". Science 314 (5797): 281–5. Bibcode 2006Sci...314..281C. doi:10.1126/science.1131871. PMID 16973839. 
22. ^ Batalov, A.; Zierl, C.; Gaebel, T.; Neumann, P.; Chan, I.-Y.; Balasubramanian, G.; Hemmer, P. R.; Jelezko, F. et al. (2008). "Temporal Coherence of Photons Emitted by Single Nitrogen-Vacancy Defect Centers in Diamond Using Optical Rabi-Oscillations". Physical Review Letters 100 (7): 077401. Bibcode 2008PhRvL.100g7401B. doi:10.1103/PhysRevLett.100.077401. PMID 18352594. 
23. ^ Jelezko, F.; Gaebel, T.; Popa, I.; Gruber, A.; Wrachtrup, J. (2004). "Observation of Coherent Oscillations in a Single Electron Spin". Physical Review Letters 92 (7): 076401. Bibcode 2004PhRvL..92g6401J. doi:10.1103/PhysRevLett.92.076401. PMID 14995873. 
24. ^ Aharonovich, Igor; Zhou, Chunyuan; Stacey, Alastair; Orwa, Julius; Castelletto, Stefania; Simpson, David; Greentree, Andrew; Treussart, François et al. (2009). "Enhanced single-photon emission in the near infrared from a diamond color center". Physical Review B 79 (23): 235316. Bibcode 2009PhRvB..79w5316A. doi:10.1103/PhysRevB.79.235316. 
25. ^ Chang, Yi-Ren; Lee, Hsu-Yang; Chen, Kowa; Chang, Chun-Chieh; Tsai, Dung-Sheng; Fu, Chi-Cheng; Lim, Tsong-Shin; Tzeng, Yan-Kai et al. (2008). "Mass production and dynamic imaging of fluorescent nanodiamonds". Nature Nanotechnology 3 (5): 284–8. doi:10.1038/nnano.2008.99. PMID 18654525. 

See also

• Crystallographic defects in diamond
• Crystallographic defect
• Material properties of diamond