- Triangulated category
-
A triangulated category is a mathematical category satisfying some axioms that are based on the properties of the homotopy category of spectra, and the derived category of an abelian category. A t-category is a triangulated category with a t-structure.
Contents
History
The notion of a derived category was introduced by Jean-Louis Verdier (1963) in his Ph.D. thesis, based on the ideas of Grothendieck. He also defined the notion of a triangulated category, based upon the observation that a derived category had some special "triangles", by writing down axioms for the basic properties of these triangles. A very similar set of axioms was written down at about the same time by Dold and Puppe (1961).
Definition
A translation functor on a category D is an automorphism (or for some authors, an auto-equivalence) T from D to D. One usually uses the notation
![X[n] = T^n X\](b/81b1f7eed546e31d57b5d170f1647c9a.png) and likewise for morphisms from X to Y.
and likewise for morphisms from X to Y.A triangle (X, Y, Z, u, v, w) is a set of 3 objects X, Y, and Z, together with morphisms u from X to Y, v from Y to Z and w from Z to X[1]. Triangles are generally written in the unravelled form:
There are two ways to rotate the above triangle:
![Y \xrightarrow{v} Z \xrightarrow{w} X[1] \xrightarrow{-u[1]} Y[1]](f/49f3659746789c93785d6d1f3dcc5dd3.png) or
or ![Z[-1] \xrightarrow{-w[-1]} X \xrightarrow{u} Y \xrightarrow{v} Z.\](7/957dd8c0353d6d9c5bbef592ee52d8f6.png)
A triangulated category is an additive category D with a translation functor and a class of triangles, called distinguished triangles, satisfying the following properties:
- (TR 1) Every triangulated category contains the following triangles:
- For any object X, the following triangle is distinguished:
- For any morphism from X to Y, there is an object Z (called a mapping cone of the morphism) fitting into a distinguished triangle
- Any triangle isomorphic to a distinguished triangle is distinguished.
- For any object X, the following triangle is distinguished:
- (TR 2) The rotations of any distinguished triangle are distinguished.
- (TR 3) Given a map between two morphisms, there is a morphism between their mapping cones (which exist by axiom (TR 1)), that makes everything commute. This means that in the following diagram (where f and g form the map of morphisms) there exists some map h (not necessarily unique) making all the squares commute:
- (TR 4) This is called the octahedral axiom. Suppose we have morphisms from X to Y and Y to Z, so that we also have a composed morphism from X to Z. Form distinguished triangles for each of these three morphisms. The octahedral axiom states (roughly) that the three mapping cones can be made into the vertices of a distinguished triangle so that "everything commutes".
These axioms are not entirely independent, since (TR 3) can be derived from the others.
The octahedral axiom
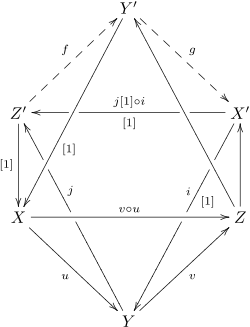
The final axiom (TR 4) is called the "octahedral axiom" because drawing all the objects and morphisms gives the skeleton of an octahedron, four of whose faces are distinguished triangles. There seems to be no really satisfactory way to draw everything in two dimensions; see (Kashiwara & Schapira 2002) for details. The presentation here is Verdier's own, and appears, complete with octahedral diagram, in (Hartshorne 1966). In the following diagram, u and v are the given morphisms, and the primed letters are the cones of various maps (chosen so that every distinguished triangle has an X, a Y, and a Z letter). Various arrows have been marked with [1] to indicate that they are of "degree 1"; e.g. the map from Z′ to X is in fact from Z′ to T(X). The octahedral axiom then asserts the existence of maps f and g forming a distinguished triangle, and so that f and g form commutative triangles in the other faces which contain them:
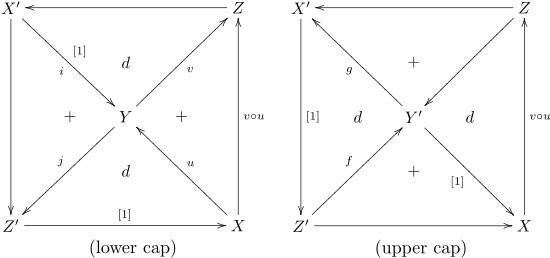
Two different pictures appear in (Beilinson, Bernstein & Deligne 1982) (Gelfand and Manin (2006) also present the first one). The first presents the upper and lower pyramids of the above octahedron and asserts that given a lower pyramid, we can fill in an upper pyramid so that the two paths from Y to Y′, and from Y′ to Y, are equal (this condition is omitted, perhaps erroneously, from Hartshorne's presentation). The triangles marked + are commutative and those marked "d" are distinguished:
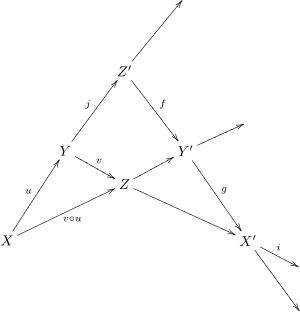
The second diagram is a more innovative presentation. Distinguished triangles are presented linearly, and the diagram emphasizes the fact that the four triangles in the "octahedron" are connected by a series of maps of triangles, where three triangles (namely, those completing the morphisms from X to Y, from Y to Z, and from X to Z) are given and the existence of the fourth is claimed. We pass between the first two by "pivoting" about X, to the third by pivoting about Z, and to the fourth by pivoting about X′. All enclosures in this diagram are commutative (both trigons and the square) but the other commutative square, expressing the equality of the two paths from Y′ to Y, is not evident. All the arrows pointing "off the edge" are degree 1:
This last diagram also illustrates a useful intuitive interpretation of the octahedral axiom. Since in triangulated categories, triangles play the role of exact sequences, we can pretend that
 in which case the existence of the last triangle expresses on the one hand
in which case the existence of the last triangle expresses on the one hand (looking at the triangle
(looking at the triangle  ), and
), and (looking at the triangle
(looking at the triangle  ).
).
Putting these together, the octahedral axiom asserts the "third isomorphism theorem":
When the triangulated category is K(A) for some abelian category A, and when X, Y, Z are objects of A placed in degree 0 in their eponymous complexes, and when the maps
 are injections in A, then the cones are literally the above quotients, and the pretense becomes truth.
are injections in A, then the cones are literally the above quotients, and the pretense becomes truth.Finally, Neeman (2001) gives a way of expressing the octahedral axiom using a two dimensional commutative diagram with 4 rows and 4 columns. Beilinson, Bernstein, and Deligne (1982) also give generalizations of the octahedral axiom.
Are there better axioms?
These axioms seem rather artificial. It is strongly suspected by experts (see, for example, (Gelfand & Manin 2006, Introduction, Chapter IV)) that triangulated categories are not really the "correct" concept. The essential reason is that the mapping cone of a morphism is unique only up to a non-unique isomorphism. In particular the mapping cone of a morphism does not in general depend functorially on the morphism (note the non-uniqueness in axiom (TR 3), for example). This non-uniqueness is a potential source of errors, among other things preventing in many cases a triangulated category from being the derived category of its core (with respect to a particular t-structure). The axioms do however seem to work adequately in practice, and there is currently no convincing replacement. A few proposals have been developed, however, such as derivators that Grothendieck has developed in his long, unfinished and unpublished manuscript from 1991.
Cohomology in triangulated categories
Triangulated categories admit a notion of cohomology and every triangulated category includes a large number of cohomological functors. By definition, a functor F from a triangulated category D into an abelian category A is a cohomological functor if for every distinguished triangle
which can be written as the doubly infinite sequence of morphisms
the following sequence (obtained by applying F to this one) is a long exact sequence:
In a general triangulated category we are guaranteed that the functors
 for any object A, are cohomological, with values in the category of abelian groups (the latter is a contravariant functor, which we view as taking values in the opposite category, also abelian). That is, we have for example an exact sequence (for the above triangle)
for any object A, are cohomological, with values in the category of abelian groups (the latter is a contravariant functor, which we view as taking values in the opposite category, also abelian). That is, we have for example an exact sequence (for the above triangle)The functors are also written
in analogy with the Ext functors in derived categories. Thus we have the familiar sequence
Examples
1. Vector spaces (over a field) form an elementary triangulated category in which X[1]=X for all X. A distinguished triangle is a sequence
 which is exact at X, Y and Z.
which is exact at X, Y and Z.2. If A is an abelian category, then the homotopy category K(A) has as objects all complexes of objects of A, and as morphisms the homotopy classes of morphisms of complexes. Then K(A) is a triangulated category; the distinguished triangles consist of triangles isomorphic to a morphism with its mapping cone (in the sense of chain complexes). It is possible to create variations, using complexes that are bounded on the left, or on the right, or on both sides.
3. The derived category of A is also a triangulated category; it is created from K(A) by localizing at the class of quasi-isomorphisms, a process we now describe.
Under some reasonable conditions on the localizing set S, a localization of a triangulated category is also triangulated. In particular, these conditions are:
- S is closed under all translations, and
- For any two triangles
![X \to Y \to Z \to X[1],](7/617a67149c7662794fa2c205a57ff6d3.png)
![X' \to Y' \to Z' \to X'[1]](6/e166b007c595650d9841108947c3496e.png) and arrows
and arrows  as in the axioms, if these arrows are both in S then the promised arrow
as in the axioms, if these arrows are both in S then the promised arrow  completing the map of triangles is also in S.
completing the map of triangles is also in S.
S is then said to be "compatible with the triangulation". It is not hard to see that this is the case when S is the class of quasi-isomorphisms in K(A), so in particular the derived category of A, which is the localization of K(A) with respect to quasi-isomorphisms, is triangulated.
4. The topologist's stable homotopy category is another example of a triangulated category. The objects are spectra, the suspension is the translation functor, and the cofibration sequences are the distinguished triangles.
t-structures
Verdier introduced triangulated categories in order to place derived categories in a category-theoretic context: for every abelian category A there exists a triangulated category D(A), containing A as a full subcategory (the "0-complexes" concentrated in cohomological degree 0), and in which we can construct derived functors. Unfortunately, different abelian categories can give rise to equivalent derived categories, so that it is impossible to reconstruct A from the triangulated category D(A).
A partial solution to this problem, is to impose a t-structure on the triangulated category D. Different t-structures on D will give rise to different abelian categories inside it. This notion was presented in (Beilinson, Bernstein & Deligne 1982).
The prototype is the t-structure on the derived category D of an abelian category A. For each n there are natural full subcategories
 and
and  consisting of complexes whose cohomology is "bounded below" or "bounded above" n, respectively. Since for any complex X, we have Hn(X) = H0(X[n]), these are related to each other:
consisting of complexes whose cohomology is "bounded below" or "bounded above" n, respectively. Since for any complex X, we have Hn(X) = H0(X[n]), these are related to each other:These subcategories also have the following properties:
 ,
, 

- Every object Y can be embedded in a distinguished triangle
 with
with  ,
, 
A t-structure on a triangulated category consists of full subcategories
 and
and  satisfying the conditions above. In Faisceaux pervers a triangulated category equipped with a t-structure is called a t-category.
satisfying the conditions above. In Faisceaux pervers a triangulated category equipped with a t-structure is called a t-category.The core or heart (the original French word is "coeur") of a t-structure is the category
 . It is an abelian category, whereas a triangulated category is additive but almost never abelian. The core of a t-structure on the derived category of A can be thought of as a sort of twisted version of A, which sometimes has better properties. For example, the category of perverse sheaves is the core of a certain (quite complicated) t-structure on the derived category of the category of sheaves. Over a space with singularities, the category of perverse sheaves is similar to the category of sheaves but behaves better.
. It is an abelian category, whereas a triangulated category is additive but almost never abelian. The core of a t-structure on the derived category of A can be thought of as a sort of twisted version of A, which sometimes has better properties. For example, the category of perverse sheaves is the core of a certain (quite complicated) t-structure on the derived category of the category of sheaves. Over a space with singularities, the category of perverse sheaves is similar to the category of sheaves but behaves better.A basic example of a t-structure is the "natural" one on the derived category D of some abelian category, where
 are the full subcategories of complexes whose cohomologies vanish in degrees less than or greater than 0. This t-structure has the following features:
are the full subcategories of complexes whose cohomologies vanish in degrees less than or greater than 0. This t-structure has the following features:- The truncation functors
 , or in fact
, or in fact  for any n, which are obtained by translating the argument of the original two functors. Abstractly, these are the left adjoint and right adjoint, respectively, to the inclusion functors of
for any n, which are obtained by translating the argument of the original two functors. Abstractly, these are the left adjoint and right adjoint, respectively, to the inclusion functors of  in D. In addition, the truncation functors fit into a triangle, and this is in fact the unique triangle satisfying the third axiom above:
in D. In addition, the truncation functors fit into a triangle, and this is in fact the unique triangle satisfying the third axiom above:
- The cohomology functor H0, or in fact Hn, which is obtained by translating its argument: Hn(A) = H0(A[n]). Its relationship to the truncation functors is that they are defined so that for any complex A,
 for i < 0 and is unchanged for
for i < 0 and is unchanged for  ; likewise for
; likewise for  ; in particular, H0 is not independent of them, but in fact
; in particular, H0 is not independent of them, but in fact  . Furthermore, the cohomology is a cohomological functor: for any triangle
. Furthermore, the cohomology is a cohomological functor: for any triangle  we obtain a long exact sequence
we obtain a long exact sequence
These properties carry over without change to any t-structure, in that if D is a t-category, then there exist truncation functors into its core, from which we obtain a cohomology functor taking values in the core, and the above properties are satisfied for both.
References
Part of Verdier's 1963 thesis is reprinted in "SGA 4 1/2" :
- Verdier, Jean-Louis, Catégories dérivées (état 0) in SGA 4½, http://modular.fas.harvard.edu/sga/sga/pdf/sga4h.pdf
and the entire thesis was published in Astérisque and is distributed by the American Mathematical Society in North America as
- Verdier, Jean-Louis (1963), "Des Catégories Dérivées des Catégories Abéliennes", Astérisque (Société Mathématique de France, Marseilles) 239, 1996
The material is also presented in English in
- Hartshorne, Robin (1966), "Chapter I. The Derived Category", Residues and Duality, Lecture Notes in Mathematics 20, Springer-Verlag, pp. 20–48
Axioms similar to Verdier's were presented in:
- Dold, A.; Puppe, D. (1961), "Homologie nicht-additiver Funktoren", Annales de l'Institut Fourier (Université de Grenoble) 11: 201–312, ISSN 0373-0956
Some textbooks that discuss triangulated categories are:
- Weibel, Charles A. (1994), An introduction to homological algebra, Cambridge Studies in Advanced Mathematics, 38, Cambridge University Press, ISBN 978-0-521-55987-4, OCLC 36131259, MR1269324
- Gelfand, S. I.; Manin, Yu. (2006), "IV. Triangulated Categories", Methods of Homological Algebra, Springer Monographs in Mathematics (2nd ed.), Springer-Verlag, ISBN 978-3540435839
- Neeman, A. (2001), Triangulated Categories, Annals of Mathematics Studies, Princeton University Press, ISBN 978-0691086866
The first section of the following paper discusses (but assumes familiarity with) the axioms of a triangulated category and introduces the notion of a t-structure:
- Beilinson, A.A.; Bernstein, J.; Deligne, P. (1982), "Faisceaux pervers" (in French), Astérisque (Société Mathématique de France, Paris) 100
Herein is a concise introduction with applications:
- Kashiwara, M.; Schapira, P. (2002), "Chapter I. Homological Algebra", Sheaves on Manifolds, Grundlehren der mathematischen Wissenschaften, Springer-Verlag, ISBN 978-3540518617
Categories:
Wikimedia Foundation. 2010.

![X \xrightarrow{u} Y \xrightarrow{v} Z \xrightarrow {w} X[1].](7/9e78568ffc9906fa378f0388f68f1bc8.png)


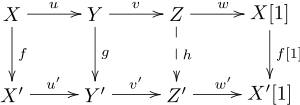


![\cdots \to X \xrightarrow{u} Y \xrightarrow{v} Z \xrightarrow{w} X[1] \xrightarrow{u[1]} \cdots,\](6/b06ad531b8fdae0c263f723eb2ca934f.png)
![\cdots \to F(X) \to F(Y) \to F(Z) \to F(X[1]) \to \cdots.\](b/07b5cd6c46be611e807c20a359dc591a.png)
![\cdots \to \operatorname{Hom}(A, X[i]) \to \operatorname{Hom}(A, Y[i]) \to \operatorname{Hom}(A, Z[i]) \to \operatorname{Hom}(A, X[i + 1]) \to \cdots.\](2/252f5a2721059fcb83d6fa9d68877b10.png)
![\operatorname{Ext}^i(A, X) = \operatorname{Hom}(A, X[i])](5/395c14c8994672fd436bde1d251f5df5.png)

![D^{\leq n} = D^{\leq 0}[-n], D^{\geq n} = D^{\geq 0}[-n].](5/615009e7bc991d13c5a1dba6b7e01d8c.png)

