Order-7 truncated triangular tiling

Order-7 truncated triangular tiling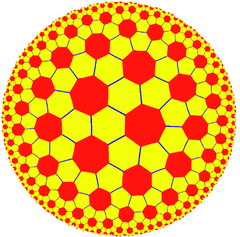
Poincaré_disk_model
Type	Hyperbolic semiregular tiling
Vertex figure	7.6.6
Schläfli symbol	t{3,7}
Wythoff symbol	2 7 | 3
Coxeter-Dynkin
Symmetry	[7,3]
Dual	Order-3 heptakis heptagonal tiling
Properties	Vertex-transitive

In geometry, the Order 7 truncated heptagonal tiling, sometimes called the hyperbolic soccerball, is a semiregular tiling of the hyperbolic plane. There are two hexagons and one heptagon on each vertex, forming a pattern similar to a conventional soccer ball (truncated icosahedron) with heptagons in place of pentagons. It has Schläfli symbol of t_1,2{7,3}.

Dual tiling

The dual tiling is called an order-3 heptakis heptagonal tiling, named for being constructible as an order-3 heptagonal tiling with every heptagon divided into seven triangles by the center point.

See also

• Triangular tiling
• Order-3 heptagonal tiling
• Order-7 triangular tiling
• Tilings of regular polygons
• List of uniform tilings

References

• John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, The Symmetries of Things 2008, ISBN 978-1-56881-220-5 (Chapter 19, The Hyperbolic Archimedean Tessellations)
• The Beauty of Geometry: Twelve Essays (1999), Dover Publications, LCCN 99-35678, ISBN 0-486-40919-8 (Chapter 10: Regular honeycombs in hyperbolic space)

External links

• Weisstein, Eric W., "Hyperbolic tiling" from MathWorld.
• Weisstein, Eric W., "Poincaré hyperbolic disk" from MathWorld.
• Hyperbolic and Spherical Tiling Gallery
• KaleidoTile 3: Educational software to create spherical, planar and hyperbolic tilings
• Hyperbolic Planar Tessellations, Don Hatch
• PDF with instructions
• The first paper hyperbolic soccerball
• A rather large hyperbolic soccerball

This geometry-related article is a stub. You can help Wikipedia by expanding it.v · Categories: Geometry stubs Tiling Wikimedia Foundation. 2010. Игры ⚽ Нужно сделать НИР? Biathlon World Championships 2007 - Men's Relay Sister Philippa Brazill Look at other dictionaries: Order-3 truncated heptagonal tiling — Poincaré disk model Type Hyperbolic semiregular tiling Vertex figure 3.14.14 Schläfli symbol t{7 …   Wikipedia Order-3 heptagonal tiling — Poincaré disk model of the hyperbolic plane Type Regular hyperbolic tiling Vertex figure 7.7.7 Schläfli symbol(s) …   Wikipedia Snub square tiling — In geometry, the snub square tiling is a semiregular tiling of the Euclidean plane. There are three triangles and two squares on each vertex. It has Schläfli symbol of s{4,4} .Conway calls it a snub quadrille, constructed as a snub operation… …   Wikipedia Bisected hexagonal tiling — Type Dual semiregular tiling Faces 30 60 90 triangle Face configuration V4.6.12 …   Wikipedia List of mathematics articles (O) — NOTOC O O minimal theory O Nan group O(n) Obelus Oberwolfach Prize Object of the mind Object theory Oblate spheroid Oblate spheroidal coordinates Oblique projection Oblique reflection Observability Observability Gramian Observable subgroup… …   Wikipedia Hyperbolic soccerball — The hyperbolic soccerball is a tiling of a surface frequently used as a manipulative for studying the properties of hyperbolic geometry. It is an embedding of the order 7 truncated triangular tiling into Euclidean space.DescriptionJust as… …   Wikipedia Icosaèdre tronqué — Type Solide d Archimède Faces Hexagones et Pentagones Éléments :  · Faces  · Arêt …   Wikipédia en Français Convex uniform honeycomb — The alternated cubic honeycomb is one of 28 space filling uniform tessellations in Euclidean 3 space, composed of alternating yellow tetrahedra and red octahedra. In geometry, a convex uniform honeycomb is a uniform tessellation which fills three …   Wikipedia Conway polyhedron notation — This example chart shows how 11 new forms can be derived from the cube using 3 operations. The new polyhedra are shown as maps on the surface of the cube so the topological changes are more apparent. Vertices are marked in all forms with circles …   Wikipedia List of mathematics articles (T) — NOTOC T T duality T group T group (mathematics) T integration T norm T norm fuzzy logics T schema T square (fractal) T symmetry T table T theory T.C. Mits T1 space Table of bases Table of Clebsch Gordan coefficients Table of divisors Table of Lie …   Wikipedia 18+ © Academic, 2000-2024 Contact us: Technical Support, Advertising Dictionaries export, created on PHP, Joomla, Drupal, WordPress, MODx. Mark and share Search through all dictionaries Translate… Search Internet Share the article and excerpts Direct link … Do a right-click on the link above and select “Copy Link”