- Magnetocrystalline anisotropy
-
Magnetocrystalline anisotropy is the dependence of the internal energy of a ferromagnet on the direction of its magnetization. As a result, certain crystallographic directions are preferred directions, or easy axes, for the magnetization. It is a special case of magnetic anisotropy. The spin-orbit interaction is the primary source of the magnetocrystalline anisotropy.
Magnetocrystalline anisotropy determines whether a magnetic material can be made into a good hard magnet, a good soft magnet or neither. Hard magnets are an essential component of electromagnetic motors and soft magnets are an essential component of transformers.
Contents
Microscopic origin
Magnetocrystalline anisotropy arises mostly from spin-orbit coupling.[1] This effect is weak compared to the exchange interaction and is difficult to compute from first principles, although some successful computations have been made.[2]
Thermodynamic theory
The magnetocrystalline anisotropy energy is generally represented as an expansion in powers of the direction cosines of the magnetization. The magnetization vector can be written M = Ms(α,β,γ), where Ms is the saturation magnetization. Because of time reversal symmetry, only even powers of the cosines are allowed.[3] The nonzero terms in the expansion depend on the crystal system (e.g., cubic or hexagonal).[3] The order of a term in the expansion is the sum of all the exponents of magnetization components, i.e., α β is second order.
Uniaxial anisotropy
More than one kind of crystal system has a single axis of high symmetry (threefold, fourfold or sixfold). The anisotropy of such crystals is called uniaxial anisotropy. If the z axis is taken to be the main symmetry axis of the crystal, the lowest order term in the energy is[4]
The ratio E/V is an energy density (energy per unit volume). This can also be represented in spherical polar coordinates with α = cos φ sin θ, β = sin φ sin θ, and γ = cos θ:
The parameter K1, often represented as Ku, has units of energy density and depends on composition and temperature.
The minima in this energy with respect to θ satisfy
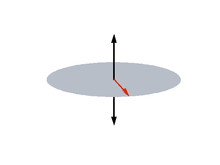
If K1 > 0, the directions of lowest energy are the ± z directions. The z axis is called the easy axis. If K1 < 0, there is an easy plane perpendicular to the symmetry axis (the basal plane of the crystal).
Many models of magnetization represent the anisotropy as uniaxial and ignore higher order terms. However, if K1 < 0, the lowest energy term does not determine the direction of the easy axes within the basal plane. For this, higher-order terms are needed, and these depend on the crystal system (hexagonal, tetragonal or rhombohedral).[3]
Hexagonal system
In a hexagonal system the c axis is an axis of sixfold rotation symmetry. The energy density is, to fourth order,
 .
.
The uniaxial anisotropy is mainly determined by the first two terms. Depending on the values K1 and K2, there are four different cases:[1]
- When K1 = K2 = 0, the ferromagnet is isotropic.
- When K1 > 0 and K2 ≥ 0, the c axis is an easy axis.
- When K1 < 0 and K2 ≤ 0, the basal plane is an easy plane.
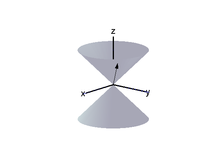
- When K1 and K2 have different signs, the ferromagnet has an easy cone (see figure to right).
The basal plane anisotropy is determined by the third term, which is sixth-order. The easy directions are projected onto three axes in the basal plane.
Below are some room-temperature anisotropy constants for hexagonal ferromagnets. Since all the values of K1 and K2 are positive, these materials have an easy axis.
Room-temperature anisotropy constants ( × 104 J/m3 ). [1] Structure K1 K2 Co 45 15 αFe2O3 (hematite) 120[6] BaO· 6Fe2O3 3 YCo5 550 MnBi 89 27 Tetragonal and Rhombohedral systems
The energy density for a tetragonal crystal is[3]
 .
.
Note that the K3 term, the one that determines the basal plane anisotropy, is fourth order (same as the K2 term). The definition of K3 may vary by a constant multiple between publications.
The energy density for a rhombohedral crystal is[3]
 .
.
Cubic anisotropy
In a cubic crystal the lowest order terms in the energy are[3][1]
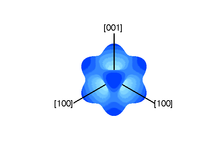
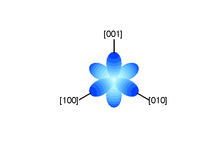
If the second term can be neglected, the easy axes are the < 100 > axes (i.e., the ± x, ± y, and ± z, directions) for K1 > 0 and the < 111 > directions for K1 < 0 (see images on right).
If K2 is not assumed to be zero, the easy axes depend on both K1 and K2. These are given in the table below, along with hard axes (directions of greatest energy) and intermediate axes (saddle points) in the energy). In energy surfaces like those on the right, the easy axes are analogous to valleys, the hard axes to peaks and the intermediate axes to mountain passes.
Easy axes for K1 > 0. [1] Type of axis  to − 9K1 / 4
to − 9K1 / 4K2 = − 9K1 / 4 to − 9K1 K2 = − 9K1 to 
Easy 


Medium 


Hard 


Easy axes for K1 < 0. [1] Type of axis  to − 9K1 / 4
to − 9K1 / 4K2 = − 9K1 / 4 to − 9K1 K2 = − 9K1 to 
Easy 


Medium 


Hard 


Below are some room-temperature anisotropy constants for cubic ferromagnets. The compounds involving Fe2O3 are ferrites, an important class of ferromagnets. In general the anisotropy parameters for cubic ferromagnets are higher than those for uniaxial ferromagnets. This is consistent with the fact that the lowest order term in the expression for cubic anisotropy is fourth order, while that for uniaxial anisotropy is second order.
Room-temperature anisotropy constants ( × 104 J/m3 ). [1] Structure K1 K2 Fe 4.8 
Ni − 0.5 − 0.2 FeO· Fe2O3 (magnetite) − 1.1 MnO· Fe2O3 − 0.3 NiO· Fe2O3 − 0.62 MgO· Fe2O3 − 0.25 CoO· Fe2O3 − 0.25 Temperature dependence of anisotropy
The magnetocrystalline anisotropy parameters have a strong dependence on temperature. They generally decrease rapidly as the temperature approaches the Curie temperature, so the crystal becomes effectively isotropic.[1] Some materials also have an isotropic point at which K1 = 0. Magnetite (Fe3O4), a mineral of great importance to rock magnetism and paleomagnetism, has an isotropic point at 130 kelvin.[6]
Magnetite also has a phase transition at which the crystal symmetry changes from cubic (above) to monoclinic or possibly triclinic below. The temperature at which this occurs, called the Verwey temperature, is 120 Kelvin.[6]
Magnetostriction
The magnetocrystalline anisotropy parameters are generally defined for ferromagnets that are constrained to remain undeformed as the direction of magnetization changes. However, coupling between the magnetization and the lattice does result in deformation, an effect called magnetostriction. To keep the lattice from deforming, a stress must be applied. If the crystal is not under stress, magnetostriction alters the effective magnetocrystalline anisotropy. If a ferromagnet is single domain (uniformly magnetized), the effect is to change the magnetocrystalline anisotropy parameters.[7]
In practice, the correction is generally not large. In hexagonal crystals, there is no change in K1.[8] In cubic crystals, there is a small change, as in the table below.
Room-temperature anisotropy constants K1 (zero-strain) and K1′ (zero-stress) ( × 104 J/m3 ). [8] Structure K1 K'1 Fe 4.7 4.7 Ni − 6.0 − 5.9 FeO· Fe2O3 (magnetite) − 1.1 − 1.4 Notes
- ^ a b c d e f g h Cullity & Graham 2005
- ^ Daalderop, Kelly & Schuurmans 1990
- ^ a b c d e f Landau, Lifshitz & Pitaevski 2004
- ^ An arbitrary constant term is ignored.
- ^ The lowest-order term in the energy can be written in more than one way because, by definition, α2+β2+γ2 = 1.
- ^ a b c Dunlop & Özdemir 1997
- ^ Chikazumi 1997, chapter 12
- ^ a b Ye, Newell & Merrill 1994
References
- Chikazumi, Sōshin (1997). Physics of Ferromagnetism. Clarendon Press. ISBN 0-19-851776-9.
- Cullity, B.D.; Graham, C.D. (2005). Introduction to Magnetic Materials. John Wiley. ISBN 0201012189.
- Daalderop, G. H. O.; Kelly, P. J.; Schuurmans, M. F. H. (1990). "First-principles calculation of the magnetocrystalline anisotropy energy of iron, cobalt, and nickel". Phys. Rev. B 41 (17): 11919–11937. Bibcode 1990PhRvB..4111919D. doi:10.1103/PhysRevB.41.11919.
- Dunlop, David J.; Özdemir, Özden (1997). Rock Magnetism: Fundamentals and Frontiers. Cambridge Univ. Press. ISBN 0-521-32514-5.
- Landau, L. D.; Lifshitz, E. M.; Pitaevski, L. P. (2004) [First published in 1960]. Electrodynamics of Continuous Media. Course of Theoretical Physics. 8 (Second ed.). Elsevier. ISBN 0750626348.
- Ye, Jun; Newell, Andrew J.; Merrill, Ronald T. (1994). "A re-evaluation of magnetocrystalline anisotropy and magnetostriction constants". Geophysical Research Letters 21 (1): 25–28. Bibcode 1994GeoRL..21...25Y. doi:10.1029/93GL03263.
Categories:- Magnetic ordering
Wikimedia Foundation. 2010.