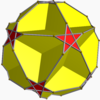
Dodecadodecahedron

Dodecadodecahedron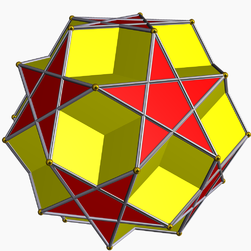
Type	Uniform star polyhedron
Elements	F = 24, E = 60 V = 30 (χ = −6)
Faces by sides	12{5}+12{5/2}
Wythoff symbol	2 | 5 5/2
Symmetry group	Ih, [5,3], *532
Index references	U36, C45, W73
5.5/2.5.5/2 (Vertex figure)	Medial rhombic triacontahedron (dual polyhedron)

In geometry, the dodecadodecahedron is a nonconvex uniform polyhedron, indexed as U₃₆. It is given a Schläfli symbol t₁{5/2,5}.

The Dodecadodecahedron has 30 vertices, 60 edges, and 24 faces (12{5}+12{⁵/₂}). The vertex configuration is 5.⁵/₂.5.⁵/₂. Its symmetry group is I_h, [5,3], *532, its Wythoff symbol is 2 | 5 ⁵/₂, and its Euler characteristic is χ=−6.

Its uniform index number is U36, its Kaleido index is K41, its number in Wenninger's Polyhedron Models is 73, and it was given the number 45 in Coxeter's 1954 paper, which first gave the complete list of the uniform polyhedra.

Net

A shape with the same exterior appearance as the dodecadodecahedron can be constructed by folding up these nets:

12 pentagrams and 20 rhombic clusters are necessary. However, this construction replaces the crossing pentagonal faces of the dodecadodecahedron with non-crossing sets of rhombs, so it does not produce the same internal structure.

Related polyhedra

Its convex hull is the icosidodecahedron. It also shares its edge arrangement with the small dodecahemicosahedron (having the pentagrammic faces in common), and with the great dodecahemicosahedron (having the pentagonal faces in common).

Dodecadodecahedron	Small dodecahemicosahedron	Great dodecahemicosahedron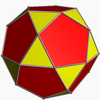
Icosidodecahedron (convex hull)

This polyhedron can be considered a rectified great dodecahedron. It is center of a truncation sequence between a small stellated dodecahedron and great dodecahedron:

The truncated small stellated dodecahedron looks like a dodecahedron on the surface, but it has 24 faces: 12 pentagons from the truncated vertices and 12 overlapping as (truncated pentagrams). The truncation of the dodecadodecahedron itself is not uniform, but it has a uniform quasitruncation, the truncated dodecadodecahedron.

Name	Small stellated dodecahedron	Truncated small stellated dodecahedron	Dodecadodecahedron	Truncated great dodecahedron	Great dodecahedron
Coxeter-Dynkin diagram
Picture

It is topologically equivalent to a quotient space of the hyperbolic order-4 pentagonal tiling, by distorting the pentagrams back into regular pentagons. As such, it is topologically a regular polyhedron of index two:^[1][2]

The colours in the above image correspond to the red pentagrams and yellow pentagons of the dodecadodecahedron at the top of this article.

See also

• List of uniform polyhedra

References

1. ^ The Regular Polyhedra (of index two), David A. Richter
2. ^ The Golay Code on the Dodecadodecahedron, David A. Richter

External links

• Weisstein, Eric W., "Dodecadodecahedron" from MathWorld.
• Uniform polyhedra and duals

This polyhedron-related article is a stub. You can help Wikipedia by expanding it.v · Categories: Polyhedron stubs Uniform polyhedra Wikimedia Foundation. 2010. Игры ⚽ Нужна курсовая? Great cubicuboctahedron Great icosidodecahedron Look at other dictionaries: dodecadodecahedron — noun /ˌdəʊdɛkəˈˌdəʊdɛkəˈhiːdrən,ˌdəʊdɪkəˈˌdəʊdɪkəˈhiːdrən,ˌdoʊdɛkəˈˌdoʊdɛkəˈhiːdrən/ A nonconvex polyhedron with twenty four faces; thirty vertices and sixty edges. See Also: dodecadodecahedral, dodecahedron, icosidodecadodecahedron …   Wiktionary Snub dodecadodecahedron — Type Uniform star polyhedron Elements F = 84, E = 150 V = 60 (χ = −6) Faces by sides 60{3}+12{5}+12{5/2} Wythoff symbol …   Wikipedia Ditrigonal dodecadodecahedron — Type Uniform star polyhedron Elements F = 24, E = 60 V = 20 (χ = −16) Faces by sides 12{5}+12{5/2} Wy …   Wikipedia Inverted snub dodecadodecahedron — Type Uniform star polyhedron Elements F = 84, E = 150 V = 60 (χ = −6) Faces by sides 60{3}+12{5}+12{5/2} …   Wikipedia Icositruncated dodecadodecahedron — In geometry, the icositruncated dodecadodecahedron is a nonconvex uniform polyhedron, indexed as U45. Cartesian coordinates Cartesian coordinates for the vertices of an icositruncated dodecadodecahedron are all the even permutations of: (±(2−1/τ) …   Wikipedia Truncated dodecadodecahedron — In geometry, the truncated dodecadodecahedron is a nonconvex uniform polyhedron, indexed as U59. Cartesian coordinates Cartesian coordinates for the vertices of a truncated dodecadodecahedron are all the even permutations of: (±1, ±1, ±3): (±1/τ …   Wikipedia Liste Des Polyèdres Uniformes — Les polyèdres uniformes et les pavages forment un groupe bien étudié. Ils sont listés ici pour une comparaison rapide de leurs propriétés et de leurs noms de schéma variés ainsi que de leurs symboles. Cette liste inclut : tous les 75… …   Wikipédia en Français Liste des polyedres uniformes — Liste des polyèdres uniformes Les polyèdres uniformes et les pavages forment un groupe bien étudié. Ils sont listés ici pour une comparaison rapide de leurs propriétés et de leurs noms de schéma variés ainsi que de leurs symboles. Cette liste… …   Wikipédia en Français Liste des polyèdres uniformes — Cette liste recense les polyèdres uniformes, ainsi que certaines de leurs propriétés. Sommaire 1 Méthodologie 2 Table des polyèdres 2.1 Formes convexes (3 faces/sommet) …   Wikipédia en Français Uniform star polyhedron — A display of uniform polyhedra at the Science Museum in London …   Wikipedia 18+ © Academic, 2000-2024 Contact us: Technical Support, Advertising Dictionaries export, created on PHP, Joomla, Drupal, WordPress, MODx. Mark and share Search through all dictionaries Translate… Search Internet Share the article and excerpts Direct link … Do a right-click on the link above and select “Copy Link”