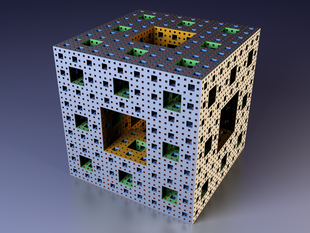
Menger sponge

An illustration of M₄, the fourth iteration of the construction process.

In mathematics, the Menger sponge is a fractal curve. It is a universal curve, in that it has topological dimension one, and any other curve (more precisely: any compact metric space of topological dimension 1) is homeomorphic to some subset of it. It is sometimes called the Menger-Sierpinski sponge or the Sierpinski sponge. It is a three-dimensional extension of the Cantor set and Sierpinski carpet. It was first described by Karl Menger (1926) while exploring the concept of topological dimension.

The Menger sponge simultaneously exhibits an infinite surface area and encloses zero volume.

Construction

The construction of a Menger sponge can be described as follows:

1. Begin with a cube (first image).
2. Divide every face of the cube into 9 squares, like a Rubik's Cube. This will sub-divide the cube into 27 smaller cubes.
3. Remove the smaller cube in the middle of each face, and remove the smaller cube in the very center of the larger cube, leaving 20 smaller cubes (second image). This is a level-1 Menger sponge (resembling a Void Cube).
4. Repeat steps 2 and 3 for each of the remaining smaller cubes, and continue to iterate ad infinitum.

The second iteration will give you a level-2 sponge (third image), the third iteration gives a level-3 sponge (fourth image), and so on. The Menger sponge itself is the limit of this process after an infinite number of iterations.

An illustration of the iterative construction of a Menger sponge up to M₃, the third iteration.

The number of cubes is 20ⁿ, with n being the number of iterations performed on the first cube.

A sculptural representation of the previous illustration.

Properties

Each face of the Menger sponge is a Sierpinski carpet; furthermore, any intersection of the Menger sponge with a diagonal or medium of the initial cube M₀ is a Cantor set.

The Menger sponge is a closed set; since it is also bounded, the Heine–Borel theorem implies that it is compact. It has Lebesgue measure 0. It is an uncountable set.

The topological dimension of the Menger sponge is one, the same as any curve. Menger showed, in the 1926 construction, that the sponge is a universal curve, in that any possible one-dimensional curve is homeomorphic to a subset of the Menger sponge, where here a curve means any compact metric space of Lebesgue covering dimension one; this includes trees and graphs with an arbitrary countable number of edges, vertices and closed loops, connected in arbitrary ways. In a similar way, the Sierpinski carpet is a universal curve for all curves that can be drawn on the two-dimensional plane. The Menger sponge constructed in three dimensions extends this idea to graphs that are not planar, and might be embedded in any number of dimensions.

The Menger sponge simultaneously exhibits an infinite surface area and encloses zero volume. In spite of this, there exists a homeomorphism of the cube having finite distortion that "squeezes the sponge" in the sense that the holes in the sponge go to a Cantor set of zero measure (Iwaniec & Martin 2001, §6.5.6).

The sponge has a Hausdorff dimension of (log 20) / (log 3) (approximately 2.726833).

Formal definition

Formally, a Menger sponge can be defined as follows:

$M := \bigcap_{n\in\mathbb{N}} M_n$

where M₀ is the unit cube and

$M_{n+1} := \left\{\begin{matrix} (x,y,z)\in\mathbb{R}^3: & \begin{matrix}\exists i,j,k\in\{0,1,2\}: (3x-i,3y-j,3z-k)\in M_n \\ \mbox{and at most one of }i,j,k\mbox{ is equal to 1}\end{matrix} \end{matrix}\right\}.$

See also

• List of fractals by Hausdorff dimension
• Sierpiński triangle
• Sierpiński tetrahedron
• Koch snowflake
• Apollonian gasket

References

• Iwaniec, Tadeusz; Martin, Gaven (2001), Geometric function theory and non-linear analysis, Oxford Mathematical Monographs, The Clarendon Press Oxford University Press, ISBN 978-0-19-850929-5, MR1859913 .
• Menger, Karl (1926), "Allgemeine Räume und Cartesische Räume. I.", Communications to the Amsterdam Academy of Sciences  English translation reprinted in Edgar, Gerald A., ed. (2004), Classics on fractals, Studies in Nonlinearity, Westview Press. Advanced Book Program, Boulder, CO, ISBN 978-0-8133-4153-8, MR2049443 
• Karl Menger, Dimensionstheorie, (1928) B.G Teubner Publishers, Leipzig.
• Zhou, Li (2007), "Problem 11208: Chromatic numbers of the Menger sponges", American Mathematical Monthly 114 (9): 842 

External links

• Menger sponge at Wolfram MathWorld
• An interactive Menger sponge
• Fractal polyhedra (VRML) and interactive Java models
• Puzzle Hunt — Video explaining Zeno's paradoxes using Menger-Sierpinski sponge
• The Business Card Menger Sponge Project
• Menger Sponge Assembly Construction of a Level-3 Menger Sponge from business cards
• Menger Sponge Animations — Menger sponge animations up to level 9, discussion of optimization for 3d.
• L3 Menger Sponge with business cards 2006 – An L3 Menger sponge by students at Cornell College built in 2006
• Level 3 Menger Sponge made of Business Cards – A level-3 Menger sponge built by students at Mississippi State University out of 48,000 folded business cards.
• Menger sphere, rendered in SunFlow
• Post-It Menger Sponge – a level 3 Menger sponge being built from Post-its
• The Mystery of the Menger Sponge. Sliced diagonally to reveal stars.