- Compound of five cubes
-
Compound of five cubes 
Type Regular compound Stellation core rhombic triacontahedron Convex hull Dodecahedron Index UC9 Polyhedra 5 cubes Faces 30 squares Edges 60 Vertices 20 Dual Compound of five octahedra Symmetry group icosahedral (Ih) Subgroup restricting to one constituent pyritohedral (Th) 
As a stellation
This polyhedral compound is a symmetric arrangement of five cubes. This compound was first described by Edmund Hess in 1876.
It is one of five regular compounds, and dual to the compound of five octahedra.
It is one of the stellations of the rhombic triacontahedron. It has icosahedral symmetry (Ih).
Its convex hull is a regular dodecahedron. It additionally shares its edge arrangement with the small ditrigonal icosidodecahedron, the great ditrigonal icosidodecahedron, and the ditrigonal dodecadodecahedron.
Small ditrigonal icosidodecahedron
Great ditrigonal icosidodecahedron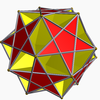
Ditrigonal dodecadodecahedron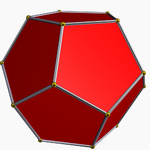
Dodecahedron (convex hull)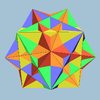
Compound of five cubesThe compound of ten tetrahedra can be formed by taking each of these five cubes and replacing them with the two tetrahedra of the Stella octangula (which share the same vertex arrangement of a cube).
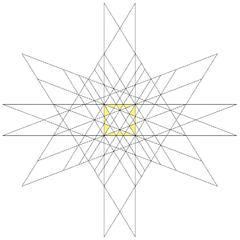
As a stellation
This compound can be formed as a stellation of the rhombic triacontahedron. The 30 rhombic faces exist in the planes of the 5 cubes.
The stellation facets for construction are:
As a facetting
Five cubes in a dodecahedronIt is also a faceting of a dodecahedron, shown at left, and is also a faceting of the icosidodecahedron.
External links
- MathWorld: Cube 5-Compound
- George Hart: Compounds of Cubes
- Steven Dutch: Uniform Polyhedra and Their Duals
- VRML model: [1]
This polyhedron-related article is a stub. You can help Wikipedia by expanding it.
