Critical path method

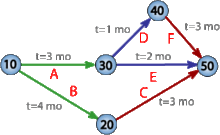PERT chart for a project with five milestones (10 through 50) and six activities (A through F). The project has two critical paths: activities B and C, or A, D, and F – giving a minimum project time of 7 months with fast tracking. Activity E is sub-critical, and has a float of 2 months.

The critical path method (CPM) is an algorithm for scheduling a set of project activities.^[1] It is an important tool for effective project management.

History

The critical path method (CPM) is a project modeling technique developed in the late 1950s by Morgan R. Walker of DuPont and James E. Kelley, Jr. of Remington Rand.^[2] Kelley and Walker related their memories of the development of CPM in 1989.^[3] Kelley attributed the term "critical path" to the developers of the Program Evaluation and Review Technique which was developed at about the same time by Booz Allen Hamilton and the US Navy.^[4] The precursors of what came to be known as Critical Path were developed and put into practice by DuPont between 1940 and 1943 and contributed to the success of the Manhattan Project.^[5]

CPM is commonly used with all forms of projects, including construction, aerospace and defense, software development, research projects, product development, engineering, and plant maintenance, among others. Any project with interdependent activities can apply this method of mathematical analysis. Although the original CPM program and approach is no longer used, the term is generally applied to any approach used to analyze a project network logic diagram.

Basic technique

The essential technique for using CPM ^[6] is to construct a model of the project that includes the following:

1. A list of all activities required to complete the project (typically categorized within a work breakdown structure),
2. The time (duration) that each activity will take to completion, and
3. The dependencies between the activities.

Using these values, CPM calculates the longest path of planned activities to the end of the project, and the earliest and latest that each activity can start and finish without making the project longer. This process determines which activities are "critical" (i.e., on the longest path) and which have "total float" (i.e., can be delayed without making the project longer). In project management, a critical path is the sequence of project network activities which add up to the longest overall duration. This determines the shortest time possible to complete the project. Any delay of an activity on the critical path directly impacts the planned project completion date (i.e. there is no float on the critical path). A project can have several, parallel, near critical paths. An additional parallel path through the network with the total durations shorter than the critical path is called a sub-critical or non-critical path.

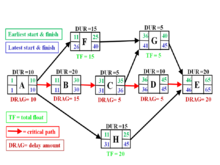
Although the activity-on-arrow diagram ("PERT Chart") is still used in a few places, it has generally been superseded by the activity-on-node diagram, where each activity is shown as a box or node and the arrows represent the logical relationships going from predecessor to successor as shown in the diagram Activity-on-node diagram showing critical path schedule, along with total float and critical path drag computations.

Activity-on-node diagram showing critical path schedule, along with total float and critical path drag computations

In this diagram, Activities A, B, C, D, and E comprise the critical or longest path, while Activities F, G, and H are off the critical path with floats of 10 days, 5 days, and 20 days respectively. Whereas activities that are off the critical path have float and are therefore not delaying completion of the project, those on the critical path will usually have critical path drag 7, i.e., they delay project completion. The drag of a critical path activity can be computed using the following formula:

1. If a critical path activity has nothing in parallel, its drag is equal to its duration. Thus A and E have drags of 10 days and 20 days respectively. 2. If a critical path activity has another activity in parallel, its drag is equal to whichever is less: its duration or the total float of the parallel activity with the least total float. Thus since B and C are both parallel to F (float of 15) and H (float of 20), B has a duration of 20 and drag of 15 (equal to F's float), while C has a duration of only 5 days and thus drag of only 5. Activity D, with a duration of 10 days, is parallel to G (float of 5) and H (float of 20) and therefore its drag is equal to 5, the float of G.

These results, including the drag computations, allow managers to prioritize activities for the effective management of project completion, and to shorten the planned critical path of a project by pruning critical path activities, by "fast tracking" (i.e., performing more activities in parallel), and/or by "crashing the critical path" (i.e., shortening the durations of critical path activities by adding resources).

Crash Duration

"Crash duration" is a term referring to the shortest possible time for which an activity can be scheduled.^[7] It is achieved by shifting more resources towards the completion of that activity, resulting in decreased time spent and often a reduced quality of work, as the premium is set on speed.^[8] Crash duration is typically modeled as a linear relationship between cost and activity duration, however in many cases a convex function or a step function is more applicable.^[9]

Expansion

Originally, the critical path method considered only logical dependencies between terminal elements. Since then, it has been expanded to allow for the inclusion of resources related to each activity, through processes called activity-based resource assignments and resource leveling. A resource-leveled schedule may include delays due to resource bottlenecks (i.e., unavailability of a resource at the required time), and may cause a previously shorter path to become the longest or most "resource critical" path. A related concept is called the critical chain, which attempts to protect activity and project durations from unforeseen delays due to resource constraints.

Since project schedules change on a regular basis, CPM allows continuous monitoring of the schedule, allows the project manager to track the critical activities, and alerts the project manager to the possibility that non-critical activities may be delayed beyond their total float, thus creating a new critical path and delaying project completion. In addition, the method can easily incorporate the concepts of stochastic predictions, using the Program Evaluation and Review Technique (PERT) and event chain methodology.

Currently, there are several software solutions available in industry that use the CPM method of scheduling, see list of project management software. The method currently used by most project management software is based on a manual calculation approach developed by Fondahl of Stanford University.

Flexibility

A schedule generated using critical path techniques often is not realised precisely, as estimations are used to calculate times: if one mistake is made, the results of the analysis may change. This could cause an upset in the implementation of a project if the estimates are blindly believed, and if changes are not addressed promptly. However, the structure of critical path analysis is such that the variance from the original schedule caused by any change can be measured, and its impact either ameliorated or adjusted for. Indeed, an important element of project postmortem analysis is the As Built Critical Path (ABCP), which analyzes the specific causes and impacts of changes between the planned schedule and eventual schedule as actually implemented.

See also

• Gantt chart
• List of project management software
• List of project management topics
• Program Evaluation and Review Technique (PERT)
• Project
• Project management
• Project planning
• Work breakdown structure

References

1. ^ Kelley, James. Critical Path Planning and Scheduling: Mathematical Basis. Operations Research, Vol. 9, No. 3, May–June, 1961. 
2. ^ Kelley, James; Walker, Morgan. Critical-Path Planning and Scheduling. 1959 Proceedings of the Eastern Joint Computer Conference. 
3. ^ Kelley, James; Walker, Morgan. The Origins of CPM: A Personal History. PMNETwork 3(2):7-22.. 
4. ^ Newell, M; Grashina, M (2003). The Project Management Question and Answer Book. American Management Association. p. 98. 
5. ^ Thayer, Harry (1996). Management of the Hanford Engineer Works in World War II, How the Corps, DuPont and the Metallurgical Laboratory fast tracked the original plutonium works. ASCE Press, pp. 66-67. 
6. ^ Samuel L. Baker, Ph.D. "Critical Path Method (CPM)" University of South Carolina, Health Services Policy and Management Courses
7. ^ Hendrickson, Chris. "11. Advanced Scheduling Techniques". Project Management for Construction. http://pmbook.ce.cmu.edu/11_Advanced_Scheduling_Techniques.html. Retrieved 27 October 2011. 
8. ^ Brooks, F.P. (1975). The Mythical Man-Hour. Reading, MA: Addison Wesley. 
9. ^ Hendrickson, C.; B.N. Janson (1984). "A Common Network Flow Formulation for Several Civil Engineering Problems". Civil Engineering Systems. 4 1: 195–203. 

Further reading

• Project Management Institute (2003). A Guide To The Project Management Body Of Knowledge (3rd ed.). Project Management Institute. ISBN 1-930699-45-X. 
• Klastorin, Ted (2003). Project Management: Tools and Trade-offs (3rd ed.). Wiley. ISBN 978-0471413844. 
• Heerkens, Gary (2001). Project Management (The Briefcase Book Series). McGraw–Hill. ISBN 0-07-137952-5. 
• Kerzner, Harold (2003). Project Management: A Systems Approach to Planning, Scheduling, and Controlling (8th ed.). ISBN 0-471-22577-0. 
• Lewis, James (2002). Fundamentals of Project Management (2nd ed.). American Management Association. ISBN 0-8144-7132-3. 
• Milosevic, Dragan Z. (2003). Project Management ToolBox: Tools and Techniques for the Practicing Project Manager. Wiley. ISBN 978-0471208228. 
• O'Brien, James J.; Plotnick, Fredric L. (2010). CPM in Construction Management, Seventh Edition. McGraw Hill. ISBN 978-0-07-163664-3. 
• Woolf, Murray B. (2007). Faster Construction Projects with CPM Scheduling. McGraw Hill. ISBN 978-0071486606. 

External links

• Critical path web calculator
• A Few Critical Path Articles
• A slide show explaining critical path concepts
• Critical Path Java Applet
• [1]