- Zero morphism
-
In category theory, a zero morphism is a special kind of morphism exhibiting properties like those to and from a zero object.
Suppose C is a category, and f : X → Y is a morphism in C. The morphism f is called a constant morphism (or sometimes left zero morphism) if for any object W in C and any g, h : W → X, fg = fh. Dually, f is called a coconstant morphism (or sometimes right zero morphism) if for any object Z in C and any g, h ∈ MorC(Y, Z), gf = hf. A zero morphism is one that is both a constant morphism and a coconstant morphism.
If C has a zero object 0, given two objects X and Y in C, there are canonical morphisms f : 0 → X and g : Y → 0. Then, fg is a zero morphism in MorC(Y, X).
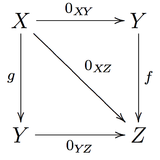
A category with zero morphisms is one where, for any two objects A and B in C, there is a fixed morphism 0AB : A → B such that for all objects X, Y, Z in C and all morphisms f : Y → Z, g : X → Y, the following diagram commutes:
The morphisms 0XY are forced to be zero morphisms and form a compatible system of such. If C is a category with zero morphisms, then the collection of 0XY is unique. A category with a zero object is a category with zero morphisms (given by the composition 0XY : X → 0 → Y described above).
If a category has zero morphisms, then one can define the notions of kernel and cokernel for any morphism in that category.
Examples
- In the category of groups (or of modules), a zero morphism is a homomorphism f : G → H that maps all of G to the identity element of H. The null object in the category of groups is the trivial group 1 = {1}, which is unique up to isomorphism. Every zero morphism can be factored through 1, i. e., f : G → 1 → H.
- More generally, suppose C is any category with a zero object 0. Then for all objects X and Y there is a unique sequence of morphisms
-
- 0XY : X → 0 → Y
- The family of all morphisms so constructed endows C with the structure of a category with zero morphisms.
- If C is a preadditive category, then every morphism set Mor(X,Y) is an abelian group and therefore has a zero element. These zero elements form a compatible family of zero morphisms for C making it into a category with zero morphisms.
- The category Set (sets with functions as morphisms) does not have a zero object, but it does have an initial object, the empty set ∅. The only zero morphisms in Set are the functions ∅ → X for a set X.
References
- Section 1.7 of Pareigis, Bodo (1970), Categories and functors, Pure and applied mathematics, 39, Academic Press, ISBN 978-0-12-545150-5
- Herrlich, Horst; Strecker, George E. (1973), Category Theory, Allen and Bacon, Inc. Boston.
Categories:- Morphisms
- Zero
Wikimedia Foundation. 2010.