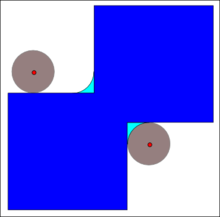
Closing (morphology)

The closing of the dark-blue shape (union of two squares) by a disk, resulting in the union of the dark-blue shape and the light-blue areas.

In mathematical morphology, the closing of a set (binary image) A by a structuring element B is the erosion of the dilation of that set,

$A\bullet B = (A\oplus B)\ominus B, \,$

where $\oplus$ and $\ominus$ denote the dilation and erosion, respectively.

In image processing, closing is, together with opening, the basic workhorse of morphological noise removal. Opening removes small objects, while closing removes small holes.

Properties

• It is idempotent, that is, $(A\bullet B)\bullet B=A\bullet B$.
• It is increasing, that is, if $A\subseteq C$, then $A\bullet B \subseteq C\bullet B$.
• It is extensive, i.e., $A\subseteq A\bullet B$.
• It is translation invariant.

See also

• Mathematical morphology
• Dilaton
• Erosion
• Opening
• Top-hat transformation

Bibliography

• Image Analysis and Mathematical Morphology by Jean Serra, ISBN 0126372403 (1982)
• Image Analysis and Mathematical Morphology, Volume 2: Theoretical Advances by Jean Serra, ISBN 0-12-637241-1 (1988)
• An Introduction to Morphological Image Processing by Edward R. Dougherty, ISBN 0-8194-0845-X (1992)

External links

• Introduction to mathematical morphology

This applied mathematics-related article is a stub. You can help Wikipedia by expanding it.v · Categories: Applied mathematics stubs Mathematical morphology Digital geometry Wikimedia Foundation. 2010. Игры ⚽ Нужно сделать НИР? CIRC Abdul Rasul Sayyaf Look at other dictionaries: Closing — may refer to: The closing of a letter or e mail: see Valediction or Complimentary close The termination or closure of a business Closing (real estate), the final step in executing a real estate transaction Closing (sales), making a sale Closing… …   Wikipedia Closing — (im deutschen auch Schließen) ist eine morphologische Basis Operation in der digitalen Bildverarbeitung. Anwendung findet der Operator beim Filtern von Bildern; durch das Schließen lassen sich lokal begrenzte dunkle Störungen in einem Bild… …   Deutsch Wikipedia Mathematical morphology — A shape (in blue) and its morphological dilation (in green) and erosion (in yellow) by a diamond shape structuring element. Mathematical morphology (MM) is a theory and technique for the analysis and processing of geometrical structures, based on …   Wikipedia Opening (morphology) — The opening of the dark blue square by a disk, resulting in the light blue square with round corners. In mathematical morphology, opening is the dilation of the erosion of a set A by a structuring element B: where …   Wikipedia Glossary of plant morphology terms — Biologists that study plant morphology use a number of different terms to describe plant organs and parts that can be observed with the human eye using no more than a hand held magnifying lens. These terms are used to identify and classify plants …   Wikipedia Dilation (morphology) — Dilation is one of the basic operations in mathematical morphology. Originally developed for binary images, it has been expanded first to grayscale images, and then to complete lattices. The dilation operation usually uses a structuring element… …   Wikipedia Erosion (morphology) — Erosion is one of two fundamental operations (the other being dilation) in Morphological image processing from which all other morphological operations are based. It was originally defined for binary images, later being extended to grayscale… …   Wikipedia List of mathematics articles (C) — NOTOC C C closed subgroup C minimal theory C normal subgroup C number C semiring C space C symmetry C* algebra C0 semigroup CA group Cabal (set theory) Cabibbo Kobayashi Maskawa matrix Cabinet projection Cable knot Cabri Geometry Cabtaxi number… …   Wikipedia PROSODY, HEBREW — This article is a survey of the history of Hebrew poetic forms from the Bible to the present time. The entry is arranged according to the following outline: introduction the variety of formal systems the specific nature of hebrew literary history …   Encyclopedia of Judaism On the Origin of Species — Origin of Species redirects here. For other uses, see Origin of Species (disambiguation). On the Origin of Species   …   Wikipedia 18+ © Academic, 2000-2024 Contact us: Technical Support, Advertising Dictionaries export, created on PHP, Joomla, Drupal, WordPress, MODx. Mark and share Search through all dictionaries Translate… Search Internet Share the article and excerpts Direct link … Do a right-click on the link above and select “Copy Link”