Dilation (morphology)

Dilation is one of the basic operations in mathematical morphology. Originally developed for binary images, it has been expanded first to grayscale images, and then to complete lattices. The dilation operation usually uses a structuring element for probing and expanding the shapes contained in the input image.

Binary Operator

The dilation of the dark-blue square by a disk, resulting in the light-blue square with rounded corners.

In binary morphology, dilation is a shift-invariant (translation invariant) operator, strongly related to the Minkowski addition.

A binary image is viewed in mathematical morphology as a subset of an Euclidean space R^d or the integer grid Z^d, for some dimension d. Let E be a Euclidean space or an integer grid, A a binary image in E, and B a structuring element.

The dilation of A by B is defined by:

$A \oplus B = \bigcup_{b\in B} A_b$.

The dilation is commutative, also given by: $A \oplus B = B\oplus A = \bigcup_{a\in A} B_a$.

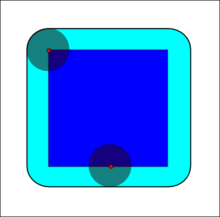
If B has a center on the origin, then the dilation of A by B can be understood as the locus of the points covered by B when the center of B moves inside A. The dilation of a square of side 10, centered at the origin, by a disk of radius 2, also centered at the origin, is a square of side 14, with rounded corners, centered at the origin. The radius of the rounded corners is 2.

The dilation can also be obtained by: $A \oplus B = \{z \in E| (B^{s})_{z} \cap A\neq \varnothing\}$, where B^s denotes the symmetric of B, that is, $B^{s}=\{x\in E | -x \in B\}$.

Properties of binary dilation

Here are some properties of the binary dilation operator:

• It is translation invariant.
• It is increasing, that is, if $A\subseteq C$, then $A\oplus B \subseteq C\oplus B$.
• It is commutative.
• If the origin of E belongs to the structuring element B, then it is extensive, i.e., $A\subseteq A\oplus B$.
• It is associative, i.e., $(A\oplus B)\oplus C = A\oplus (B\oplus C)$.
• It is distributive over set union

Grayscale dilation

In grayscale morphology, images are functions mapping a Euclidean space or grid E into $\mathbb{R}\cup\{\infty,-\infty\}$, where $\mathbb{R}$ is the set of reals, $\infty$ is an element larger than any real number, and $-\infty$ is an element smaller than any real number.

Grayscale structuring elements are also functions of the same format, called "structuring functions".

Denoting an image by f(x) and the structuring function by b(x), the grayscale dilation of f by b is given by

$(f\oplus b)(x)=\sup_{y\in E}[f(y)+b(x-y)]$,

where "sup" denotes the supremum.

Flat structuring functions

It is common to use flat structuring elements in morphological applications. Flat structuring functions are functions b(x) in the form

$b(x)=\left\{\begin{array}{ll}0,&x\in B,\\-\infty,&\mbox{otherwise}\end{array}\right.$,

where $B\subseteq E$.

In this case, the dilation is greatly simplified, and given by

$(f\oplus b)(x)=\sup_{y\in E}[f(y)+b(x-y)]=\sup_{z\in E}[f(x-z)+b(z)]=\sup_{z\in B}f(x-z)=\sup_{z\in B^{s}}f(x+z)$.

In the bounded, discrete case (E is a grid and B is bounded), the supremum operator can be replaced by the maximum. Thus, dilation is a particular case of order statistics filters, returning the maximum value within a moving window (the symmetric of the structuring function support B).

Dilation on complete lattices

Complete lattices are partially ordered sets, where every subset has an infimum and a supremum. In particular, it contains a least element and a greatest element (also denoted "universe").

Let $(L,\leq)$ be a complete lattice, with infimum and minimum symbolized by $\wedge$ and $\vee$, respectively. Its universe and least element are symbolized by U and $\varnothing$, respectively. Moreover, let {X_i} be a collection of elements from L.

A dilation is any operator $\delta: L\rightarrow L$ that distributes over the supremum, and preserves the least element. I.e.:

• $\bigvee_{i}\delta(X_i)=\delta\left(\bigvee_{i} X_i\right)$,
• $\delta(\varnothing)=\varnothing$.

See also

• Mathematical morphology
• Closing
• Erosion
• Opening

Bibliography

• Image Analysis and Mathematical Morphology by Jean Serra, ISBN 0126372403 (1982)
• Image Analysis and Mathematical Morphology, Volume 2: Theoretical Advances by Jean Serra, ISBN 0-12-637241-1 (1988)
• An Introduction to Morphological Image Processing by Edward R. Dougherty, ISBN 0-8194-0845-X (1992)