Domain of holomorphy

The sets in the definition.

In mathematics, in the theory of functions of several complex variables, a domain of holomorphy is a set which is maximal in the sense that there exists a holomorphic function on this set which cannot be extended to a bigger set.

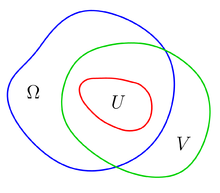
Formally, an open set Ω in the n-dimensional complex space ${\mathbb{C}}^n$ is called a domain of holomorphy if there do not exist non-empty open sets $U \subset \Omega$ and $V \subset {\mathbb{C}}^n$ where V is connected, $V \not\subset \Omega$ and $U \subset \Omega \cap V$ such that for every holomorphic function f on Ω there exists a holomorphic function g on V with f = g on U

In the n = 1 case, every open set is a domain of holomorphy: we can define a holomorphic function with zeros accumulating everywhere on the boundary of the domain, which must then be a natural boundary for a domain of definition of its inverse. For $n \geq 2$ this is no longer true, as it follows from Hartogs' lemma.

Equivalent conditions

For a domain Ω the following conditions are equivalent:

1. Ω is a domain of holomorphy
2. Ω is holomorphically convex
3. Ω is pseudoconvex
4. Ω is Levi convex - for every sequence $S_{n} \subseteq \Omega$ of analytic compact surfaces such that $S_{n} \rightarrow S, \partial S_{n} \rightarrow \Gamma$ for some set Γ we have $S \subseteq \Omega$ ($\partial \Omega$ cannot be "touched from inside" by a sequence of analytic surfaces)
5. Ω has local Levi property - for every point $x \in \partial \Omega$ there exist a neighbourhood U of x and f holomorphic on $U \cap \Omega$ such that f cannot be extended to any neighbourhood of x

Implications $1 \Leftrightarrow 2, 3 \Leftrightarrow 4, 1 \Rightarrow 4, 3 \Rightarrow 5$ are standard results. The main difficulty lies in proving $5 \Rightarrow 1$, i.e. constructing a global holomoprhic function which admits no extension from non-extendable functions defined only locally. This is called the Levi problem and was first solved by Kiyoshi Oka, and then by Lars Hörmander using methods from functional analysis and partial differential equations (a consequence of -problem).

Properties

• if Ω_n are domains of holomorphy, then their intersection $\Omega = \bigcap_{n=1}^{\infty} \Omega_{n}$ is also a domain of holomorphy
• if $\Omega_{1} \subseteq \Omega_{2} \subseteq \dots$ is an ascending sequence of domains of holomorphy, then their union $\Omega = \bigcup_{n=1}^{\infty}\Omega_{n}$ is also a domain of holomorphy (see Behnke-Stein theorem)
• product $\Omega = \Omega_{1} \times \Omega_{2}$ of domains of holomorphy Ω₁,Ω₂ is a domain of holomorphy
• the first Cousin problem is always solvable in a domain of holomorphy; this is also true, with additional topological assumptions, for the second Cousin problem

References

• Steven G. Krantz. Function Theory of Several Complex Variables, AMS Chelsea Publishing, Providence, Rhode Island, 1992.
• Boris Vladimirovich Shabat, Introduction to Complex Analysis, AMS, 1992

See also

• Behnke–Stein theorem
• Levi pseudoconvex
• solution of the Levi problem

---

This article incorporates material from Domain of holomorphy on PlanetMath, which is licensed under the Creative Commons Attribution/Share-Alike License.