- Orthodiagonal quadrilateral
-
In Euclidean geometry, an orthodiagonal quadrilateral is a quadrilateral in which the diagonals cross at right angles. In other words, it is a four-sided figure in which the line segments between non-adjacent vertices are orthogonal to each other.
Contents
Special cases
A kite is an orthodiagonal quadrilateral in which one diagonal is a line of symmetry. The kites are exactly the orthodiagonal quadrilaterals that contain a circle tangent to all four of their sides; that is, the kites are the tangential orthodiagonal quadrilaterals.[1]
A rhombus is an orthodiagonal quadrilateral with two pairs of parallel sides (that is, an orthodiagonal quadrilateral that is also a parallelogram).
A square is a limiting case of both a kite and a rhombus.
Characterization
For any orthodiagonal quadrilateral, the sum of the squares of two opposite sides equals that of the other two opposite sides: for successive sides a, b, c, and d, we have [2]:p.136, #272;[3]
This follows from the Pythagorean theorem, by which either of these two sums of two squares can be expanded to equal the sum of the four squared distances from the quadrilateral's vertices to the point where the diagonals cross. Conversely, any quadrilateral in which a2 + c2 = b2 + d2 must be orthodiagonal.[4]
Area
The area K of an orthodiagonal quadrilateral equals one half the product of the lengths of the diagonals p, q:[5]
The orthodiagonal quadrilateral has the biggest area of all convex quadrilaterals with given diagonals.
Other properties
- Orthodiagonal quadrilaterals are the only quadrilaterals for which the sides and the angle formed by the diagonals do not uniquely determine the area.[3] For example, two rhombi both having common side a (and of course both having a right angle between the diagonals), but one having a smaller acute angle than the other, have different areas (the area of the former approaching zero as the acute angle approaches zero).
- In an orthodiagonal quadrilateral the two lines joining the midpoints of the pairs of opposite sides are of equal length.[2]:p.136
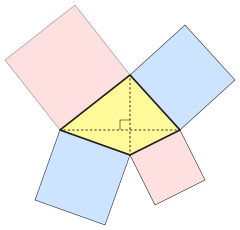
- If squares are erected outward on the sides of a convex quadrilateral, then their centres (centroids) are the vertices of an orthodiagonal quadrilateral with diagonals of equal length.
- The quadrilateral formed by the midpoints of the sides of an orthodiagonal quadrilateral is a rectangle.
Properties of orthodiagonal quadrilaterals that are also cyclic
- For a cyclic orthodiagonal quadrilateral (one that can be inscribed in a circle), suppose the intersection of the diagonals divides one diagonal into segments of lengths p1 and p2 and divides the other diagonal into segments of lengths q1 and q2. Then[6]
- where D is the diameter of the circumcircle. This holds because the diagonals are perpendicular chords of a circle. Equivalently, letting R = D / 2 be the radius of the circumcircle, the average of


 and
and  is R2. Moreover, the equations a2 + c2 = b2 + d2 = D2 imply that in an orthodiagonal cyclic quadrilateral, the sum of the squares of the sides equals eight times the square of the circumradius.
is R2. Moreover, the equations a2 + c2 = b2 + d2 = D2 imply that in an orthodiagonal cyclic quadrilateral, the sum of the squares of the sides equals eight times the square of the circumradius.
- Brahmagupta's theorem states that for a cyclic orthodiagonal quadrilateral, the perpendicular from any side through the point of intersection of the diagonals bisects the other side.[2]:p.137
- If an orthodiagonal quadrilateral is also cyclic, the distance from the circumcenter (the center of the circumscribed circle) to any side equals half the length of the opposite side.[2]:p.138
- If an orthodiagonal quadrilateral is also cyclic, then the midpoints of the sides and the feet of the perpendiculars from these midpoints to the opposite sides lie on a circle centered at the centroid of the quadrilateral. This circle is called the eight point circle.
See also
- Bicentric quadrilateral
- Brahmagupta's theorem
- Cyclic quadrilateral
- Ex-tangential quadrilateral
- Kite
- Rhombus
- Tangential quadrilateral
References
- ^ Josefsson, Martin (2010), "Calculations concerning the tangent lengths and tangency chords of a tangential quadrilateral", Forum Geometricorum 10: 119–130, http://forumgeom.fau.edu/FG2010volume10/FG201013.pdf.
- ^ a b c d Altshiller-Court, N. (2007), College Geometry, Dover Publications. Republication of second edition, 1952, Barnes & Noble.
- ^ a b Mitchell, Douglas, W. (2009), "The area of a quadrilateral", Mathematical Gazette 93: 306–309.
- ^ Ismailescu, Dan; Vojdany, Adam (2009), "Class preserving dissections of convex quadrilaterals", Forum Geometricorum 9: 195–211, http://forumgeom.fau.edu/FG2009volume9/FG200919.pdf.
- ^ Harries, J. "Area of a quadrilateral," Mathematical Gazette 86, July 2002, 310–311.
- ^ Posamentier, Alfred S., and Charles T. Salkind, Challenging Problems in Geometry, Dover Publ., second edition, 1996:pp. 104–105, #4–23.
Categories:- Quadrilaterals
Wikimedia Foundation. 2010.