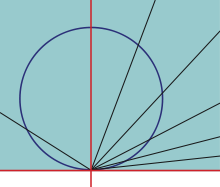
Conical combination

Given a finite number of vectors $x_1, x_2, \dots, x_n\,$ in a real vector space, a conical combination or a conical sum ^{[1] [2]} of these vectors is a vector of the form

$\alpha_1x_1+\alpha_2x_2+\cdots+\alpha_nx_n$

where the real numbers $\alpha_i\,$ satisfy $\alpha_i\ge 0.$

The name derives from the fact that a conical sum of vectors defines a cone (possibly in a lower dimensional subspace).

Conical hull

The set of all conical combinations for a given set S is called the conical hull of S and denoted cone (S),^[1] or coni (S)^[2], that is,

$\operatorname{coni} (S)=\Bigl\{\sum_{i=1}^k \alpha_i x_i \;\Big|\; x_i\in S, \, \alpha_i\in \mathbb{R}, \, \alpha_i\geq 0, i, k=1, 2, \dots\Bigr\}.$

By definition, the zero point (origin) belongs to all conical hulls.

The conical hull of a set S is a convex set. In fact, it is the intersection of all convex cones containing S plus the origin.^[1] If S is a compact set (in particular, when it is a finite set of points), then the condition "plus the origin" is unnecessary.

If we discard the origin, we can divide all coefficients by their sum to see that a conical combination is a convex combination scaled by a positive factor.

In the plane, the conical hull of a circle passing through the origin is the open half-plane defined by the tangent line to the circle at the origin plus the origin.

Therefore, the "conical combination" and "conical hull" are more accurately to be called the "convex conical combination" and "convex conical hull" respectively.^[1] Moreover, the above remark about dividing the coefficients while discarding the origin implies that the conical combinations and hulls may be considered as convex combinations and convex hulls in the projective space.

While the convex hull of a compact set is a compact set as well, this is not so for the conical hull: first of all, the latter one is unbounded. Moreover, it is even not necessarily a closed set: a counterexample is a sphere passing through the origin, with the conical hull being an open half-space plus the origin. However if S is a nonempty compact set which does not contain the origin, the conical hull is a closed set.^[1]

See also

Related combinations

For more details on this topic, see Linear combination#Affine, conical, and convex combinations.

• Affine combination
• Convex combination
• Linear combination

References

1. ^ ^{a b c d e} Convex Analysis and Minimization Algorithms by Jean-Baptiste Hiriart-Urruty, Claude Lemaréchal, 1993, ISBN 3540568506, pp. 101, 102
2. ^ ^{a b} Mathematical Programming, by Melvyn W. Jeter (1986) ISBN 0824774787, p. 68