Unit sphere

some unit spheres

In mathematics, a unit sphere is the set of points of distance 1 from a fixed central point, where a generalized concept of distance may be used; a closed unit ball is the set of points of distance less than or equal to 1 from a fixed central point. Usually a specific point has been distinguished as the origin of the space under study and it is understood that a unit sphere or unit ball is centered at that point. Therefore one speaks of "the" unit ball or "the" unit sphere.

For example, a one-dimensional sphere is the surface of what is commonly called a "circle", while such a circle's interior and surface together are the two-dimensional ball. Similarly, a two-dimensional sphere is the surface of the Euclidean solid known colloquially as a "sphere", while the interior and surface together are the three-dimensional ball.

A unit sphere is simply a sphere of radius one. The importance of the unit sphere is that any sphere can be transformed to a unit sphere by a combination of translation and scaling. In this way the properties of spheres in general can be reduced to the study of the unit sphere.

Unit spheres and balls in Euclidean space

In Euclidean space of n dimensions, the unit sphere is the set of all points $(x_1, \ldots, x_n)$ which satisfy the equation

$x_1^2 + x_2^2 + \cdots + x_n ^2 = 1$

and the closed unit ball is the set of all points satisfying the inequality

$x_1^2 + x_2^2 + \cdots + x_n ^2 \le 1.$

General area and volume formulas

The classical equation of a unit sphere is that of the ellipsoid with a radius of 1 and no alterations to the x-, y-, or z- axes:

f(x,y,z) = x² + y² + z² = 1

The volume of the unit ball in n-dimensional Euclidean space, and the surface area of the unit sphere, appear in many important formulas of analysis. The volume of the unit ball in n dimensions, which we denote V_n, can be expressed by making use of the Gamma function. It is

$V_n = \frac{\pi ^ {n/2}}{\Gamma(1+n/2)} = \begin{cases} {\pi^{n/2}}/{(n/2)!} & \mathrm{if~}n \ge 0\mathrm{~is~even,} \\ ~\\ {\pi^{\lfloor n/2 \rfloor}2^{\lceil n/2 \rceil}}/{n!!} & \mathrm{if~}n \ge 0\mathrm{~is~odd,} \end{cases}$

where n!! is the double factorial.

The hypervolume of the (n–1)-dimensional unit sphere (i.e., the "area" of the surface of the n-dimensional unit ball), which we denote A_n, can be expressed as

$A_n = n V_n = \frac{n \pi ^ {n/2}}{\Gamma(1+n/2)} = \frac{2 \pi ^ {n/2}}{\Gamma(n/2)}\,,$

where the last equality holds only for n > 0.

The surface areas and the volumes for some values of n are as follows:

n	An (surface area)		Vn (volume)
0	0(1 / 0!)π0	0.000	(1 / 0!)π0	1.000
1	1(21 / 1!!)π0	2.000	(21 / 1!!)π0	2.000
2	2(1 / 1!)π1 = 2π	6.283	(1 / 1!)π1 = π	3.142
3	3(22 / 3!!)π1 = 4π	12.57	(22 / 3!!)π1 = (4 / 3)π	4.189
4	4(1 / 2!)π2 = 2π2	19.74	(1 / 2!)π2 = (1 / 2)π2	4.935
5	5(23 / 5!!)π2 = (8 / 3)π2	26.32	(23 / 5!!)π2 = (8 / 15)π2	5.264
6	6(1 / 3!)π3 = π3	31.01	(1 / 3!)π3 = (1 / 6)π3	5.168
7	7(24 / 7!!)π3 = (16 / 15)π3	33.07	(24 / 7!!)π3 = (16 / 105)π3	4.725
8	8(1 / 4!)π4 = (1 / 3)π4	32.47	(1 / 4!)π4 = (1 / 24)π4	4.059
9	9(25 / 9!!)π4 = (32 / 105)π4	29.69	(25 / 9!!)π4 = (32 / 945)π4	3.299
10	10(1 / 5!)π5 = (1 / 12)π5	25.50	(1 / 5!)π5 = (1 / 120)π5	2.550

where the decimal expanded values for n ≥ 2 are rounded to the displayed precision.

Recursion

The A_n values satisfy the recursion:

A₀ = 0

A₁ = 2

A₂ = 2π

$A_n = \frac{2 \pi}{n-2} A_{n-2}$ for n > 2.

The V_n values satisfy the recursion:

V₀ = 1

V₁ = 2

$V_n = \frac{2 \pi}{n} V_{n-2}$ for n > 1.

Fractional dimensions

Main article: Hausdorff measure

The formulae for A_n and V_n can be computed for any real number n ≥ 0, and there are circumstances under which it is appropriate to seek the sphere area or ball volume when n is not a non-negative integer.

This shows the hypervolume of an (x–1)-dimensional sphere (i.e., the "area" of the surface of the x-dimensional unit ball) as a continuous function of x.

This shows the volume of a ball in x dimensions as a continuous function of x.

Other radii

Main article: Sphere

The surface area of an (n–1)-dimensional sphere with radius r is A_n rⁿ⁻¹ and the volume of an n-dimensional ball with radius r is V_n rⁿ. For instance, the area is A = 4π r² for the surface of the three-dimensional ball of radius r. The volume is V = 4π r³ / 3 for the three-dimensional ball of radius r.

Unit balls in normed vector spaces

More precisely, the open unit ball in a normed vector space V, with the norm $\|\cdot\|$, is

$\{ x\in V: \|x\|<1 \}.$

It is the interior of the closed unit ball of (V,||·||),

$\{ x\in V: \|x\|\le 1\}.$

The latter is the disjoint union of the former and their common border, the unit sphere of (V,||·||),

$\{ x\in V: \|x\| = 1 \}.$

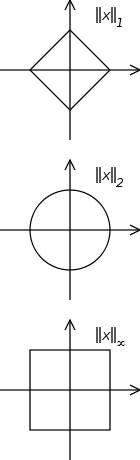
The 'shape' of the unit ball is entirely dependent on the chosen norm; it may well have 'corners', and for example may look like [−1,1]ⁿ, in the case of the norm l_∞ in Rⁿ. The round ball is understood as the usual Hilbert space norm, based in the finite dimensional case on the Euclidean distance; its boundary is what is usually meant by the unit sphere. Here are some images of the unit ball for the two-dimensional $\ell^p$ space for various values of p (the unit ball being concave for p < 1 and convex for p ≥ 1):

These illustrate why the condition p ≥ 1 is necessary in the definition of the $\ell^p$ norm, as the unit ball in any normed space must be convex as a consequence of the triangle inequality.

Note that for the circumferences C_p of the two-dimensional unit balls we have:

$C_{0} = C_{\infty} = 8$ is the maximum value.

$C_{1} = 4 \sqrt{2}$ is the minimum value.

$C_{2} = 2 \pi \,.$

Generalizations

Metric spaces

All three of the above definitions can be straightforwardly generalized to a metric space, with respect to a chosen origin. However, topological considerations (interior, closure, border) need not apply in the same way (e.g., in ultrametric spaces, all of the three are simultaneously open and closed sets), and the unit sphere may even be empty in some metric spaces.

Quadratic forms

If V is a linear space with a real quadratic form F:V → R, then { p ∈ V : F(p) = 1 } is sometimes called the unit quasi-sphere of V. For example, the quadratic form x² − y² corresponds to a unit quasi-sphere that is a hyperbola which plays the role of the "unit circle" in the plane of split-complex numbers. Similarly, quadratic form x² yields a pair of lines for the unit quasi-sphere in the dual number plane.

See also

• ball (mathematics)
• sphere
• Table of mathematical symbols
• unit circle
• unit disk
• unit sphere bundle
• unit square

References

• Mahlon M. Day (1958) Normed Linear Spaces, page 24, Springer-Verlag.
• Deza, E.; Deza, M. (2006), Dictionary of Distances, Elsevier, ISBN 0444520872 . Reviewed in Newsletter of the European Mathematical Society 64 (June 2007), p. 57. This book is organized as a list of distances of many types, each with a brief description.

External links

• Weisstein, Eric W., "Unit sphere" from MathWorld.