Mean width

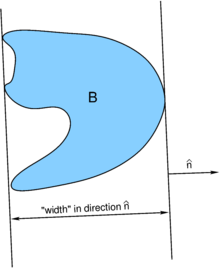
In geometry, the mean width is a measure of dimension length of the "size" a body; see Hadwiger's theorem for more about the available measures of bodies. In n dimensions, one has to consider (n − 1)-dimensional hyperplanes perpendicular to a given direction $\hat{n}$ in S^{n − 1}, where Sⁿ is the n-sphere (the surface of a (n + 1)-dimensional sphere). The "width" of a body in a given direction $\hat{n}$ is the distance between the closest pair of such planes, such that the body is entirely in between the two hyper planes (the planes only intersect with the boundary of the body). The mean width is the average of this "width" over all $\hat{n}$ in S^{n − 1}.

The definition of the "width" of body B in direction $\hat{n}$ in 2 dimensions.

More formally, define a compact body B as being equivalent to set of points in its interior plus the points on the boundary (here, points denote elements of $\mathbb{R}^n$). The support function of body B is defined as

$h_B(v)=\max\{ \langle n,x\rangle |x \in B \}$

where n is a direction and $\langle,\rangle$ denotes the usual inner product on $\mathbb{R}^n$. The mean width is then

$b(B)=\frac{1}{S_{n-1}} \int_{S^{n-1}} h_B(\hat{n})+h_B(-\hat{n}),$

where S_{n − 1} is the (n − 1)-dimensional volume of S^{n − 1}. Note, that the mean width can be defined for any body (that is compact), but it is most useful for convex bodies (that is bodies, whose corresponding set is a convex set).

The relation between mean width and mean curvature

For convex bodies K in three dimensions, the mean width of K is related to the average of the mean curvature, H, over the whole surface of K. In fact,

$\int_{\delta K} \frac{H}{2\pi} dS = b(K)$

where δK is the boundary of the convex body K and dS a surface integral element, H is the mean curvature at the corresponding position on δK. Similar relations can be given between the other measures and the generalizations of the mean curvature, also for other dimensions .^[1] As the integral over the mean curvature is typically much easier to calculate than the mean width, this is a very useful result.

Reference material

The mean width is usually mentioned in any good reference on convex geometry, for instance, Selected topics in convex geometry by Maria Moszyńska (Birkhäuser, Boston 2006). The relation between the mean width and the mean curvature is also derived in that reference.

The application of the mean width as one of the measures featuring in Hadwiger's theorem is discussed in Beifang Chen in "A simplified elementary proof of Hadwiger's volume theorem." Geom. Dedicata 105 (2004), 107—120.

Cited references

1. ^ Jiazu, Zhou; Deshuo, Jiang (2008), "On mean curvatures of a parallel convex body", Acta Mathematica Scientia 28 (3): 489–494, doi:10.1016/S0252-9602(08)60050-8