- Cup product
-
In mathematics, specifically in algebraic topology, the cup product is a method of adjoining two cocycles of degree p and q to form a composite cocycle of degree p + q. This defines an associative (and distributive) graded commutative product operation in cohomology, turning the cohomology of a space X into a graded ring, H∗(X), called the cohomology ring. The cup product was introduced in work of J. W. Alexander, Eduard Čech and Hassler Whitney from 1935–1938, and, in full generality, by Samuel Eilenberg in 1944.
Contents
Definition
In singular cohomology, the cup product is a construction giving a product on the graded cohomology ring H∗(X) of a topological space X.
The construction starts with a product of cochains: if cp is a p-cochain and dq is a q-cochain, then
where σ is a (p + q) -singular simplex and
 is the canonical embedding of the simplex spanned by S into the (p + q)-standard simplex.
is the canonical embedding of the simplex spanned by S into the (p + q)-standard simplex.Informally,
 is the p-th front face and
is the p-th front face and  is the q-th back face of σ, respectively.
is the q-th back face of σ, respectively.The coboundary of the cup product of cocycles cp and dq is given by
The cup product of two cocycles is again a cocycle, and the product of a coboundary with a cocycle (in either order) is a coboundary. Thus, the cup product operation passes to cohomology, defining a bilinear operation
Properties
The cup product operation in cohomology satisfies the identity
so that the corresponding multiplication is graded-commutative.
The cup product is functorial, in the following sense: if
is a continuous function, and
is the induced homomorphism in cohomology, then
for all classes α, β in H *(Y). In other words, f * is a (graded) ring homomorphism.
Interpretation
It is possible to view the cup product
 as induced from the following composition:
as induced from the following composition:
in terms of the chain complexes of X and
 , where the first map is the Künneth map and the second is the map induced by the diagonal
, where the first map is the Künneth map and the second is the map induced by the diagonal  .
.This composition passes to the quotient to give a well-defined map in terms of cohomology, this is the cup product. This approach explains the existence of a cup product for cohomology but not for homology:
 induces a map
induces a map  but would also induce a map
but would also induce a map  , which goes the wrong way round to allow us to define a product. This is however of use in defining the cap product.
, which goes the wrong way round to allow us to define a product. This is however of use in defining the cap product.Bilinearity follows from this presentation of cup product, i.e.
 and
and 
Examples
As singular spaces, the 2-sphere S2 with two disjoint 1-dimensional loops attached by their endpoints to the surface and the torus T have identical cohomology groups in all dimensions, but the multiplication of the cup product distinguishes the associated cohomology rings. In the former case the multiplication of the cochains associated to the loops is degenerate, whereas in the latter case multiplication in the first cohomology group can be used to decompose the torus as a 2-cell diagram, thus having product equal to Z (more generally M where this is the base module).
Other definitions
Cup product and differential forms
In de Rham cohomology, the cup product of differential forms is also known as the wedge product, and in this sense is a special case of Grassmann's exterior product.
Cup product and geometric intersections
 The linking number can be defined in terms of a non-vanishing cup product on the complement of a link. The complement of these two linked circles deformation retracts to a torus, which has a non-vanishing cup product.
The linking number can be defined in terms of a non-vanishing cup product on the complement of a link. The complement of these two linked circles deformation retracts to a torus, which has a non-vanishing cup product.
When two submanifolds of a smooth manifold intersect transversely, their intersection is again a submanifold. By taking the fundamental homology class of these manifolds, this yields a bilinear product on homology. This product is dual to the cup product, i.e. the homology class of the intersection of two submanifolds is the Poincaré dual of the cup product of their Poincaré duals.
Similarly, the linking number can be defined in terms of intersections, shifting dimensions by 1, or alternatively in terms of a non-vanishing cup product on the complement of a link.
Massey products
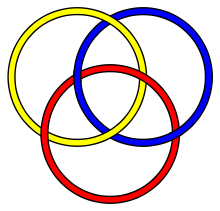 Massey products generalize cup product, allowing one to define "higher order linking numbers", the Milnor invariants.
Massey products generalize cup product, allowing one to define "higher order linking numbers", the Milnor invariants.
The cup product is a binary (2-ary) operation; one can define a ternary (3-ary) and higher order operation called the Massey product, which generalizes the cup product. This is a higher order cohomology operation, which is only partly defined (only defined for some triples).
See also
References
- James R. Munkres, "Elements of Algebraic Topology", Perseus Publishing, Cambridge Massachusetts (1984) ISBN 0-201-04586-9 (hardcover) ISBN 0-201-62778-0 (paperback)
- Glen E. Bredon, "Topology and Geometry", Springer-Verlag, New York (1993) ISBN 0-387-97926-3
- Allen Hatcher, "Algebraic Topology", Cambridge Publishing Company (2002) ISBN 0-521-79540-0
Categories:- Homology theory
- Algebraic topology
- Binary operations
Wikimedia Foundation. 2010.







