Conchoid of Dürer

Conchoid of Dürer
Conchoid of Dürer, constructed by him

The conchoid of Dürer, also called Dürer's shell curve, is a variant of a conchoid or plane algebraic curve. It is not a true conchoid.

Contents

Construction

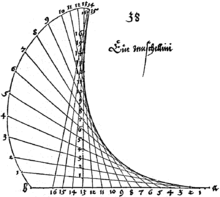
Let Q and R be points moving on a pair of perpendicular lines which intersect at O in such a way that OQ + OR is constant. On any line QR mark point P at a fixed distance from Q. The locus of the points P is Dürer's conchoid.

Equation

The equation of the conchoid in Cartesian form is

2y^2(x^2+y^2) - 2by^2(x+y) + (b^2-3a^2)y^2 - a^2x^2 + 2a^2b(x+y) + a^2(a^2-b^2) = 0 . \,

Properties

The curve has two components, asymptotic to the lines y = \pm a / \sqrt2. Each component is a rational curve. If a>b there is a loop, if a=b there is a cusp at (0,a).

Special cases include:

  • a=0: the line y=0;
  • b=0: the line pair y = \pm x / \sqrt2 together with the circle x2 + y2 = a2;


History

It was first described by the German painter and mathematician Albrecht Dürer (1471–1528) in his book Underweysung der Messung (S. 38), calling it Ein muschellini.

See also

References

  • J. Dennis Lawrence (1972). A catalog of special plane curves. Dover Publications. pp. 157–159. ISBN 0-486-60288-5. 
Stub icon This geometry-related article is a stub. You can help Wikipedia by expanding it.