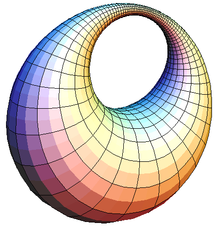
Dupin cyclide

A Dupin cyclide

In mathematics, a Dupin cyclide or cyclide of Dupin is any geometric inversion of any standard torus. In particular, the standard (or circular) tori are themselves examples of Dupin cyclides. They were discovered by (and named after) Charles Dupin in his 1803 dissertation under Gaspard Monge.^[1] The key property of a Dupin cyclide is that it is a channel surface (envelope of a one parameter family of spheres) in two different ways. This property means that Dupin cyclides are natural objects in Lie sphere geometry.

Dupin cyclides are often simply known as "cyclides", but the latter term is also used to refer to a more general class of quartic surfaces which are important in the theory of separation of variables for the Laplace equation in three dimensions.

Definitions and properties

There are several equivalent definitions of Dupin cyclides, of which, there are several main characteristics of them. The definition as geometric inversions of standard tori shows that the class of Dupin cyclides is invariant under Möbius (or conformal) transformations. Since a standard torus is an orbit of a two dimensional abelian subgroup of the Euclidean group, it follows that the cyclides are orbits of two dimensional abelian subgroups of the group of Möbius transformations, and this provides a second way to define them.

A third property which characterizes Dupin cyclides is the fact that their curvature lines are all circles (possibly through the point at infinity). Equivalently, the curvature spheres, which are the spheres tangent to the surface with radii equal to the reciprocals of the principal curvatures at the point of tangency, are constant along the corresponding curvature lines: they are the tangent spheres containing the corresponding curvature lines as great circles. Equivalently again, both sheets of the focal surface degenerate to conics.^[2] It follows that any Dupin cyclide is a channel surface (i.e., the envelope of a one parameter family of spheres) in two different ways, and this gives another characterization.

The definition in terms of spheres shows that the class of Dupin cyclides is invariant under the larger group of all Lie sphere transformations. In fact any two Dupin cyclides are Lie equivalent. They form (in some sense) the simplest class of Lie invariant surfaces after the spheres, and are therefore particularly significant in Lie sphere geometry.^[3]

The definition also means that a Dupin cyclide is the envelope of the one parameter family of spheres tangent to three given mutually tangent spheres. It follows that it is tangent to infinitely many Soddy's hexlet configurations of spheres.

Cyclides and separation of variables

Dupin cyclides are a special case of a more general notion of a cyclide, which is a natural extension of the notion of a quadric surface. Whereas a quadric can be described as the zero-set of second order polynomial in Cartesian coordinates (x₁,x₂,x₃), a cyclide is given by the zero-set of a second order polynomial in (x₁,x₂,x₃,r²), where r²=x₁²+x₂²+x₃². Thus it is a quartic surface in Cartesian coordinates, with an equation of the form:

$A r^4 + \sum_{i=1}^3 P_i x_i r^2 + \sum_{i,j=1}^3 Q_{ij} x_i x_j + \sum_{i=1}^3 R_i x_i + B = 0$

where Q is a 3x3 matrix, P and R are a 3-dimensional vectors, and A and B are constants.^[4]

Families of cyclides give rise to various cyclidic coordinate geometries.

In Maxime Bôcher's 1891 dissertation, Ueber die Reihenentwickelungen der Potentialtheorie, it was shown that the Laplace equation in three variables can be solved using separation of variables in 17 conformally distinct quadric and cyclidic coordinate geometries. Many other cyclidic geometries can be obtained by studying R-separation of variables for the Laplace equation.^[5]

Notes

1. ^ O'Connor & Robertson 2000
2. ^ Hilbert & Cohn-Vossen 1999
3. ^ Cecil 1992
4. ^ Miller 1977
5. ^ Moon & Spencer 1961

References

• Cecil, Thomas E. (1992), Lie sphere geometry, New York: Universitext, Springer-Verlag, ISBN 978-0387977478 .
• Eisenhart, Luther P. (1960), "§133 Cyclides of Dupin", A Treatise on the Differential Geometry of Curves and Surfaces, New York: Dover, pp. 312–314 .
• Hilbert, David; Cohn-Vossen, Stephan (1999), Geometry and the Imagination, American Mathematical Society, ISBN 0-8218-1998-4 .
• Moon, Parry; Spencer, Domina Eberle (1961), Field Theory Handbook: including coordinate systems, differential equations, and their solutions, Springer, ISBN 0-3870-2732-7 .
• O'Connor, John J.; Robertson, Edmund F. (2000), "Pierre Charles François Dupin", MacTutor History of Mathematics archive, http://www-groups.dcs.st-and.ac.uk/~history/Biographies/Dupin.html .
• Pinkall, Ulrich (1986), "§3.3 Cyclides of Dupin", in G. Fischer, Mathematical Models from the Collections of Universities and Museums, Braunschweig, Germany: Vieweg, pp. 28–30 .
• Miller, Willard (1977), Symmetry and Separation of Variables .

External links

• Weisstein, Eric W., "Cyclide" from MathWorld.
• "Javaview of Dupin Cycloid". http://www.javaview.de/demo/surface/common/PaSurface_DupinCycloid.html.