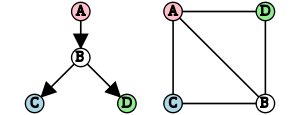
Comparability graph

In graph theory, a comparability graph is an undirected graph that connects pairs of elements that are comparable to each other in a partial order. Comparability graphs have also been called transitively orientable graphs, partially orderable graphs, and containment graphs.^[1]

Definitions and characterization

Hasse diagram of a poset (left) and its comparability graph (right)

One of the forbidden induced subgraphs of a comparability graph. The generalized cycle a–b–d–f–d–c–e–c–b–a in this graph has odd length (nine) but has no triangular chords.

For any strict partially ordered set (S,<), the comparability graph of (S, <) is the graph (S, ⊥) of which the vertices are the elements of S and the edges are those pairs {u, v} of elements such that u < v. That is, for a partially ordered set, take the directed acyclic graph, apply transitive closure, and remove orientation.

Equivalently, a comparability graph is a graph that has a transitive orientation,^[2] an assignment of directions to the edges of the graph such that the adjacency relation of the resulting directed graph is transitive: whenever there exist directed edges (x,y) and (y,z), there must exist an edge (x,z).

One can represent any partial order as a family of sets, such that x < y in the partial order whenever the set corresponding to x is a subset of the set corresponding to y. In this way, comparability graphs can be shown to be equivalent to containment graphs of set families; that is, a graph with a vertex for each set in the family and an edge between two sets whenever one is a subset of the other.^[3]

Alternatively^[4], a comparability graph is a graph such that, for every generalized cycle of odd length, one can find an edge (x,y) connecting two vertices that are at distance two in the cycle. Such an edge is called a triangular chord. In this context, a generalized cycle is defined to be a closed walk that uses each edge of the graph at most once in each direction.

Comparability graphs can also be characterized by a list of forbidden induced subgraphs.^[5]

Relation to other graph families

Any complete graph is a comparability graph, as any acyclic orientation of a complete graph is transitive.

Any bipartite graph is also a comparability graph. Orienting the edges of a bipartite graph from one side of the bipartition to the other results in a transitive orientation.

The complement of any interval graph is a comparability graph. The comparability relation is called an interval order. Interval graphs are exactly the graphs that are chordal and that have comparability graph complements.^[6]

A permutation graph is a containment graph on a set of intervals.^[7] Therefore, permutation graphs are another subclass of comparability graphs.

The trivially perfect graphs are the comparability graphs of rooted trees.^[8] Cographs can be characterized as the comparability graphs of series-parallel partial orders; thus, cographs are also comparability graphs.^[9]

Every comparability graph is perfect. The perfection of comparability graphs can be seen as a dual form of Dilworth's theorem, and this fact together with the complementation property of perfect graphs can be used to prove Dilworth's theorem itself.^[10] More specifically, comparability graphs are perfectly orderable graphs, a subclass of perfect graphs: a greedy coloring algorithm for a topological ordering of a transitive orientation of the graph will optimally color them.^[11]

The complement graph of every comparability graph is a string graph.^[12]

Algorithms

A transitive orientation of a graph, if it exists, can be found in linear time.^[13] However, the algorithm for doing so will assign orientations to the edges of any graph, so to complete the task of testing whether a graph is a comparability graph, one must test whether the resulting orientation is transitive, a problem provably equivalent in complexity to matrix multiplication.

Because comparability graphs are perfect, many problems that are hard on more general classes of graphs, including graph coloring and the independent set problem, can be computed in polynomial time for comparability graphs.

Notes

1. ^ Golumbic (1980), p. 105; Brandstädt, Le & Spinrad (1999), p. 94.
2. ^ Ghouila-Houri (1962); see Brandstädt, Le & Spinrad (1999), theorem 1.4.1, p. 12. Although the orientations coming from partial orders are acyclic, it is not necessary to include acyclicity as a condition of this characterization.
3. ^ Urrutia (1989); Trotter (1992); Brandstädt, Le & Spinrad (1999), section 6.3, pp. 94–96.
4. ^ Ghouila-Houri (1962) and Gilmore & Hoffman (1964). See also Brandstädt, Le & Spinrad (1999), theorem 6.1.1, p. 91.
5. ^ Gallai (1967); Trotter (1992); Brandstädt, Le & Spinrad (1999), p. 91 and p. 112.
6. ^ Transitive orientability of interval graph complements was proven by Ghouila-Houri (1962); the characterization of interval graphs is due to Gilmore & Hoffman (1964). See also Golumbic (1980), prop. 1.3, pp. 15–16.
7. ^ Dushnik & Miller (1941). Brandstädt, Le & Spinrad (1999), theorem 6.3.1, p. 95.
8. ^ Brandstädt, Le & Spinrad (1999), theorem 6.6.1, p. 99.
9. ^ Brandstädt, Le & Spinrad (1999), corollary 6.4.1, p. 96; Jung (1978).
10. ^ Golumbic (1980), theorems 5.34 and 5.35, p. 133. See also the proof in the Dilworth's theorem article.
11. ^ Maffray (2003).
12. ^ Golumbic, Rotem & Urrutia (1983) and Lovász (1983). See also Fox & Pach (2009).
13. ^ McConnell & Spinrad (1997); see Brandstädt, Le & Spinrad (1999), p. 91.

References

• Brandstädt, Andreas; Le, Van Bang; Spinrad, Jeremy (1999), Graph Classes: A Survey, SIAM Monographs on Discrete Mathematics and Applications, ISBN 0-89871-432-X .
• Dushnik, Ben; Miller, E. W. (1941), "Partially ordered sets", American Journal of Mathematics (The Johns Hopkins University Press) 63 (3): 600–610, doi:10.2307/2371374, JSTOR 2371374, MR0004862 .
• Fox, J.; Pach, J. (2009), String graphs and incomparability graphs, http://www.princeton.edu/~jacobfox/papers/stringpartial071709.pdf .
• Gallai, Tibor (1967), "Transitiv orientierbare Graphen", Acta Math. Acad. Sci. Hung. 18: 25–66, doi:10.1007/BF02020961, MR0221974 .
• Gilmore, P. C.; Hoffman, A. J. (1964), "A characterization of comparability graphs and of interval graphs", Canadian Journal of Mathematics 16: 539–548, doi:10.4153/CJM-1964-055-5, MR0175811 .
• Ghouila-Houri, Alain (1962), "Caractérisation des graphes non orientés dont on peut orienter les arrêtes de manière à obtenir le graphe d'une relation d'ordre", Les Comptes rendus de l'Académie des sciences 254: 1370–1371, MR0172275 .
• Golumbic, Martin Charles (1980), Algorithmic Graph Theory and Perfect Graphs, Academic Press, ISBN 0-12-289260-7 .
• Golumbic, M.; Rotem, D.; Urrutia, J. (1983), "Comparability graphs and intersection graphs", Discrete Mathematics 43 (1): 37–46, doi:10.1016/0012-365X(83)90019-5 .
• Jung, H. A. (1978), "On a class of posets and the corresponding comparability graphs", Journal of Combinatorial Theory, Series B 24 (2): 125–133, doi:10.1016/0095-8956(78)90013-8, MR0491356 .
• Lovász, L. (1983), "Perfect graphs", Selected Topics in Graph Theory, 2, London: Academic Press, pp. 55–87 .
• Maffray, Frédéric (2003), "On the coloration of perfect graphs", in Reed, Bruce A.; Sales, Cláudia L., Recent Advances in Algorithms and Combinatorics, CMS Books in Mathematics, 11, Springer-Verlag, pp. 65–84, doi:10.1007/0-387-22444-0_3 .
• McConnell, R. M.; Spinrad, J. (1997), "Linear-time transitive orientation", 8th ACM-SIAM Symposium on Discrete Algorithms, pp. 19–25 .
• Trotter, William T. (1992), Combinatorics and Partially Ordered Sets — Dimension Theory, Johns Hopkins University Press .
• Urrutia, Jorge (1989), Rival, I., ed., Partial orders and Euclidean geometry, Kluwer Academic Publishers, pp. 327–436 .