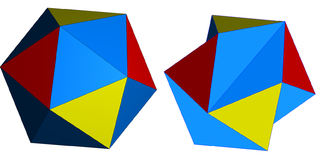
Jessen's icosahedron

The regular icosahedron and the Jessen's icosahedron.

Jessen's icosahedron, sometimes called Jessen's orthogonal icosahedron is a non-convex polyhedron with the same number of vertices, edges and faces as the regular icosahedron. It was introduced by Børge Jessen in 1967 and has several geometric properties:

• it is vertex-transitive (or isogonal),
• it has only right dihedral angles,
• it is (continuously) rigid but not infinitesimally rigid,
• as with the simpler Schönhardt polyhedron, its interior cannot be triangulated into tetrahedra without adding new vertices,
• it is scissors congruent to a cube.

Although a shape resembling Jessen's icosahedron can be formed by keeping the vertices of a regular icosahedron in their original positions and replacing some of its faces, the resulting polyhedron does not have right-angled dihedrals. The vertices of Jessen's icosahedron are perturbed from these positions in order to give all the dihedrals right angles.

See also

• The fifty nine icosahedra

References

• B. Jessen, Orthogonal Icosahedra, Nordisk Mat. Tidskr. 15 (1967), pp. 90–96.
• Peter R. Cromwell, Polyhedra, Cambridge University Press, (1997) pp. ?
• M. Goldberg, Unstable Polyhedral Structures, Math. Mag. 51 (1978), pp. 165–170
• Wells, D. The Penguin Dictionary of Curious and Interesting Geometry, London: Penguin, (1991). p. 161.

External links

• Weisstein, Eric W., "Jessen's icosahedron" from MathWorld.
• Weisstein, Eric W., "Shaky polyhedron" from MathWorld.
• Jessen's icosahedron
• Java applet