Ptolemy's table of chords

The table of chords, created by the astronomer and geometer Ptolemy in Egypt during the 2nd century AD, is a trigonometric table in Book I, chapter 11 of Ptolemy's Almagest,^[1] a treatise on mathematical astronomy. It is essentially equivalent to a table of values of the sine function. It was the earliest trigonometric table extensive enough for many practical purposes, including those of astronomy (an earlier table of chords by Hipparchus gave chords only for arcs that were multiples of 7½° = π/24 radians).^[2] Several centuries passed before more extensive trigonometric tables were created.

The chord function and the table

Example: The length of the chord subtending a 109.5° arc is approximately 98.

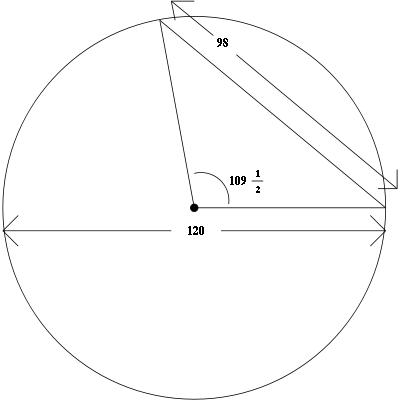
A chord of a circle is a line segment whose endpoints are on the circle. Ptolemy considered a circle of diameter 120. He tabulated the length of a chord whose endpoints are separated by an arc of n degrees, for n ranging from 1/2 to 180 by increments of 1/2. In modern notation, the length of the chord corresponding to an arc of θ degrees is

$\mathrm{chord}\ \theta = 120 \, \sin\left(\frac{\theta^\circ}{2}\right) = 60 \cdot \left( 2 \, \sin\left(\frac{\theta \cdot \pi}{360}\right) \right).$

As θ goes from 0 to 180, the chord of a θ° arc goes from 0 to 120. For tiny arcs, the chord is to the arc as π is to 3, or more precisely, the ratio can be made as close as desired to π/3 ≈ 1.04719755 by making θ small enough. Thus, for the arc of (1/2)°, the chord is slightly more than the arc. As the arc increases, the ratio of the chord to the arc decreases. When the arc reaches 60°, the chord is exactly equal to the arc, i.e. chord 60° = 60. For arcs of more than 60°, the chord is less than the arc, until an arc of 180° is reached, when the chord is only 120.

The fractional parts of chord lengths were expressed in sexagesimal, i.e. base-60, numerals. For example, where the length of a chord subtended by a 112° arc is reported to be "99  29  5", that means that its length is

$99 + \frac{29}{60} + \frac{5}{60^2},$

rounded to the nearest 1/60².^[1]

After the columns for the arc and the chord, a third column is labeled "sixtieths". For an arc of θ°, the entry in the "sixtieths" column is

$\frac{\mathrm{chord} \left(\theta + \tfrac12 \right)^\circ - \mathrm{chord} \left( \theta^\circ\right)}{1/2}.$

This is the average number of sixtieths that must be added to chord(θ°) for each 1° increase in θ°, between the entry for θ° and that for (θ + ½)°. Thus, it is used for linear interpolation. Glowatzki and Göttsche showed that Ptolemy must have calculated chords to five sexigesimal places in order to achieve the degree of accuracy found in the "sixtieths" column.^[3]

$\begin{array}{|l|rrr|rrr|} \hline \text{arc} & \text{chord} & & & \text{sixtieths} & & \\ \hline {}\,\,\,\,\,\,\,\,\,\, \tfrac12 & 0 & 31 & 25 & 1 & 2 & 50 \\ {}\,\,\,\,\,\,\, 1 & 1 & 2 & 50 & 1 & 2 & 50 \\ {}\,\,\,\,\,\,\, 1\tfrac12 & 1 & 34 & 15 & 1 & 2 & 50 \\ {}\,\,\,\,\,\,\, \vdots & \vdots & \vdots & \vdots & \vdots & \vdots & \vdots \\ 109 & 97 & 41 & 38 & 0 & 36 & 23 \\ 109\tfrac12 & 97 & 59 & 49 & 0 & 36 & 9 \\ 110 & 98 & 17 & 54 & 0 & 35 & 56 \\ 110\tfrac12 & 98 & 35 & 52 & 0 & 35 & 42\\ 111 & 98 & 53 & 43 & 0 & 35 & 29 \\ 111\tfrac12 & 99 & 11 & 27 & 0 & 35 & 15 \\ 112 & 99 & 29 & 5 & 0 & 35 & 1\\ 112\tfrac12 & 99 & 46 & 35 & 0 & 34 & 48 \\ 113 & 100 & 3 & 59 & 0 & 34 & 34 \\ {}\,\,\,\,\,\,\, \vdots & \vdots & \vdots & \vdots & \vdots & \vdots & \vdots \\ 179 & 119 & 59 & 44 & 0 & 0 & 25 \\ 179\frac12 & 119 & 59 & 56 & 0 & 0 & 9 \\ 180 & 120 & 0 & 0 & 0 & 0 & 0 \\ \hline \end{array}$

How Ptolemy computed chords

Chapter 10 of Book I of the Almagest presents geometric theorems used for computing chords. Ptolemy used geometric reasoning based on Proposition 10 of Book XIII of Euclid's Elements to find the chords of 72° and 36°. That Proposition states that if an equilateral pentagon is inscribed in a circle, then the area of the square on the side of the pentagon equals the sum of the areas of the squares on the sides of the hexagon and the decagon inscribed in the same circle.

He used Ptolemy's theorem on quadrilaterals inscribed in a circle to derive formulas for the chord of a half-arc, the chord of the sum of two arcs, and the chord of a difference of two arcs. The theorem states that for a quadrilateral inscribed in a circle, the product of the lengths of the diagonals equals the sum of the products of the two pairs of opposite sides. The derivations of trigonometric identities rely on a cyclic quadrilateral in which one side is a diameter of the circle.

To find the chords of arcs of 1°  and ½° he used approximations based on Aristarchus' inequality. The inequality states that for arcs α and β with 0 < β < α < 90°, we have

$\frac{\sin \alpha}{\sin \beta} < \frac\alpha\beta < \frac{\tan\alpha}{\tan\beta}.$

Ptolemy showed that for arcs of 1° and ½°, when rounded to two sexigesimal places after the integer part, the approximations are accurate.

The numeral system and the appearance of the untranslated table

Main article: Greek numerals

Lengths of arcs of the circle, in degrees, and the integer parts of chord lengths, were expressed in a base-10 numeral system that used 21 letters of the Greek alphabet with the meanings given in the following table, and a symbol, "∠' ", that means 1/2. Two of the letters, labeled "archaic" below, had not been in use in the Greek language for some centuries before the Almagest was written.

$\begin{array}{|rlr|rlr|rlr|} \hline \alpha & \mathrm{alpha} & 1 & \iota & \mathrm{iota} & 10 & \varrho & \mathrm{rho} & 100 \\ \beta & \mathrm{beta} & 2 & \kappa & \mathrm{kappa} & 20 & & & \\ \gamma & \mathrm{gamma} & 3 & \lambda & \mathrm{lambda} & 30 & & & \\ \delta & \mathrm{delta} & 4 & \mu & \mathrm{mu} & 40 & & & \\ \varepsilon & \mathrm{epsilon} & 5 & \nu & \mathrm{nu} & 50 & & & \\ \stigma & \mathrm{stigma\ (archaic)} & 6 & \xi & \mathrm{xi} & 60 & & & \\ \zeta & \mathrm{zeta} & 7 & \omicron & \mathrm{omicron} & 70 & & & \\ \eta & \mathrm{eta} & 8 & \pi & \mathrm{pi} & 80 & & & \\ \vartheta & \mathrm{theta} & 9 & \koppa & \mathrm{koppa\ (archaic)} & 90 & & & \\ \hline \end{array}$

Thus, for example, an arc of 143 ¹⁄₂° is expressed as $\varrho\mu\gamma\angle'$.

The fractional parts of chord lengths required great accuracy, and were given in two columns in the table: the first giving an integer multiple of 1/60, in the range 0–59, the second an integer multiple of 1/60² = 1/3600, also in the range 0–59.

Thus in Heiberg's edition of the Almagest with the table of chords on pages 48–63, the beginning of the table, corresponding to arcs from 1/2° through 7 ¹⁄₂°, looks like this:

$\begin{array}{ccc} \pi\varepsilon\varrho\iota\varphi\varepsilon\varrho\varepsilon\iota\tilde\omega\nu & \varepsilon\overset{\text{'}}\nu\vartheta\varepsilon\iota\tilde\omega\nu & \overset{\text{`}}\varepsilon\xi\eta\kappa\omicron\sigma\tau\tilde\omega\nu \\ \begin{array}{|l|} \hline \angle' \\ \alpha \\ \alpha\;\angle' \\ \hline\beta \\ \beta\;\angle' \\ \gamma \\ \hline\gamma\;\angle' \\ \delta \\ \delta\;\angle' \\ \hline\varepsilon \\ \varepsilon\;\angle' \\ \stigma \\ \hline\stigma\;\angle' \\ \zeta \\ \zeta\;\angle' \\ \hline \end{array} & \begin{array}{|r|r|r|} \hline\circ & \lambda\alpha & \kappa\varepsilon \\ \alpha & \beta & \nu \\ \alpha & \lambda\delta & \iota\varepsilon \\ \hline \beta & \varepsilon & \mu \\ \beta & \lambda\zeta & \delta \\ \gamma & \eta & \kappa\eta \\ \hline \gamma & \lambda\vartheta & \nu\beta \\ \delta & \iota\alpha & \iota\stigma \\ \delta & \mu\beta & \mu \\ \hline \varepsilon & \iota\delta & \delta \\ \varepsilon & \mu\varepsilon & \kappa\zeta \\ \stigma & \iota\stigma & \mu\vartheta \\ \hline \stigma & \mu\eta & \iota\alpha \\ \zeta & \iota\vartheta & \lambda\gamma \\ \zeta & \nu & \nu\delta \\ \hline \end{array} & \begin{array}{|r|r|r|r|} \hline \circ & \alpha & \beta & \nu \\ \circ & \alpha & \beta & \nu \\ \circ & \alpha & \beta & \nu \\ \hline \circ & \alpha & \beta & \nu \\ \circ & \alpha & \beta & \mu\eta \\ \circ & \alpha & \beta & \mu\eta \\ \hline\circ & \alpha & \beta & \mu\eta \\ \circ & \alpha & \beta & \mu\zeta \\ \circ & \alpha & \beta & \mu\zeta \\ \hline \circ & \alpha & \beta & \mu\stigma \\ \circ & \alpha & \beta & \mu\varepsilon \\ \circ & \alpha & \beta & \mu\delta \\ \hline \circ & \alpha & \beta & \mu\gamma \\ \circ & \alpha & \beta & \mu\beta \\ \circ & \alpha & \beta & \mu\alpha \\ \hline \end{array} \end{array}$

Later in the table, one can see the base-10 nature of the numerals expressing the integer parts of the arc and the chord length. Thus an arc of 85° is written as πε (π for 80 and ε for 5) and not broken down into 60 + 25, and the corresponding chord length of 81 plus a fractional part begins with πα, likewise not broken into 60 + 21. But the fractional part, 4/60 + 15/60², is written as δ, for 4, in the 1/60 column, followed by ιε, for 15, in the 1/60² column.

$\begin{array}{ccc} \pi\varepsilon\varrho\iota\varphi\varepsilon\varrho\varepsilon\iota\tilde\omega\nu & \varepsilon\overset{\text{'}}\nu\vartheta\varepsilon\iota\tilde\omega\nu & \overset{\text{`}}\varepsilon\xi\eta\kappa\omicron\sigma\tau\tilde\omega\nu \\ \begin{array}{|l|} \hline \pi\delta\angle' \\ \pi\varepsilon \\ \pi\varepsilon\angle' \\ \hline \pi\stigma \\ \pi\stigma\angle' \\ \pi\zeta \\ \hline \end{array} & \begin{array}{|r|r|r|} \hline \pi & \mu\alpha & \gamma \\ \pi\alpha & \delta & \iota\varepsilon \\ \pi\alpha & \kappa\zeta & \kappa\beta \\ \hline \pi\alpha & \nu & \kappa\delta \\ \pi\beta & \iota\gamma & \iota\vartheta \\ \pi\beta & \lambda\stigma & \vartheta \\ \hline \end{array} & \begin{array}{|r|r|r|r|} \hline \circ & \circ & \mu\stigma & \kappa\varepsilon \\ \circ & \circ & \mu\stigma & \iota\delta \\ \circ & \circ & \mu\stigma & \gamma \\ \hline \circ & \circ & \mu\varepsilon & \nu\beta \\ \circ & \circ & \mu\varepsilon & \mu \\ \circ & \circ & \mu\varepsilon & \kappa\vartheta \\ \hline \end{array} \end{array}$

The table has 45 lines on each of eight pages—thus 360 lines.

Notes and references

1. ^ ^{a b} Toomer, G. J. (1998), Ptolemy's Almagest, Princeton University Press, ISBN 0-691-00260-6 
2. ^ Thurston, pp. 235–236.
3. ^ Ernst Glowatzki and Helmut Göttsche, Die Sehnentafel des Klaudios Ptolemaios. Nach den historischen Formelplänen neuberechnet., München, 1976.

• Aaboe, Asger (1997), Episodes from the Early History of Mathematics, Mathematical Association of America, ISBN 978-0883856130 

• Clagett, Marshall (2002), Greek Science in Antiquity, Courier Dover Publications, ISBN 978-0836921502 

• Neugebauer, Otto (1975), A History of Ancient Mathematical Astronomy, Springer-Verlag, ISBN 978-0387069951 

• Thurston, Hugh (1996), Early Astronomy, Springer, ISBN 978-0387948225 

External links

• J. L. Heiberg's edition of the Almagest with the table of chords on pages 48–63
• Ptolemy's Table of Chords: Trigonometry in the Second Century, by Glenn Elert