Neighbourhood (mathematics)

For the concept in graph theory, see Neighbourhood (graph theory).

A set V in the plane is a neighbourhood of a point p if a small disk around p is contained in V.

A rectangle is not a neighbourhood of any of its corners.

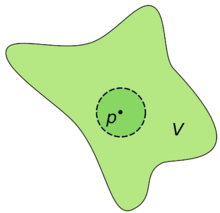
In topology and related areas of mathematics, a neighbourhood (or neighborhood) is one of the basic concepts in a topological space. Intuitively speaking, a neighbourhood of a point is a set containing the point where you can move that point some amount without leaving the set.

This concept is closely related to the concepts of open set and interior.

Definition

If X is a topological space and p is a point in X, a neighbourhood of p is a set V, which includes an open set U containing p,

$p \in U \subseteq V.$

This is also equivalent to $p\in X$ being in the interior of V.

Note that the neighbourhood V need not be an open set itself. If V is open it is called an open neighbourhood. Some authors require that neighbourhoods be open, so it is important to note conventions.

A set which is a neighbourhood of each of its points is open since it can be expressed as the union of open sets containing each of its points.

The collection of all neighbourhoods of a point is called the neighbourhood system at the point.

If S is a subset of X then a neighbourhood of S is a set V which includes an open set U containing S. It follows that a set V is a neighbourhood of S if and only if it is a neighbourhood of all the points in S. Furthermore, it follows that V is a neighbourhood of S iff S is a subset of the interior of V.

In a metric space

A set S in the plane and a uniform neighbourhood V of S.

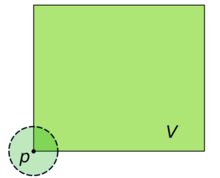
In a metric space M = (X,d), a set V is a neighbourhood of a point p if there exists an open ball with centre p and radius r, such that

$B_r(p) = B(p;r) = \{ x \in X \mid d(x,p) < r \}$

is contained in V.

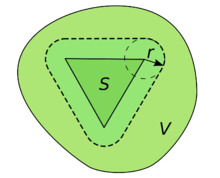
V is called uniform neighbourhood of a set S if there exists a positive number r such that for all elements p of S,

$B_r(p) = \{ x \in X \mid d(x,p) < r \}$

is contained in V.

For r > 0 the r-neighbourhood S_r of a set S is the set of all points in X which are at distance less than r from S (or equivalently, S_r is the union of all the open balls of radius r which are centred at a point in S).

It directly follows that an r-neighbourhood is a uniform neighbourhood, and that a set is a uniform neighbourhood if and only if it contains an r-neighbourhood for some value of r.

Examples

Given the set of real numbers $\mathbb{R}$ with the usual Euclidean metric and a subset V defined as

$V:=\bigcup_{n \in \mathbb{N}} B\left(n\,;\,1/n \right),$

then V is a neighbourhood for the set $\mathbb{N}$ of natural numbers, but is not a uniform neighbourhood of this set.

Topology from neighbourhoods

The above definition is useful if the notion of open set is already defined. There is an alternative way to define a topology, by first defining the neighbourhood system, and then open sets as those sets containing a neighbourhood of each of their points.

A neighbourhood system on X is the assignment of a filter N(x) (on the set X) to each x in X, such that

1. the point x is an element of each U in N(x)
2. each U in N(x) contains some V in N(x) such that for each y in V, U is in N(y).

One can show that both definitions are compatible, i.e. the topology obtained from the neighbourhood system defined using open sets is the original one, and vice versa when starting out from a neighbourhood system.

Uniform neighbourhoods

In a uniform space S = (X,δ), V is called a uniform neighbourhood of P if P is not close to $X \setminus V$, that is there exists no entourage containing P and $X \setminus V$.

Punctured neighbourhood

A punctured neighbourhood of a point p (sometimes called a deleted neighbourhood) is a neighbourhood of p, without {p}. For instance, the interval ( − 1,1) = {y: − 1 < y < 1} is a neighbourhood of p = 0 in the real line, so the set $(-1, 0) \cup (0, 1) = (-1, 1) \setminus \{0\}$ is a punctured neighbourhood of 0. Note that a punctured neighbourhood of a given point is not in fact a neighbourhood of the point. The concept of punctured neighbourhood occurs in the definition of the limit of a function.

See also

• Tubular neighborhood

References

• Kelley, John L. (1975). General topology. New York: Springer-Verlag. ISBN 0387901256. 
• Bredon, Glen E. (1993). Topology and geometry. New York: Springer-Verlag. ISBN 0387979263. 
• Kaplansky, Irving (2001). Set Theory and Metric Spaces. American Mathematical Society. ISBN 0821826948.