Mason's rule

Mason's gain formula (MGF) is a method for finding the transfer function of a linear signal-flow graph (SFG). The formula was derived by Samuel Jefferson Mason^[1] and is named after its discoverer. MGF is an alternate method to finding the transfer function algebraically by labeling each signal, writing down the equation for how that signal depends on other signals, and then solving the multiple equations for the output signal in terms of the input signal. MGF provides a step by step method to obtain the transfer function from a SFG. Often, MGF can be determined by inspection of the SFG. The method can easily handle SFGs with many variables and loops including loops with inner loops. The method, being simple and mechanical and can often require less effort and is less error prone than the direct algebraic method. MGF comes up often in the context of control systems and digital filters because control systems and digital filters are often represented by SFGs.

Formula

The gain formula is as follows:

$G = \frac{y_\text{out}}{y_\text{in}} = \frac{ \sum_{k=1}^N {G_k \Delta _k} }{ \Delta\ }$

$\Delta = 1 - \sum L_i + \sum L_iL_j- \sum L_iL_jL_k + \cdots + (-1)^m \sum \cdots +\cdots$

where:

• Δ = the determinant of the graph.
• y_in = input-node variable
• y_out = output-node variable
• G = complete gain between y_in and y_out
• N = total number of forward paths between y_in and y_out
• G_k = gain of the kth forward path between y_in and y_out
• L_i = loop gain of each closed loop in the system
• L_iL_j = product of the loop gains of any two non-touching loops (no common nodes)
• L_iL_jL_k = product of the loop gains of any three pairwise nontouching loops
• Δ_k = the cofactor value of Δ for the k^th forward path, with the loops touching the k^th forward path removed. I.e. Remove those parts of the graph which form the loop, while retaining the parts needed for the forward path.

Procedure

To use this technique,

1. Make a list of all forward paths, and their gains, and label these G_k.
2. Make a list of all the loops and their gains, and label these L_i (for i loops). Make a list of all pairs of non-touching loops, and the products of their gains (L_iL_j). Make a list of all pairwise non-touching loops taken three at a time (L_iL_jL_k), then four at a time, and so forth, until there are no more.
3. Compute the determinant Δ and cofactors Δ_k.
4. Apply the formula.

Examples

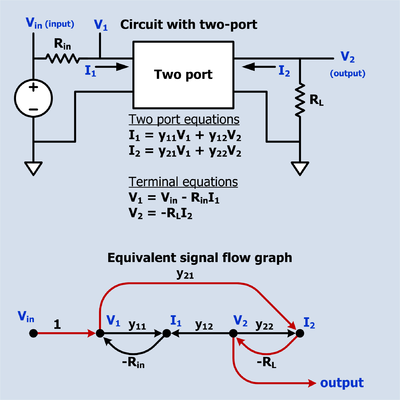
Circuit containing two-port

Signal flow graph of a circuit containing a two port. The forward path from input to output is shown in a different color.

The transfer function from V_in to V₂ is desired.

There is only one forward path:

• V_in to V₁ to I₂ to V₂ with gain $G_1 = -y_{21} R_L \,$

There are three loops:

• V₁ to I₁ to V₁ with gain $L_1 = -R_{in} y_{11} \,$
• V₂ to I₂ to V₂ with gain $L_2 = -R_L y_{22} \,$
• V₁ to I₂ to V₂ to I₁ to V₁ with gain $L_3 = y_{21} R_L y_{12} R_{in} \,$

$\Delta = 1 - ( L_1 + L_2 + L_3 ) + ( L_1 L_2 ) \,$ note: L₁ and L₂ do not touch each other whereas L₃ touches both of the other loops.

$\Delta_1 = 1 \,$ note: the forward path touches all the loops so all that is left is 1.

$G = \frac { G_1 \Delta_1 } { \Delta } = \frac { -y_{21} R_L } {1 + R_{in}y_{11} + R_L y_{22} - y_{21} R_L y_{12} R_{in} + R_{in}y_{11} R_L y_{22} } \,$

Digital IIR biquad filter

The signal flow graph (SFG) for a digital infinite impulse response bi-quad filter. This SFG has three forward paths and two loops.

Digital filters are often diagramed as signal flow graphs.

There are two loops

• $L_1 = -a_1 Z^{-1} \,$
• $L_2 = -a_2 Z^{-2} \,$

$\Delta = 1 - ( L_1 + L_2 ) \,$ Note, the two loops touch so there is no term for their product.

There are three forward paths

• $G_0 = b_0 \,$
• $G_1 = b_1 Z^{-1} \,$
• $G_2 = b_2 Z^{-2} \,$

All the forward paths touch all the loops so $\Delta_0 = \Delta_1 = \Delta_2 = 1 \,$

$G = \frac { G_0 \Delta_0 +G_1 \Delta_1 + G_2 \Delta_2 } {\Delta} \,$

$G = \frac { b_0 + b_1 Z^{-1} + b_2 Z^{-2} } {1 +a_1 Z^{-1} + a_2 Z^{-2} } \,$

Servo

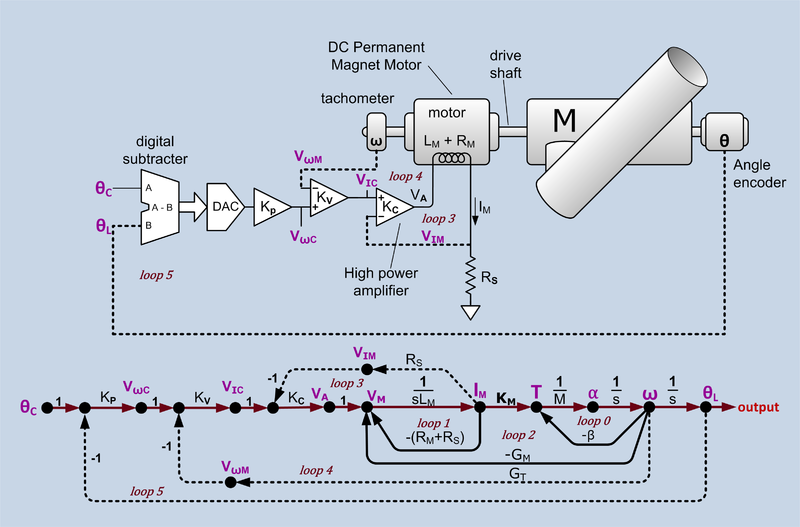Angular position servo and signal flow graph. θ_C = desired angle command, θ_L = actual load angle, K_P = position loop gain, V_ωC = velocity command, V_ωM = motor velocity sense voltage, K_V = velocity loop gain, V_IC = current command, V_IM = current sense voltage, K_C = current loop gain, V_A = power amplifier output voltage, V_M = effective voltage across the inductance, L_M = motor inductance, I_M = motor current, R_M = motor resistance, R_S = current sense resistance, K_M = motor torque constant (Nm/amp) , T = torque, M = momment of inertia of all rotating components α = angular acceleration, ω = angular velocity, β = mechanical damping, G_M = motor back EMF constant, G_T = tachometer conversion gain constant,. There is one forward path (shown in a different color) and six feedback loops. The drive shaft assumed to be stiff enough to not treat as a spring. Constants are shown in black and variables in purple.

The signal flow graph has six loops. They are:

• $L_0 = - \frac {\beta} {sM} \,$

• $L_1 = \frac{-1} {sL_M( R_M + R_S)} \,$

• $L_2 = \, \frac {-G_M K_M} {s^2 L_M M}$

• $L_3 = \frac {-K_C R_S} {sL_M} \,$

• $L_4 = \frac {-K_V K_C K_M G_T} {s^2 L_M M} \,$

• $L_5 = \frac {-K_P K_V K_C K_M } {s^3 L_M M} \,$

$\Delta = 1 - (L_0+L_1+L_2+L_3+L_4+L_5) + (L_0 L_1 + L_0 L_3)\,$

There is one forward path:

• $g_0 = \frac {-K_P K_V K_C K_M } {s^3 L_M M} \,$

The forward path touches all the loops therefore the co-factor Δ₀ = 1

And the gain from input to output is $\frac {\theta_L} {\theta_C} = \frac {g_0 \Delta_0} {\Delta} \,$

Equivalent matrix form

Mason's rule can be stated in a simple matrix form. Assume $\mathbf{T}$ is the transient matrix of the graph where $t_{nm} = \left[\mathbf{T}\right]_{nm}$ is the sum transmittance of branches from node m toward node n. Then, the gain from node m to node n of the graph is equal to $u_{nm} = \left[\mathbf{U}\right]_{nm}$, where

$\mathbf{U} = \left ( \mathbf{I} - \mathbf{T} \right ) ^ {-1}$,

and $\mathbf{I}$ is the identity matrix.

Mason's Rule is also particularly useful for deriving the z-domain transfer function of discrete networks that have inner feedback loops embedded within outer feedback loops (nested loops). If the discrete network can be drawn as a signal flow graph, then the application of Mason's Rule will give that network's z-domain H(z) transfer function.

Notes

1. ^ Mason, Samuel J. (July 1956). "Feedback Theory - Further Properties of Signal Flow Graphs". Proceedings of the IRE: 920–926. 

References

• Bolton, W. Newnes (1998). Control Engineering Pocketbook. Oxford: Newnes. 
• Van Valkenburg, M. E. (1974). Network Analysis (3rd ed.). Englewood Cliffs, NJ: Prentice-Hall. 

A mason rule example