Orbifold notation

In geometry, orbifold notation (or orbifold signature) is a system, invented by William Thurston and popularized by the mathematician John Conway, for representing types of symmetry groups in two-dimensional spaces of constant curvature. The advantage of the notation is that it describes these groups in a way which indicates many of the groups' properties: in particular, it describes the orbifold obtained by taking the quotient of Euclidean space by the group under consideration.

Groups representable in this notation include the point groups on the sphere (S²), the frieze groups and wallpaper groups of the Euclidean plane (E²), and their analogues on the hyperbolic plane (H²).

Definition of the notation

The following types of Euclidean transformation can occur in a group described by orbifold notation:

• reflection through a line (or plane)
• translation by a vector
• rotation of finite order around a point
• infinite rotation around a line in 3-space
• glide-reflection, i.e. reflection followed by translation

All translations which occur are assumed to form a discrete subgroup of the group symmetries being described.

Each group is denoted in orbifold notation by a finite string made up from the following symbols:

• positive integers $1,2,3,\dots$
• the infinity symbol, $\infty$
• the asterisk, *
• the symbol o, which is called a wonder (Older documents show this as a solid circle)
• the symbol x, which is called a miracle (Older documents show this as an open circle)

A string written in boldface represents a group of symmetries of Euclidean 3-space. A string not written in boldface represents a group of symmetries of the Euclidean plane, which is assumed to contain two independent translations.

Each symbol corresponds to a distinct transformation:

• an integer n to the left of an asterisk indicates a rotation of order n around a point
• an integer n to the right of an asterisk indicates a transformation of order 2n which rotates around a point and reflects through a line (or plane)
• an x indicates a glide reflection
• the symbol $\infty$ indicates infinite rotational symmetry around a line; it can only occur for bold face groups. By abuse of language, we might say that such a group is a subgroup of symmetries of the Euclidean plane with only one independent translation. The frieze groups occur in this way.
• the exceptional symbol o indicates that there are precisely two linearly independent translations.

Chirality and achirality

An object is chiral if its symmetry group contains no reflections; otherwise it is called achiral. The corresponding orbifold is orientable in the chiral case and non-orientable otherwise.

The Euler characteristic and the order

The Euler characteristic of an orbifold can be read from its Conway symbol, as follows. Each feature has a value:

• n without or before an asterisk counts as $\frac{n-1}{n}$

• n after an asterisk counts as $\frac{n-1}{2 n}$

• asterisk and x count as 1

• o counts as 2

Subtracting the sum of these values from 2 gives the Euler characteristic.

If the sum of the feature values is 2, the order is infinite, i.e., the notation represents a wallpaper group or a frieze group. Indeed, Conway's "Magic Theorem" indicates that the 17 wallpaper groups are exactly those with the sum of the feature values equal to 2. Otherwise, the order is 2 divided by the Euler characteristic.

Equal groups

The following groups are isomorphic:

• 1* and *11
• 22 and 221
• *22 and *221
• 2* and 2*1

This is because 1-fold rotation is the "empty" rotation.

Other objects

A perfect snowflake would have *66 symmetry,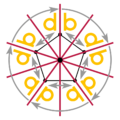

The pentagon has symmetry *55, the whole image with arrows 55.

The Flag of Hong Kong has 5 fold rotation symmetry, 55.

The symmetry of a 2D object without translational symmetry can be described by the 3D symmetry type by adding a third dimension to the object which does not add or spoil symmetry. For example, for a 2D image we can consider a piece of carton with that image displayed on one side; the shape of the carton should be such that it does not spoil the symmetry, or it can be imagined to be infinite. Thus we have nn and *nn.

Similarly, a 1D image can be drawn horizontally on a piece of carton, with a provision to avoid additional symmetry with respect to the line of the image, e.g. by drawing a horizontal bar under the image. Thus the discrete symmetry groups in one dimension are 11, *11, $\infty\infty$ and *$\infty\infty$.

Another way of constructing a 3D object from a 1D or 2D object for describing the symmetry is taking the Cartesian product of the object and an asymmetric 2D or 1D object, respectively.

Correspondence tables

Spherical

532 symmetry

See also: List of spherical symmetry groups

Spherical Symmetry Groups: (n=3,4,..)^[1]

Orbifold Signature	Coxeter	Schönflies	Hermann–Mauguin	Order
Polyhedral groups
*532	[3,5]	Ih	53m	120
532	[3,5]+	I	532	60
*432	[3,4]	Oh	m3m	48
432	[3,4]+	O	432	24
*332	[3,3]	Td	43m	24
3*2	[3+,4]	Th	m3	24
332	[3,3]+	T	23	12
Dihedral and cyclic groups: n=3,4,5...
*22n	[2,n]	Dnh	n/mmm or 2nm2	4n
2*n	[2+,2n]	Dnd	2n2m or nm	4n
22n	[2,n]+	Dn	n2	2n
*nn	[n]	Cnv	nm	2n
n*	[2,n+]	Cnh	n/m or 2n	2n
nx	[2+,2n+]	S2n	2n or n	2n
nn	[n]+	Cn	n	n
Special cases
*222	[2,2]	D2h	2/mmm or 22m2	8
2*2	[2+,4]	D2d	222m or 2m	8
222	[2,2]+	D2	22	4
*22	[2]	C2v	2m	4
2*	[2,2+]	C2h	2/m or 22	4
2x	[2+,4+]	S4	22 or 2	4
22	[2]+	C2	2	2
*221	[1,2]	D1h	1/mmm or 21m2	4
2*1	[2+,2]	D1d	212m or 1m	4
221	[1,2]+	D1	12	2
*11	[ ]	C1v	1m	2
1*	[2,1+]	C1h	1/m or 21	2
1x	[2+,2+]	S2	21 or 1	2
11	[ ]+	C1	1	1

Euclidean plane

See also: List of planar symmetry groups

Frieze groups

Frieze groups

Notations				Description	Examples
IUC	Orbifold	Coxeter	Schönflies*
p1	∞∞	[∞,1]+	C∞	(hop): Translations only. This group is singly generated, with a generator being a translation by the smallest distance over which the pattern is periodic. Abstract group: Z, the group of integers under addition.
p11g	∞x	[∞+,2+]	S∞	(step): Glide-reflections and translations. This group is generated by a glide reflection, with translations being obtained by combining two glide reflections. Abstract group: Z
p11m	∞*	[∞+,2]	C∞h	(jump): Translations, the reflection in the horizontal axis and glide reflections. This group is generated by a translation and the reflection in the horizontal axis. Abstract group: Z × Z2
p1m1	*∞∞	[∞,1]	C∞v	(sidle): Translations and reflections across certain vertical lines. The group is the same as the non-trivial group in the one-dimensional case; it is generated by a translation and a reflection in the vertical axis. The elements in this group correspond to isometries (or equivalently, bijective affine transformations) of the set of integers, and so it is isomorphic to a semidirect product of the integers with Z2. Abstract group: Dih∞, the infinite dihedral group.
p2	22∞	[∞,2]+	D∞	(spinning hop): Translations and 180° rotations. The group is generated by a translation and a 180° rotation. Abstract group: Dih∞
p2mg	2*∞	[∞,2+]	D∞d	(spinning sidle): Reflections across certain vertical lines, glide reflections, translations and rotations. The translations here arise from the glide reflections, so this group is generated by a glide reflection and either a rotation or a vertical reflection. Abstract group: Abstract group: Dih∞
p2mm	*22∞	[∞,2]	D∞h	(spinning jump): Translations, glide reflections, reflections in both axes and 180° rotations. This group is the "largest" frieze group and requires three generators, with one generating set consisting of a translation, the reflection in the horizontal axis and a reflection across a vertical axis. Abstract group: Dih∞ × Z2
*Schönflies's point group notation is extended here as infinite cases of the equivalent dihedral points symmetries

Wallpaper groups

632 symmetry

17 wallpaper groups^[2]

Orbifold Signature	Coxeter	Hermann–Mauguin	Speiser Niggli	Polya Guggenhein	Fejes Toth Cadwell
*632	[6,3]	p6m	C(I)6v	D6	W16
632	[6,3]+	p6	C(I)6	C6	W6
*442	[4,4]	p4m	C(I)4	D*4	W14
4*2	[4+,4]	p4g	CII4v	Do4	W24
442	[4,4]+	p4	C(I)4	C4	W4
*333	[3[3]]	p3m1	CII3v	D*3	W13
3*3	[6,3+]	p31m	CI3v	Do4	W23
333	[3[3]]+	p3	CI3	C3	W3
*2222	[∞,2,∞]	pmm	CI2v	D2kkkk	W22
2*22	[∞,2+,∞]	cmm	CIV2v	D2kgkg	W12
22*	[(∞,2)+,∞]	pmg	CIII2v	D2kkgg	W32
22x	[∞+,2+,∞+]	pgg	CII2v	D2gggg	W42
2222	[∞,2,∞]+	p2	C(I)2	C2	W2
**	[∞,2,∞+]	pm	CIs	D1kk	W21
*x	[∞,2+,∞+]	cm	CIIIs	D1kg	W11
xx	[(∞,2)+,∞+]	pg	CII2	D1gg	W31
o	[∞+,2,∞+]	p1	C(I)1	C1	W1

Hyperbolic plane

732 symmetry

A first few hyperbolic groups, ordered by their orbifold characteristic are:

Hyperbolic Symmetry Groups^[3]

(-1/char)	Orbifolds	Coxeter
(84)	*237	[7,3]
(48)	*238	[8,3]
(42)	237	[7,3]+
(40)	*245	[5,4]
(24)	*2.3.12, *246, *334, 3*4, 238	[12,3], [6,4], [(4,3,3)], [8,3]+
(20)	*2.3.15, *255, 5*2, 245	[15,3], [5,5], [5+,4], [5,4]+
(18+2/3)	*247	[7,4]
(18)	*2.3.18, 239	[18,3], [9,3]+
(16)	*2.3.24, *248	[24,3], [8,4]
(15)	*2.3.30, *256, *335, 3*5, 2.3.10	[30,3], [6,5], [(5,3,3)], [3+,10], [10,3]+
(14+2/5)	*2.3.36, *249	[36,3], [9,4]
(13+1/3)	*2.3.60, *2.4.10	[60,3], [10,4]
(13+1/5)	*2.3.66, 2.3.11	[66,3], [11,3]+
(12+8/11)	*2.3.105, *257	[105,3], [7,5]
(12+4/7)	*2.3.132, *2.4.11 ... *23∞, *2.4.12, *266, 6*2	[132,3], [11,4], ..., [∞,3], [12,4], [6,6], [6+,4]
(12)	*336, 3*6, *344, 4*3, *2223, 2*23, 2.3.12, 246, 334	[(6,3,3)], [3+,12], [(4,4,3)], [4+,6], ... [12,3]+, [6,4]+ [(4,3,3)]+
...

See also

• Fibrifold notation - an extension of orbifold notation for 3d space groups

References

1. ^ Symmetries of Things, Appendix A, page 416
2. ^ Symmetries of Things, Appendix A, page 416
3. ^ Symmetries of Things, Chapter 18, More on Hyperbolic groups, Enumerating hyperbolic groups, p239

• John H. Conway, Olaf Delgado Friedrichs, Daniel H. Huson, and William P. Thurston. On Three-dimensional Orbifolds and Space Groups. Contributions to Algebra and Geometry, 42(2):475-507, 2001.
• J. H. Conway, D. H. Huson. The Orbifold Notation for Two-Dimensional Groups. Structural Chemistry, 13 (3-4): 247-257, August 2002.
• J. H. Conway (1992). "The Orbifold Notation for Surface Groups". In: M. W. Liebeck and J. Saxl (eds.), Groups, Combinatorics and Geometry, Proceedings of the L.M.S. Durham Symposium, July 5–15, Durham, UK, 1990; London Math. Soc. Lecture Notes Series 165. Cambridge University Press, Cambridge. pp. 438–447
• John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, The Symmetries of Things 2008, ISBN 978-1-56881-220-5

External links

• A field guide to the orbifolds (Notes from class on "Geometry and the Imagination" in Minneapolis, with John Conway, Peter Doyle, Jane Gilman and Bill Thurston, on June 17-28, 1991. See also PDF, 2006)
• 2DTiler Software for visualizing two-dimensional tilings of the plane and editing their symmetry groups in orbifold notation