Continuous Progress Mathematics

Continuous Progress Mathematics

The Continuous Progress Mathematics (CPM) system of multi-age classrooms organizes students into classes based on their immediate instructional level. Implemented at Peach Plains Elementary School in Grand Haven, Michigan, in the fall of 1992, instructional groups called terminals are composed of students who have mastered the critical prerequisites of some 250 objectives within 28 areas of mathematical knowledge. Individual learner mastery of the non-linear hierarchy of mathematical objectives is carefully monitored by teachers and curriculum management software allowing all students to advance through the same curriculum, albeit at different times[1].

Contents

History

Stephen E. Rubin, Ph.D, of Westport, Connecticut, originally designed the hierarchy of mathematical objectives for Center School in New Canaan, Connecticut. Grand Haven Area Public Schools adapted the hierarchy and contracted Tom Orzechowski, systems engineer, to develop a networked DOS version of the management software in the summer of 1992. Kirby Chittenden provided design consultation for the graphical user interface and the software functionality. Additional design support was provided by Melinda Brink, principal of Peach Plains Elementary School, and the school’s math coordinator, Jean Sharp.

Design

A Section of the Mathematics Hierarchy

CPM was designed around the developmental philosophy that learning is more a function of time rather than ability[2]. Within this design, elementary students leave their homerooms each afternoon to participate in a multi-age instructional group called a terminal. Students flow in and out of these terminals as they are able to demonstrate mastery.

These instructional groups are organized according to two criteria:

  1. Everyone in a terminal shares a common need to learn the same thing, with the group formed around a specific skill, objective, or outcome; and
  2. Everyone in a terminal has already mastered the objectives that are prerequisite to learning this new objective.

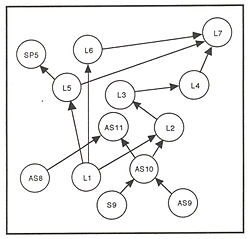
Classes in various mathematical strands such subtraction (S), decimals (L), statistic and probability (SP) are open and closed as student eligibility increases or decreases respectively. This fluency allows for all students to move through the hierarchy at developmentally appropriate times[3].

Delivery

Teachers often provide a focused whole group instruction based upon one of some 250 objectives available to students. In addition, students may participate in independent or small group activities using classroom manipulatives, learning center activities, cooperative learning groups, peer tutoring, and computer courseware.

Instructors have full latitude to decide what will be used in the way of resources to bring each student to the point of mastery. Not all students achieve success on the same day and in the same way. Level of mastery, rather than rate, is emphasized.

A criterion referenced test allows the student to demonstrate mastery of the terminal objective. The management software then assigns the student to an open eligible class the next day. The software also determines which classes need to open and close based upon student eligibility.

Technology

CPM Management Software Locale Feature

Early management software, designed to operate on the Apple II platform, did not take full advantage of developments in relational database management. In addition, advancements in object-oriented program language allowed access to information from various sources in a substantially more graphic form. The new management software developed by Tom Orzechowski integrated eight independent databases with the object-oriented characteristics of Borland’s Paradox (database) software development engine[4].

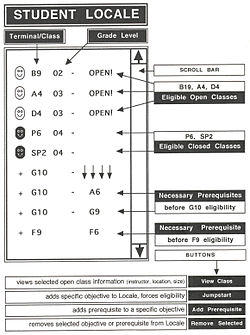
An integral part of Orzechowski’s development process is that of a student’s Locale, or their whereabouts in the hierarchy of mathematical objectives. The Locale (see diagram) provided data about each child’s skill set and functioned as a decision making tool and gateway for enrolling students in appropriate classes each day.

References

  1. ^ Chittenden, K.L. (1993) Outome based mathematics: A case study in change. Education 695 final project. Grand Valley State University.
  2. ^ Rubin, S.E. (1990) Public schools should learn to ski: A design for educating in the 21st century. International Journal of Educational Management, 4 (4), 5-17.
  3. ^ Rubin, S.E. (1993). Resource-based education: It’s like inviting kids to a buffet instead of a sit-down dinner. The New England League of Middle Schools Journal, 6 (1), 8-13.
  4. ^ Orzechowski, T. (1993) Outcome based curriculum management system. [Computer program]. Grand Rapids, MI: Author.

Wikimedia Foundation. 2010.

Игры ⚽ Поможем написать курсовую

Look at other dictionaries:

  • Continuous modelling — is the mathematical practice of applying a model to continuous data (data which has a potentially infinite number, and divisibility, of attributes). They often use differential equations and are converse to discrete modelling. Modelling is… …   Wikipedia

  • Mathematics and art — have a long historical relationship. The ancient Egyptians and ancient Greeks knew about the golden ratio, regarded as an aesthetically pleasing ratio, and incorporated it into the design of monuments including the Great Pyramid,[1] the Parthenon …   Wikipedia

  • mathematics — /math euh mat iks/, n. 1. (used with a sing. v.) the systematic treatment of magnitude, relationships between figures and forms, and relations between quantities expressed symbolically. 2. (used with a sing. or pl. v.) mathematical procedures,… …   Universalium

  • Mathematics and Physical Sciences — ▪ 2003 Introduction Mathematics       Mathematics in 2002 was marked by two discoveries in number theory. The first may have practical implications; the second satisfied a 150 year old curiosity.       Computer scientist Manindra Agrawal of the… …   Universalium

  • Progress (philosophy) — Historical progress has been a main object of philosophy of history. However, in particular following the Holocaust, the idea and possibility of social progress, which was a main idea of the Enlightenment philosophy, has been more and more put to …   Wikipedia

  • Computer Based Mathematics Education — (CBME) refers to a mathematics education method that is enriched by using computers. In order to analyze CBME in detail, the disciplines of mathematics, education, and computers including their features should be explored more and understood… …   Wikipedia

  • Science and mathematics from the Renaissance to Descartes — George Molland Early in the nineteenth century John Playfair wrote for the Encyclopaedia Britannica a long article entitled ‘Dissertation; exhibiting a General View of the Progress of Mathematics and Physical Science, since the Revival of Letters …   History of philosophy

  • Logic and the philosophy of mathematics in the nineteenth century — John Stillwell INTRODUCTION In its history of over two thousand years, mathematics has seldom been disturbed by philosophical disputes. Ever since Plato, who is said to have put the slogan ‘Let no one who is not a geometer enter here’ over the… …   History of philosophy

  • Space (mathematics) — This article is about mathematical structures called spaces. For space as a geometric concept, see Euclidean space. For all other uses, see space (disambiguation). A hierarchy of mathematical spaces: The inner product induces a norm. The norm… …   Wikipedia

  • History of mathematics — A proof from Euclid s Elements, widely considered the most influential textbook of all time.[1] …   Wikipedia

Share the article and excerpts

Direct link
… Do a right-click on the link above
and select “Copy Link”