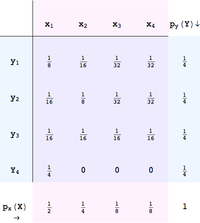
Marginal distribution

In probability theory and statistics, the marginal distribution of a subset of a collection of random variables is the probability distribution of the variables contained in the subset. The term marginal variable is used to refer to those variables in the subset of variables being retained. These terms are dubbed "marginal" because they used to be found by summing values in a table along rows or columns, and writing the sum in the margins of the table.^[1] The distribution of the marginal variables (the marginal distribution) is obtained by marginalizing over the distribution of the variables being discarded, and the discarded variables are said to have been marginalized out.

The context here is that the theoretical studies being undertaken, or the data analysis being done, involves a wider set of random variables but that attention is being limited to a reduced number of those variables. In many applications an analysis may start with a given collection of random variables, then first extend the set by defining new ones (such as the sum of the original random variables) and finally reduce the number by placing interest in the marginal distribution of a subset (such as the sum). Several different analyses may be done, each treating a different subset of variables as the marginal variables.

Two-variable case

Joint and marginal distributions of a pair of discrete, random variables X,Y having nonzero mutual information I(X; Y).

Given two random variables X and Y whose joint distribution is known, the marginal distribution of X is simply the probability distribution of X averaging over information about Y. This is typically calculated by summing or integrating the joint probability distribution over Y.

For discrete random variables, the marginal probability mass function can be written as Pr(X = x). This is

Pr(X = x) =	∑	Pr(X = x,Y = y) =	∑	Pr(X = x | Y = y)Pr(Y = y),
	y		y

where Pr(X = x,Y = y) is the joint distribution of X and Y, while Pr(X = x|Y = y) is the conditional distribution of X given Y. In this case, the variable Y has been marginalized out.

Bivariate marginal and joint probabilities for discrete random variables are often displayed as two-way tables.

Similarly for continuous random variables, the marginal probability density function can be written as p_X(x). This is

$p_{X}(x) = \int_y p_{X,Y}(x,y) \, dy = \int_y p_{X|Y}(x|y) \, p_Y(y) \, dy ,$

where p_X,Y(x,y) gives the joint distribution of X and Y, while p_X|Y(x|y) gives the conditional distribution for X given Y. Again, the variable Y has been marginalized out.

Real-world example

Imagine for example you want to compute the probability that a pedestrian will be hit by a car while crossing the road at a pedestrian crossing. Let H be a discrete random variable describing the probability of being hit by a car while walking over the crossing, taking one value from { hit, not hit }. Let L be a discrete random variable describing the probability of the cross traffic's traffic light state at a given moment, taking one from { red, yellow, green }.

Realistically, H will be dependent on L. That is, P(H = hit) and P(H = not hit) will take different values depending on whether L is red, yellow or green. You are, for example, far more likely to be hit by a car if you try to cross while the lights for cross traffic are green than if they are red. In other words, for any given possible pair of values for H and L, you must feed them into the joint probability distribution of H and L to find the probability of that pair of events occurring together.

However, in trying to calculate the marginal probability P(H=hit), what we are asking for is the probability that H=hit, where we don't actually know the particular value of L. In general you can be hit if the lights are red OR if the lights are yellow OR if the lights are green. So in this case the answer for the marginal probability can be found by summing P(H,L) = P(hit,L) for all possible values of L.

Here is a table showing the conditional probabilities of being hit, depending on the state of the lights. (Note that due to the dependence, only the columns in this table must add up to 1).

Conditional distribution: P(H|L)
	L=Red	L=Yellow	L=Green
H=Not Hit	0.99	0.9	0.2
H=Hit	0.01	0.1	0.8

To find the joint probability distribution, we need more data. Let's say that P(L=red) = 0.7, P(L=yellow) = 0.1, P(L=green) = 0.2. Multiplying the columns in the conditional distribution by the appropriate values, we find the joint probability distribution of H and L. (Note that the cells in this table, excluding the marginal probabilities, now add up to 1).

Joint distribution: P(H,L)
	L=Red	L=Yellow	L=Green	Marginal probability
H=Not Hit	0.693	0.09	0.04	0.823
H=Hit	0.007	0.01	0.16	0.177
Total	0.7	0.1	0.2	1

The marginal probability P(H=Hit) is the sum of the bottom row (above the totals row), as this is the probability of being hit when the lights are red OR yellow OR green. Similarly, the marginal probability that P(H=Not Hit) is the sum of the top row. It is important to interpret this result correctly. The chance of being hit by a car when you cross the road is obviously a lot less than 17.7%. However, what this figure is actually saying is that if you were to ignore the state of the traffic lights and cross the road no matter their color, you would have a 17.7% risk of being hit by a car. This seems more reasonable.

General cases

For multivariate distributions, formulae similar to those above apply with the symbols X and/or Y being interpreted as vectors. In particular, each summation or integration would be over all variables except those contained in X.

See also

• Joint probability distribution
• Wasserstein metric

References

1. ^ Trumpler and Weaver (1962), pp. 32–33.

Bibliography

• Everitt, B. S. (2002). The Cambridge Dictionary of Statistics. Cambridge University Press. ISBN 0-521-81099-x. 
• Trumpler, Robert J. and Harold F. Weaver (1962). Statistical Astronomy. Dover Publications.