Contact process (mathematics)

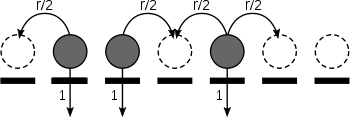
The Contact Process (on a 1-D lattice): Active sites are indicated by grey circles and inactive sites by dotted circles. Active sites can activate inactive sites to either side of them at a rate r/2 or become inactive at rate 1.

The contact process is a model of an interacting particle system. It is a continuous time Markov process with state space {0,1}^S, where S is a finite or countable graph, usually Z^d. The process is usually interpreted as a model for the spread of an infection: if the state of the process at a given time is η, then a site x in S is "infected" if η(x) = 1 and healthy if η(x) = 0. Infected sites become healthy at a constant rate, while healthy sites become infected at a rate proportional to the number infected neighbors. One can generalize the state space to $\{0,\ldots, \kappa\}^S$, such is called the multitype contact process. It represents a model when more than one type of infection is competing for space.

More specifically, the dynamics of the basic contact process is defined by the following transition rates: at site x,

$1\rightarrow0\quad\mbox{at rate }1,$

$0\rightarrow1\quad\mbox{at rate }\lambda\sum_{y:y\sim x}\eta(y),$

where the sum is over all the neighbors in S of x. This means that each site waits an exponential time with the corresponding rate, and then flips (so 0 becomes 1 and viceversa).

For each graph S there exists a critical value λ_c for the parameter λ so that if λ > λ_c then the 1's survive (that is, if there is at least one 1 at time zero, then at any time there are ones) with positive probability, while if λ < λ_c then the process dies out. For contact process on the integer lattice, a major breakthrough came in 1990 when Bezuidenhout and Grimmett showed that the contact process also dies out at the critical value. Their proof makes use of percolation theory.

Voter model

The voter model (usually in continuous time, but there are discrete versions as well) is a process similar to the contact process. In this process η(x) is taken to represent a voter's attitude on a particular topic. Voters reconsider their opinions at times distributed according to independent exponential random variables (this gives a Poisson process locally-- note that there are in general infinitely many voters so no global Poisson process can be used). At times of reconsideration, a voter chooses one neighbor uniformly from amongst all neighbors and takes that neighbor's opinion. One can generalize the process by allowing the picking of neighbors to be something other than uniform.

Discrete time process

In the discrete time voter model in one dimension, $\xi_t(x): \mathbb{Z} \to \{0,1\}$ represents the state of particle x at time t. Informally each individual is arranged on a line and can "see" other individuals that are within a radius, r. If more than a certain proportion, θ of these people disagree then the individual changes her attitude, otherwise she keeps it the same. Durrett and Steif (1993) and Steif (1994) show that for large radii there is a critical value θ_c such that if θ > θ_c most individuals never change, and for $\theta \in (1/2, \theta_c)$ in the limit most sites agree. (Both of these results assume the probability of ξ₀(x) = 1 is one half.)

This process has a natural generalization to more dimensions, some results for this are discussed in Durrett and Steif (1993).

Continuous time process

The continuous time process is similar in that it imagines each individual has a belief at a time and changes it based on the attitudes of its neighbors. The process is described informally by Liggett (1985, 226), "Periodically (i.e., at independent exponential times), an individual reassesses his view in a rather simple way: he chooses a 'friend' at random with certain probabilities and adopts his position." A model was constructed with this interpretation first by Holley and Liggett (1975).

This process is analogous to a process first suggested by Clifford and Sudbury (1973) where animals are conflicting over territory and the animals are equally matched. A site is selected to be invaded by a neighbor at a given time.

References

• Clifford, Peter; Aidan Sudbury (1973). "A Model for Spatial Conflict". Biometrika 60 (3): 581–588. doi:10.1093/biomet/60.3.581. 
• Durrett, Richard; Jeffrey E. Steif (1993). "Fixation Results for Threshold Voter Systems". The Annals of Probability 21 (1): 232–247. doi:10.1214/aop/1176989403. 
• Holley, Richard A.; Thomas M. Liggett (1975). "Ergodic Theorems for Weakly Interacting Infinite Systems and The Voter Model". The Annals of Probability 3 (4): 643–663. doi:10.1214/aop/1176996306. 
• Steif, Jeffrey E. (1994). "The Threshold Voter Automaton at a Critical Point". The Annals of Probability 22 (3): 1121–1139. doi:10.1214/aop/1176988597. 
• Liggett, Thomas M. (1985). Interacting Particle Systems. New York: Springer Verlag. ISBN 0387960694. 
• Thomas M. Liggett, "Stochastic Interacting Systems: Contact, Voter and Exclusion Processes", Springer-Verlag, 1999.
• C. Bezuidenhout and G. R. Grimmett, The critical contact process dies out, Ann. Probab. 18 (1990), 1462 -- 1482.