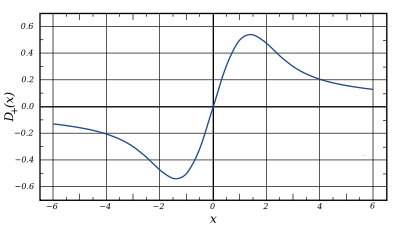
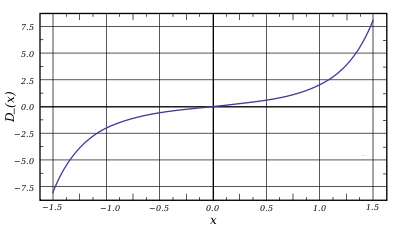
Dawson function

The Dawson function, F(x) = D ₊ (x), around the origin

The Dawson function, D ₋ (x), around the origin

In mathematics, the Dawson function (named for John M. Dawson) is

$D_+(x) = F(x) = e^{-x^2} \int_0^x e^{t^2}\,dt = \frac12 \int_0^\infty e^{-t^2/4}\,\sin{(xt)}\,dt$

The notation D(x) is also in use. The Dawson function is also called the Dawson integral. A variation of this function is given by

$D_-(x) = e^{x^2} \int_0^x e^{-t^2}\,dt.\,\!$

The Dawson function is closely related to the error function erf, as

$F(x) = {\sqrt{\pi} \over 2} e^{-x^2} \mathrm{erfi} (x) = - {i \sqrt{\pi} \over 2} e^{-x^2} \mathrm{erf} (ix)$

where erfi is the imaginary error function, erfi(x) = −i erf(ix).

For |x| near zero, F(x) ≈ x, and for |x| large, F(x) ≈ 1/(2x). More specifically, near the origin it has the series expansion

$F(x) = \sum_{k=0}^{\infty} \frac{(-1)^k \, 2^k}{(2k+1)!!} \, x^{2k+1} = x - \frac{2}{3} x^3 + \frac{4}{15} x^5 - \cdots$

F(x) satisfies the differential equation

$\frac{dF}{dx} + 2xF=1\,\!$

with the initial condition F(0) = 0.

References

• Press, WH; Teukolsky, SA; Vetterling, WT; Flannery, BP (2007), "Section 6.9. Dawson's Integral", Numerical Recipes: The Art of Scientific Computing (3rd ed.), New York: Cambridge University Press, ISBN 978-0-521-88068-8, http://apps.nrbook.com/empanel/index.html#pg=302 
• Temme, N. M. (2010), "Error Functions, Dawson’s and Fresnel Integrals", in Olver, Frank W. J.; Lozier, Daniel M.; Boisvert, Ronald F. et al., NIST Handbook of Mathematical Functions, Cambridge University Press, ISBN 978-0521192255, MR2723248, http://dlmf.nist.gov/7 

External links

• Cephes – C and C++ language special functions math library
• Dawson's Integral (at Mathworld)
• Error functions

This applied mathematics-related article is a stub. You can help Wikipedia by expanding it.v · Categories: Applied mathematics stubs Special functions Gaussian function Wikimedia Foundation. 2010. Игры ⚽ Нужен реферат? Geometrized unit system Debye function Look at other dictionaries: Dawson — may refer to: People Dawson (surname) Roger Dawson, author of Secrets of Power Negotiating Fictional characters Dawson, in Thomas the Tank Engine and Friends Dawson Leery, on the TV show Dawson s Creek Frank Dawson, character on the 1959 1960… …   Wikipedia Dawson-Gärtner theorem — In mathematics, the Dawson Gärtner theorem is a result in large deviations theory. Heuristically speaking, the Dawson Gärtner theorem allows one to transport a large deviation principle on a smaller topological space to a larger one.tatement of… …   Wikipedia Dawson–Gärtner theorem — In mathematics, the Dawson–Gärtner theorem is a result in large deviations theory. Heuristically speaking, the Dawson–Gärtner theorem allows one to transport a large deviation principle on a “smaller” topological space to a “larger” one.… …   Wikipedia Dawson — /daw seuhn/, n. 1. Sir John William, 1820 99, Canadian geologist and educator. 2. William Levi, born 1899, U.S. composer and conductor. 3. a town in NW Canada, at the confluence of the Yukon and Klondike rivers: former capital of the Yukon… …   Universalium Error function — Plot of the error function In mathematics, the error function (also called the Gauss error function) is a special function (non elementary) of sigmoid shape which occurs in probability, statistics and partial differential equations. It is defined …   Wikipedia Fonction de Dawson — La fonction de Dawson, F(x), près de l origine …   Wikipédia en Français Joe Dawson — Highlander character name = Joseph Dawson age = c.55 born = 1950 teacher = Ian Bancroft teacher of = portrayed = Jim Byrnes first = The Watchers movies = , seasons = , , , 5, , |Joe Dawson is a character from the fictional universe of the… …   Wikipedia Rate function — In mathematics mdash; specifically, in large deviations theory mdash; a rate function is a function used to quantify the probabilities of rare events. It is required to have several nice properties which assist in the formulation of the large… …   Wikipedia Origin and function of meiosis — Eukaryotes are organisms with a true nucleus in which the DNA genome is enclosed in a double membrane (e.g. fungi, protozoans, vertebrates, higher plants), in contrast to prokaryotes (bacteria and blue green algae) that lack a nuclear membrane.… …   Wikipedia List of mathematics articles (D) — NOTOC D D distribution D module D D Agostino s K squared test D Alembert Euler condition D Alembert operator D Alembert s formula D Alembert s paradox D Alembert s principle Dagger category Dagger compact category Dagger symmetric monoidal… …   Wikipedia 18+ © Academic, 2000-2024 Contact us: Technical Support, Advertising Dictionaries export, created on PHP, Joomla, Drupal, WordPress, MODx. Mark and share Search through all dictionaries Translate… Search Internet Share the article and excerpts Direct link … Do a right-click on the link above and select “Copy Link”