Dedekind eta function

For the Dirichlet series see Dirichlet eta function.

Dedekind η-function in the complex plane

The Dedekind eta function, named after Richard Dedekind, is a function defined on the upper half-plane of complex numbers, where the imaginary part is positive. For any such complex number $\tau\,$, we define $q = e^{2\pi {\rm{i}} \tau}\,$, and define the eta function by

$\eta(\tau) = q^{\frac{1}{24}} \prod_{n=1}^{\infty} (1-q^{n}).$

(The notation $q \equiv e^{2{\rm{i}} \pi\tau}\,$ is now standard in number theory, though many older books use q for the nome $q \equiv e^{\pi{\rm{i}} \tau}\,$). The presence of 24 can be understood by connection with other occurrences, as in the modular discriminant and the Leech lattice.

The eta function is holomorphic on the upper half-plane but cannot be continued analytically beyond it.

Modulus of Euler phi on the unit disc, colored so that black=0, red=4

The real part of the modular discriminant as a function of q.

The eta function satisfies the functional equations^[1]

$\eta(\tau+1) =e^{\frac{\pi {\rm{i}}}{12}}\eta(\tau),\,$

$\eta(-\tau^{-1}) = \sqrt{-{\rm{i}}\tau} \eta(\tau).\,$

More generally, suppose $a, b, c, d \,$ are integers with $ad-bc=1 \,$, so that

$\tau\mapsto\frac{a\tau+b}{c\tau+d}$

is a transformation belonging to the modular group. We may assume that either $c>0\,$, or $c=0 \,$ and $d=1 \,$. Then

$\eta \left( \frac{a\tau+b}{c\tau+d} \right) = \epsilon (a,b,c,d) (c\tau+d)^{\frac{1}{2}} \eta(\tau),$

where

$\epsilon (a,b,c,d)=e^{\frac{b{\rm{i}} \pi}{12}}\quad(c=0,d=1);$

$\epsilon (a,b,c,d)=e^{{\rm{i}}\pi [\frac{a+d}{12c} - s(d,c) -\frac{1}{4}]}\quad(c>0).$

Here $s(h,k)\,$ is the Dedekind sum

$s(h,k)=\sum_{n=1}^{k-1} \frac{n}{k} \left( \frac{hn}{k} - \left\lfloor \frac{hn}{k} \right\rfloor -\frac{1}{2} \right).$

Because of these functional equations the eta function is a modular form of weight 1/2 and level 1 for a certain character of order 24 of the metaplectic double cover of the modular group, and can be used to define other modular forms. In particular the modular discriminant of Weierstrass can be defined as

$\Delta(\tau) = (2 \pi)^{12} \eta(\tau)^{24}\,$

and is a modular form of weight 12. (Some authors omit the factor of (2π)¹², so that the series expansion has integral coefficients).

The Jacobi triple product implies that the eta is (up to a factor) a Jacobi theta function for special values of the arguments:

$\eta(z) = \sum_{n=1}^\infty \chi(n) \exp(\tfrac{1}{12} \pi i n^2 z),$

where χ(n) is the Dirichlet character modulo 12 with $\chi(\pm1) = 1$, $\chi(\pm 5)=-1$.

The Euler function

$\phi(q) = \prod_{n=1}^{\infty} \left(1-q^n\right),$

related to $\eta \,$ by $\phi(q)= q^{-1/24} \eta(\tau)\,$, has a power series by the Euler identity:

$\phi(q)=\sum_{n=-\infty}^\infty (-1)^n q^{(3n^2-n)/2}.$

Because the eta function is easy to compute numerically from either power series, it is often helpful in computation to express other functions in terms of it when possible, and products and quotients of eta functions, called eta quotients, can be used to express a great variety of modular forms.

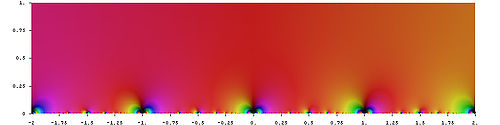
The picture on this page shows the modulus of the Euler function: the additional factor of q^{1 / 24} between this and eta makes almost no visual difference whatsoever (it only introduces a tiny pinprick at the origin). Thus, this picture can be taken as a picture of eta as a function of q.

See also

• q-series
• Weierstrass's elliptic functions
• partition function (number theory)
• Kronecker limit formula
• superstring theory

References

1. ^ Siegel, C.L. (1954). "A Simple Proof of $\eta(-1/\tau) = \eta(\tau)\sqrt{\tau/{\rm{i}}}\,$". Mathematika 1: 4. doi:10.1112/S0025579300000462. 

• Tom M. Apostol, Modular functions and Dirichlet Series in Number Theory (2 ed), Graduate Texts in Mathematics 41 (1990), Springer-Verlag, ISBN 3-540-97127-0 See chapter 3.
• Neil Koblitz, Introduction to Elliptic Curves and Modular Forms (2 ed), Graduate Texts in Mathematics 97 (1993), Springer-Verlag, ISBN 3-540-97966-2