Mordell curve

Mordell curve
y2 = x3 + 1, with solutions at (-1, 0), (0, 1) and (0, -1)

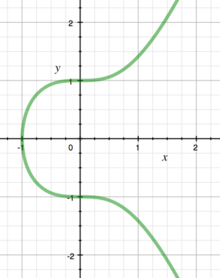
In algebra, a Mordell curve is an elliptic curve of the form y2 = x3 + n, where n is an integer and where n ≠ 0[1]. In Mordell curves, if (x, y) is a solution, it therefore follows that (x, -y) is as well.

These curves were closely studied by Louis Mordell, from the point of view of determining their integer points. He showed that for n fixed there are only finitely many solutions (x, y) in integers.

There are certain values for n in a Mordell curve in which the equation gives no integer solutions, these values are 6, 7, 11, 13, 14, 20, 21, 23, 29, 32, 34, 39, 42...[1]

In other words, the differences of perfect squares and perfect cubes tend to ∞. The question of how fast was dealt with in principle by Baker's method. Hypothetically this issue is dealt with by Marshall Hall's conjecture.

References

  1. ^ a b ""Mathworld Wolfram". October 17. http://mathworld.wolfram.com/MordellCurve.html. 

Bibliography


Wikimedia Foundation. 2010.

Игры ⚽ Нужно решить контрольную?

Look at other dictionaries:

  • Mordell–Weil theorem — In mathematics, the Mordell–Weil theorem states that for an abelian variety A over a number field K, the group A(K) of K rational points of A is a finitely generated abelian group, called the Mordell Weil group. The case with A an elliptic curve… …   Wikipedia

  • Louis J. Mordell — Louis Mordell Louis Mordell in Nizza, 1970. Born 28 January 1888( …   Wikipedia

  • Louis Mordell — Louis Joel Mordell (28 January 1888 12 March 1972) was a British mathematician, known for pioneering research in number theory. He was born in Philadelphia, USA, in a Jewish family of Lithuanian extraction. He came in 1906 to Cambridge to take… …   Wikipedia

  • Elliptic curve — In mathematics, an elliptic curve is a smooth, projective algebraic curve of genus one, on which there is a specified point O . An elliptic curve is in fact an abelian variety mdash; that is, it has a multiplication defined algebraically with… …   Wikipedia

  • Plane curve — In mathematics, a plane curve is a curve in a Euclidean plane (cf. space curve). The most frequently studied cases are smooth plane curves (including piecewise smooth plane curves), and algebraic plane curves. A smooth plane curve is a curve in a …   Wikipedia

  • List of curve topics — This is a list of curve topics in mathematics. See also curve, list of curves, and list of differential geometry topics.*acnode *algebraic curve *arc *asymptote *asymptotic curve *Barbier s theorem *barycentric… …   Wikipedia

  • Fermat curve — In mathematics, the Fermat curve is the algebraic curve in the complex projective plane defined in homogeneous coordinates ( X : Y : Z ) by the Fermat equation: X n + Y n = Z n .Therefore in terms of the affine plane its equation is: x n + y n =… …   Wikipedia

  • Courbe elliptique — Une sélection de courbes cubiques réelles définies par l équation y2 = x3 + ax + b.. La région montrée est [ − 3,3]2. La courbe pour a = b = 0 n est pas elliptique. En mathématiques, une courbe elliptique est un …   Wikipédia en Français

  • Diophantine geometry — In mathematics, diophantine geometry is one approach to the theory of Diophantine equations, formulating questions about such equations in terms of algebraic geometry over a ground field K that is not algebraically closed, such as the field of… …   Wikipedia

  • List of mathematics articles (M) — NOTOC M M estimator M group M matrix M separation M set M. C. Escher s legacy M. Riesz extension theorem M/M/1 model Maass wave form Mac Lane s planarity criterion Macaulay brackets Macbeath surface MacCormack method Macdonald polynomial Machin… …   Wikipedia

Share the article and excerpts

Direct link
… Do a right-click on the link above
and select “Copy Link”